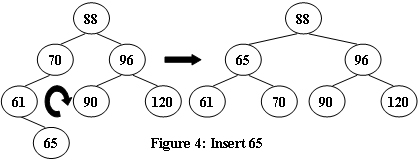
An AVL tree is a self-balancing binary search tree. In an AVL tree, the heights of the two child subtrees of any node differ by at most one; if at any time they differ by more than one, rebalancing is done to restore this property. Figures 1-4 illustrate the rotation rules.
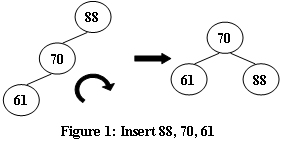
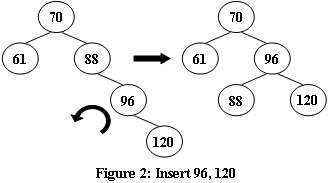
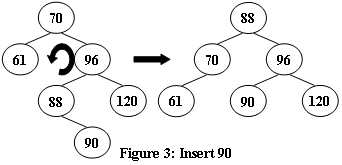
Now given a sequence of insertions, you are supposed to tell the root of the resulting AVL tree.
Input Specification:
Each input file contains one test case. For each case, the first line contains a positive integer N (≤) which is the total number of keys to be inserted. Then N distinct integer keys are given in the next line. All the numbers in a line are separated by a space.
Output Specification:
For each test case, print the root of the resulting AVL tree in one line.
Sample Input 1:
5
88 70 61 96 120
Sample Output 1:
70
Sample Input 2:
7
88 70 61 96 120 90 65
Sample Output 2:
88
题目分析:写一个AVL树 背课文是好的 但也要理解清除AVL树的原理 之后还要学习各种平衡树 如红黑树什么的
1 #define _CRT_SECURE_NO_WARNINGS 2 #include <climits> 3 #include<iostream> 4 #include<vector> 5 #include<queue> 6 #include<map> 7 #include<set> 8 #include<stack> 9 #include<algorithm> 10 #include<string> 11 #include<cmath> 12 using namespace std; 13 typedef struct TNode* Tree; 14 struct TNode { 15 int Data; 16 Tree TL; 17 Tree TR; 18 int Height; //要初始化为-1; 19 }; 20 int GetHeight(Tree T) { 21 if (T) 22 return T->Height; 23 else 24 return -1; 25 } 26 Tree singleLeftRotate(Tree T) { 27 Tree TL = T->TL; 28 T->TL = TL->TR; 29 TL->TR = T; 30 T->Height = max(GetHeight(T->TL), GetHeight(T->TR)) + 1; 31 TL->Height = max(GetHeight(TL->TL), GetHeight(TL->TR)) + 1; 32 return TL; 33 } 34 Tree singleRightRotate(Tree T) { 35 Tree TR = T->TR; 36 T->TR = TR->TL; 37 TR->TL = T; 38 T->Height = max(GetHeight(T->TL), GetHeight(T->TR)) + 1; 39 TR->Height = max(GetHeight(TR->TL), GetHeight(TR->TR)) + 1; 40 return TR; 41 } 42 Tree doubleLeftRightRotate(Tree T) { 43 T->TL = singleRightRotate(T->TL); 44 return singleLeftRotate(T); 45 } 46 Tree doubleRightLeftRotate(Tree T) { 47 T->TR = singleLeftRotate(T->TR); 48 return singleRightRotate(T); 49 } 50 Tree Insert(Tree T,int data) { 51 if (!T) 52 { 53 T = new TNode(); 54 T->Data = data; 55 T->Height = 0; 56 T->TL = T->TR = NULL; 57 } 58 else if (data > T->Data) { 59 T->TR = Insert(T->TR, data); 60 T->Height = max(GetHeight(T->TL), GetHeight(T->TR)) + 1; 61 if (GetHeight(T->TR) - GetHeight(T->TL) == 2) { 62 if (data > T->TR->Data) 63 T=singleRightRotate(T); 64 else 65 T=doubleRightLeftRotate(T); 66 } 67 } 68 else { 69 T->TL = Insert(T->TL, data); 70 T->Height = max(GetHeight(T->TL), GetHeight(T->TR)) + 1; 71 if (GetHeight(T->TL) - GetHeight(T->TR) == 2) { 72 if (data < T->TL->Data) 73 T=singleLeftRotate(T); 74 else 75 T=doubleLeftRightRotate(T); 76 } 77 } 78 return T; 79 } 80 81 82 int main() 83 { 84 int N; 85 Tree T = NULL; 86 int data; 87 cin >> N; 88 for (int i = 0; i < N; i++) 89 { 90 cin >> data; 91 T = Insert(T, data); 92 } 93 cout << T->Data; 94 }