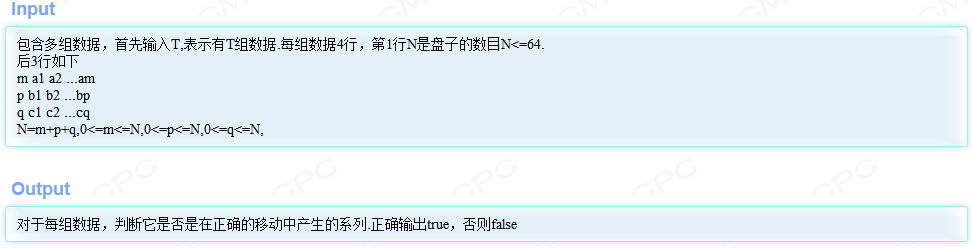
Sample Input
6 3 1 3 1 2 1 1 3 1 3 1 1 1 2 6 3 6 5 4 1 1 2 3 2 6 3 6 5 4 2 3 2 1 1 3 1 3 1 2 1 1 20 2 20 17 2 19 18 16 16 15 14 13 12 11 10 9 8 7 6 5 4 3 2 1
Sample Output
true false false false true true
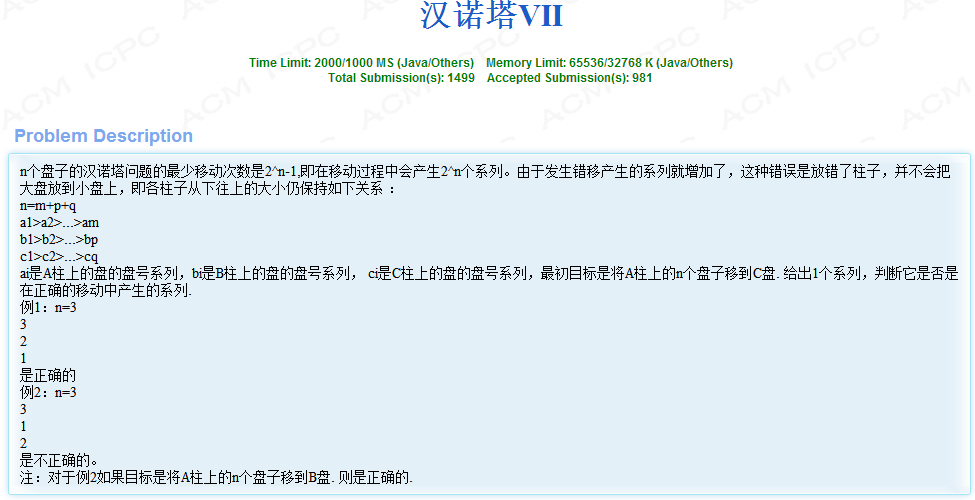
(1)首先判断是不是已经完全放好了或者还没有开始移动,这样就不用考虑是否最优化了,即所有的盘子在C柱上或者所有的盘子在A柱上,这样是合法的,直接输出true。
(2)【我们考虑盘号最大的盘子第n号盘子,移动方向为A–>C,它只可能在A柱或者C柱上,如果在B柱上我们可以直接返回false–①】;
如果盘号最大的盘子在A柱上,说明其它盘子正在进行A–>B的操作,所以考虑移动的那个n-1个盘子,然后同样按①判断,只不过,最大盘号变成了n-1,而移动方向变成了A–>B;
如果盘号最大的盘子在C柱上,说明其它盘子正在进行B–>C的操作,所以考虑移动的那个n-1个盘子,然后同样按①判断,只不过,最大盘号变成了n-1,而移动方向变成了B–>C;
利用递归按这种方式不断地进行①操作。
#include<stdio.h>
int map[4][100];
int f(int a,int b) {
if(a==1)
return b==2?3:2;
if(a==2)
return b==1?3:1;
if(a==3)
return b==1?2:1;
}
bool judge(int n,int t1,int t2) {
if(n==0)
return true;
for(int i=0; i<map[0][f(t1,t2)]; i++)
if(map[f(t1,t2)][i]==n)
return false;
for(int i=0; i<map[0][t1]; i++)
if(map[t1][i]==n)
return judge(n-1,f(t1,t2),t1);
for(int i=0; i<map[0][t2]; i++)
if(map[t2][i]==n)
return judge(n-1,f(t1,t2),t2);
}
int main() {
int T;
scanf("%d",&T);
while(T--) {
int n;
scanf("%d",&n);
scanf("%d",&map[0][1]);
for(int i=0; i<map[0][1]; i++)
scanf("%d",&map[1][i]);
scanf("%d",&map[0][2]);
for(int i=0; i<map[0][2]; i++)
scanf("%d",&map[2][i]);
scanf("%d",&map[0][3]);
for(int i=0; i<map[0][3]; i++)
scanf("%d",&map[3][i]);
printf("%s
",judge(n,1,3)?"true":"false");
}
return 0;
}题目地址:【杭电】[1997]汉诺塔VII