#include <iostream>
#include <algorithm>
#include <cmath>
using namespace std;
struct point {
double x, y;
};
struct v {
point start, end;
};
double dotProduct(v v1, v v2) {
return (v1.end.x - v1.start.x)*(v2.end.x - v2.start.x) + (v1.end.y - v1.start.y)*(v2.end.y - v2.start.y);
}
double crossProduct(v v1, v v2) {
return (v1.end.x - v1.start.x)*(v2.end.y - v2.start.y) - (v2.end.x - v2.start.y)*(v1.end.y - v1.start.y);
}
bool onSegment(point p1, point p2, point p0) {
if (fabs((p1.x - p2.x)*(p1.y - p0.y) - (p1.x - p0.x)*(p1.y - p2.y))<1e-7 &&
min(p1.x, p2.y) <= p0.x&&max(p1.x, p1.x) >= p0.x&&
min(p1.y, p2.y) <= p0.y&&max(p1.y, p2.y) >= p0.y
)
return true;
return false;
}
point polygon[100];
//n表示多边形的顶点个数
bool inPolygon(point *polygon,point p,int n) {
int cnt = 0;
point p1, p2;
for (int i = 0; i < n; i++) {
p1 = polygon[i];
p2 = polygon[(i + 1) % n];
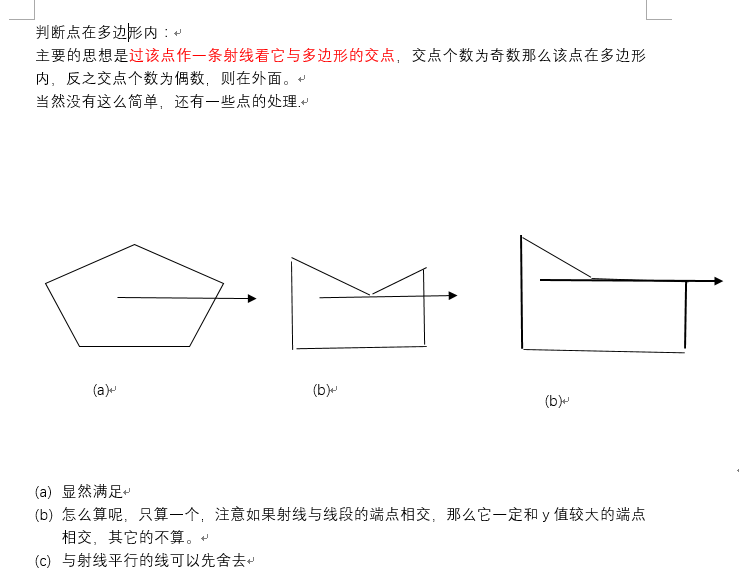
//判断点是否在多边形上
if (onSegment(p1, p2, p)) return true;
//这是排除(b)种情况
if(p.y>min(p1.y,p2.y))
if (p.y <= max(p1.y, p2.y))
//排除p1p2和射线平行的情况
if (p1.y != p2.y) {
//这是求出线段和过该点的射线的交点
int x0 = (p.y - p1.y) / (p2.y - p1.y)*(p2.x - p1.x) + p1.x;
if (p.x <= x0) cnt++;
}
}
if (cnt % 2 == 0) return false;
else return true;
}
int main() {
int n;
point p;
while (cin >> n) {
for (int i = 0; i < n; i++)
cin >> polygon[i].x >> polygon[i].y;
cin >> p.x >> p.y;
cout << inPolygon(polygon, p, n) << endl;
}
}
