根据字典和指定长度,按排列组合生成字符串(七个方案)
命题
根据指定的字符集合(字典),按排列组合的规则(允许重复),生成指定长度的所有字符串。如下代码:
class Program { static void Main(string[] args) { string dic = "abcdef"; int len = 3; int top_last_N = 5; IGenerator g = new Generator1(); var result = g.Generate(dic, len) ?? new List<string>(); Console.WriteLine("{0} strings generated:", result.Count); PrintTheResult(top_last_N, result); Console.ReadKey(); } private static void PrintTheResult(int top_last_N, List<string> result) { if (result.Count > top_last_N * 2) { for (int i = 0;i < top_last_N;i++) { Console.WriteLine(result[i]); } Console.WriteLine("......"); for (int i = result.Count - top_last_N;i < result.Count;i++) { Console.WriteLine(result[i]); } } else { foreach (var s in result) { Console.WriteLine(s); } } } } interface IGenerator { List<string> Generate(string dic, int length); } class Generator1 : IGenerator { public List<string> Generate(string dic, int length) { // we should implement this method throw new NotImplementedException(); } }
举个例子,比如:字典为[a,b,c],指定长度为 2,则生成的所有字符串应当为:
"aa","ab","ac","ba",……,"ca","cb","cc",总计9个字符串。
那么程序里我们应当怎么来生成这些字符串呢?如下循序渐进地列出解决方案。
- 投石问路:假设长度固定
- 方案一:我们来多循环几次(循环拼接字符串)
- 方案二:不用字符串,使用不安全代码(循环拼接字符串Unsafe版)
- 方案三:换个角度看问题(字符串模拟数字依次循环进行进制转换)
- 方案四:小学的加法运算正闪闪发光(字符串模拟数字依次自增+1)
- 方案五:再次改写,不用字符串,使用不安全代码(字符串模拟数字依次自增+1的Unsafe版)
- 方案六:闲来没事(1):Fixed-point combinator
- 方案七:闲来没事(2):Fixed-point combinator + Unsafe 双剑合璧
投石问路:假设长度固定
命题中有三个关键要素:字典、长度、排列组合(允许重复)。
首先我们假定长度固定,比如长度为“3”,直接使用给定的字典进行排列组合就行了。
既然是排列组合,我首先想到的是循环,于是有:
class Generator1 : IGenerator { public List<string> Generate(string dic, int length) { // since we deal with the "length" as a constant parameter, so we leave it alone. var result = new List<string>(); foreach (var x in dic) { foreach (var y in dic) { foreach (var z in dic) { result.Add(string.Format("{0}{1}{2}", x, y, z)); } } } return result; } }
长度为3,那么我们用3个循环生成每一位的字符,然后拼凑起来,这简直太简单了,运行效果如下:
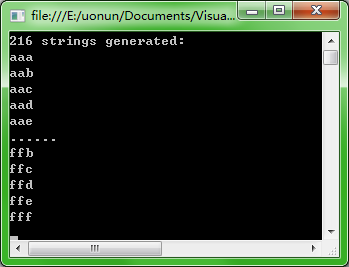
OK,好了,如果考虑长度为呢?长度直接关系到需要循环的次数,完了,我们的循环都是写死在代码里的,我们怎么知道传进来的长度参数值是多少,需要循环多少次啊?
方案一:我们来多循环几次
说实在的,我觉得循环就是一个玩命却又任劳任怨的苦工。无论多么深、多么广、多么耗时的循环,他都能反反复复、机机械械地去执行,要么执行完成,要么累死——资源耗尽。
对于上文中的 Generate 方法,我们需要根据参数来确定循环的次数,那么我们定义一个方法专门来做循环,然后根据参数的值来运行若干次。
仔细观察我们的第一次实现,除第一次循环之外,每一次的循环都是在之前循环得到的字符串基础上,追加一个字符,从而实现“拼凑”到足够长度的目的。那么第一次呢?其实第一次的循环就只是从依次从字典里取出每一个字符,放到结果集里,作为后续“拼凑”动作的种子。因此,首先,我们需要一个种子,循环在这个基础上进行,有了种子之后,就从种子的每一个元素上,发芽(分化)出第二次的“拼凑”...直到N次——看起来,这已经是一个树的生成了,只不过这个树比较特殊,每一个节点的分支数都一样。
好了,见代码:
class Generator2LoopSegments : IGenerator { public List<string> Generate(string dic, int length) { List<string> result = null; for (int i = 0;i <= length;i++) { result = Loop(dic, result); } return result; } private List<string> Loop(string dic, List<string> seed) { var result = new List<string>(); if (seed == null || seed.Count == 0) { // for the first time, we just choose one char only to fill full into the List. // result.AddRange(from c in dic select c.ToString()); result.Add(string.Empty); } else { for (int i = 0;i < seed.Count;i++) { foreach (var c in dic) { result.Add(string.Format("{0}{1}", seed[i], c)); } } } return result; } public override string ToString() { return "Loop segments"; } }
好了,完美无瑕,实现了命题中要求的排列组合!不过,等等,咦,怎么当我 length 设为 5 的时候,竟然抛出 OutOfMemoryException 的异常了?内存占用很高啊!嗯,是不是因为编译成了 32 位程序的原因?改成 64 位试试?哈,行了,不过内存竟然占用了 3G!
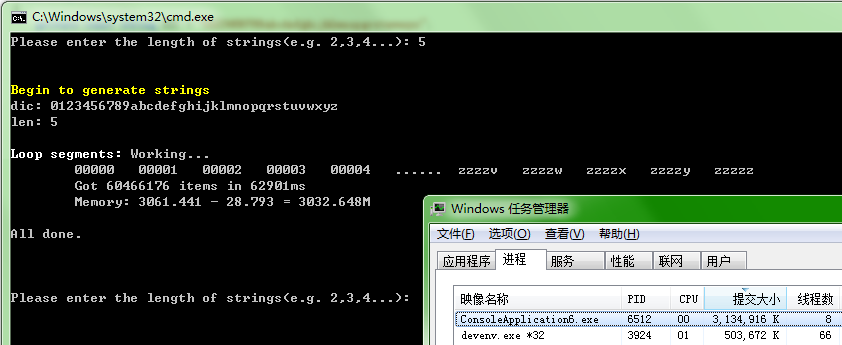
Tips:
编译为32位程序,在64位Win7系统中运行,当内存占用接近2G时,程序即抛出 OutOfMemoryException 的异常,直接表现为无法创建字符串或无法向集合添加新项。
运行是能运行了,不过时间上貌似不尽人意,运行太久了,是不是还能有提高呢?怎么办呢?这里使用了大量字符串,对字符串的操作也非常多,要不咱不使用字符串,直接使用 char* 试试?
方案二:不用字符串,使用不安全代码
思路和上面的解决方案一致,只是对上面字符串相关的操作进行了修改,使用不安全代码 unsafe 。
class Generator2LoopSegmentsUnsafe : IGenerator { public unsafe List<string> Generate(string dic, int length) { char*[] result = null; for (int i = 0;i < length;i++) { result = Loop(dic, result); } var list = new List<string>(); if (result != null) { foreach (var p in result) { var aa = new String(p); list.Add(aa); } } return list; } private unsafe char*[] Loop(string dic, char*[] seed) { char*[] result = null; if (seed == null || seed.Length == 0) { result = new char*[dic.Length]; // for the first time, we just choose one char only to fill full into the List. for (int i = 0;i < dic.Length;i++) { IntPtr p = Marshal.AllocHGlobal(sizeof(char)); result[i] = (char*)p.ToPointer(); *result[i] = dic[i]; // string will/should be end with the char '�'. *(result[i] + 1) = '�'; } } else { result = new char*[seed.Length * dic.Length]; int n = 0; for (int i = 0;i < seed.Length;i++) { foreach (var c in dic) { IntPtr p = Marshal.AllocHGlobal(sizeof(char)); result[n] = (char*)p.ToPointer(); *(result[n]) = *seed[i]; int j = 0; // string will/should be end with the char '�'. while (*(seed[i] + ++j) != '�') { *(result[n] + j) = *(seed[i] + j); } *(result[n] + j) = c; // string will/should be end with the char '�'. *(result[n] + j + 1) = '�'; n++; } } } return result; } public override string ToString() { return "Loop segments(unsafe)"; } }
运行结果如下:
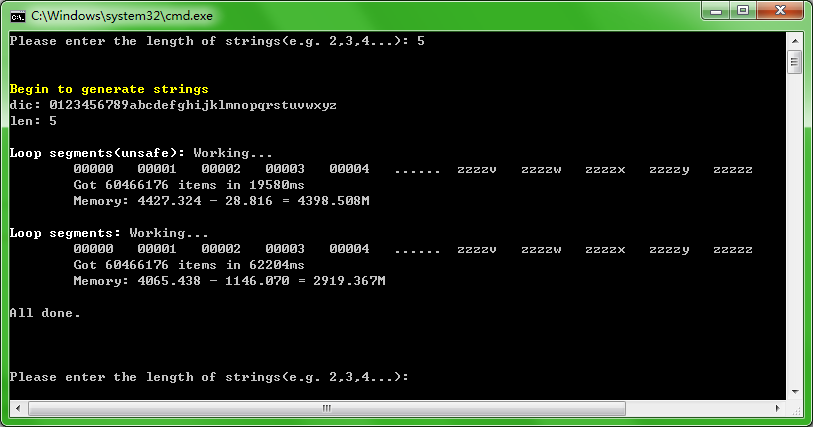
OK,从结果来看和上一个版本一致,不过时间和内存占用都不一样了!时间上少了很多,内存占用貌似不减反增,我猜想应当是因为 framework 中对字符串的处理比我手写得高端大气上档次一些吧……
到这里,看起来已经没太多的提升空间了,毕竟我们连这么高端的不安全代码都用了。
果然是这样吗?唉,别忘记了基础的东西,这玩意的时间复杂度有点高啊?(O(N^3)?猜的。时间复杂度这玩意,我一直没怎么看过...如果不对,请不吝赐教....)
嗯,能不能有个更简单的算法,让它的时间复杂度更低呢?
方案三:换个角度看问题(字符串模拟数字依次循环进行进制转换)
其实,如果细心你可能发现,这个排列组合,如果我们把字典换一下……
比如 [0,1],长度 3,这将得到:000,001,010,011,100,101,110,111
比如 [0-9],长度 2,这将得到:00,01,02,03,04,…,97,98,99
我去~这是啥?这不就是连续的整数么?
嗯,我们再看看,比如字典为 [0-9A-F],长度 2,这将得到:00,01,02,...,09,0A,0B,...,0F,10,11,...,1A,1B,...,1F,20,21,...,FD,FE,FF
这,依然是连续整数,只不过是十六进制而已!
说到这里,其实你已经看出来了,上面依次就是二进制,十进制和十六进制。当他们是这些情况时,我们想排列组合,那岂不是易如反掌?让数字递增加一,直接一个循环就输出搞定了!
嗯,好办法,继续看,当字典为任意字符集的时候,可以吗?答案当然是可以的。[0-9A-F] 是十六进制,那 [0-9A-Z] 则自然是36进制!
既然如此,那我们的“排列组合”算法,就成了进制转换的算法了,囧。
参考:http://baike.baidu.com/link?url=q_M-tOHBN0XtyvdHBv-sk8vuV8dJf-Yna-7nRp1xwYQP-m2pj9CHV0x-u0nbChAN
好吧,代码来了:
class Generator3LoopAsNumbers : IGenerator { public List<string> Generate(string dic, int length) { var fromBase = dic.Length; int min = 0, max = 0; if (fromBase > 1) { max = (int)Math.Pow(fromBase, length) - 1; } else { max = min; } //Console.WriteLine("min = {0}, max = {1}", min, max); var result = new List<string>(); for (var current = min;current <= max;current++) { var tmp = ""; for (var j = length - 1;j >= 0;j--) { var last = (int)(current % Math.Pow(fromBase, j + 1) / Math.Pow(fromBase, j)); tmp += dic[last]; } result.Add(tmp); } return result; } public override string ToString() { return "Loop as numbers"; } }
运行如图:
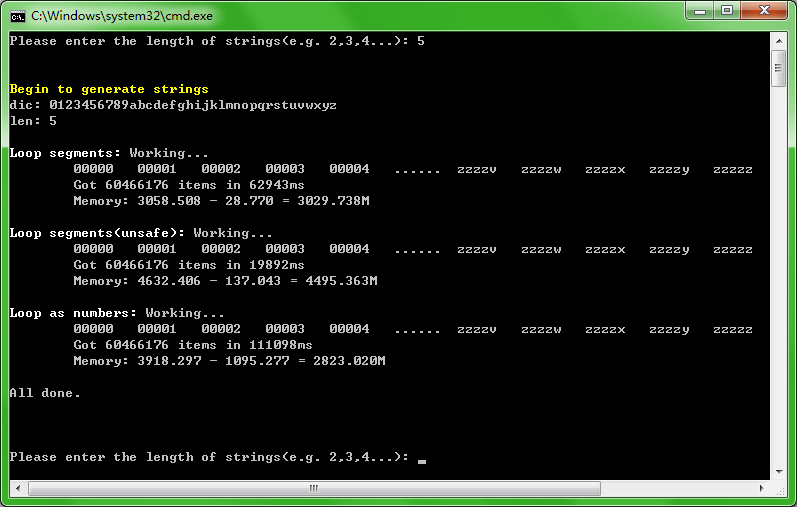
不过,好的想法不一定能运行出好的结果。按照这个解决方案,内存占用降了下来,但耗时却高了不少,将近第一个方案的两倍了,简直惨不忍睹!
正当我为之沮丧时,却突然又发现一丝曙光:既然我已经将这个排列组合看成是数字递增了,而且递增多少我也很清楚地知道,那为何我还要“劳心劳力”地去做进制转换呢?何不直接根据进制的规则,+1,+1,升位+1去做呢?小学加法啊!
说改就改!
方案四:小学的加法运算正闪闪发光(字符串模拟数字依次自增+1)
还记得小学时学的加法运算怎么算么?按小数点对齐,个位加个位,十位加十位,满10进一位。OK,按照这个思路再试一次看看,代码来了:
class Generator4GenerateNumbers : IGenerator { public List<string> Generate(string dic, int length) { var result = new List<string>(); string seed = new string(dic[0], length); result.Add(seed); while (GetNext(ref seed, seed.Length - 1, dic)) { result.Add(seed); } return result; } private bool GetNext(ref string seed, int idx, string dic) { if (idx < 0 || idx >= seed.Length) return false; char c = seed[idx]; if (dic.IndexOf(c) < dic.Length - 1) { c = dic[dic.IndexOf(c) + 1]; seed = seed.Substring(0, idx) + c + seed.Substring(idx + 1, seed.Length - 1 - idx); return true; } else { c = dic[0]; seed = seed.Substring(0, idx) + c + seed.Substring(idx + 1, seed.Length - 1 - idx); return GetNext(ref seed, idx - 1, dic); } } public override string ToString() { return "Generate numbers"; } }
当然,可以从代码中看出来,我们这里的加法运算太简单了,简单到第二个因子是 1,也就是每次运算加法只是数字递增1而已。其中dic[0]表示了每位可能的最小的数,相应地,dic[dic.Length-1]表示了没位可能的最大的数。
我们来运行试试:

成是成了,不过……这个结果依然有点惨!效率不高,内存占用仍然居高不下,看来这闪闪的光——要不是我还没发掘出来,那就是我眼花了?嗯……要不再改写成非安全代码试试?
方案五:再次改写,不用字符串,使用不安全代码
照例,算法思路和上面一样,只是改写成非安全代码:
class Generator4GenerateNumbersUnsafe : IGenerator { public List<string> Generate(string dic, int length) { var result = new List<string>(); unsafe { IntPtr p = Marshal.AllocHGlobal(sizeof(char)); var seed = (char*)p.ToPointer(); int i = 0; for (;i < length;i++) { *(seed + i) = dic[0]; } *(seed + i) = '�'; do { result.Add(new string(seed)); } while (GetNext(ref seed, length - 1, dic)); } return result; } private unsafe bool GetNext(ref char* seed, int idx, string dic) { int len = 0; for (;*(seed + len) != '�';len++) { } if (idx < 0 || idx >= len) return false; char c = seed[idx]; if (dic.IndexOf(c) < dic.Length - 1) { c = dic[dic.IndexOf(c) + 1]; *(seed + idx) = c; return true; } else { c = dic[0]; *(seed + idx) = c; return GetNext(ref seed, idx - 1, dic); } } public override string ToString() { return "Generate numbers(unsafe)"; } }
哈,看起来效果不错哦,时间和空间的占用都有提升,并且效果比第二个方案还好!
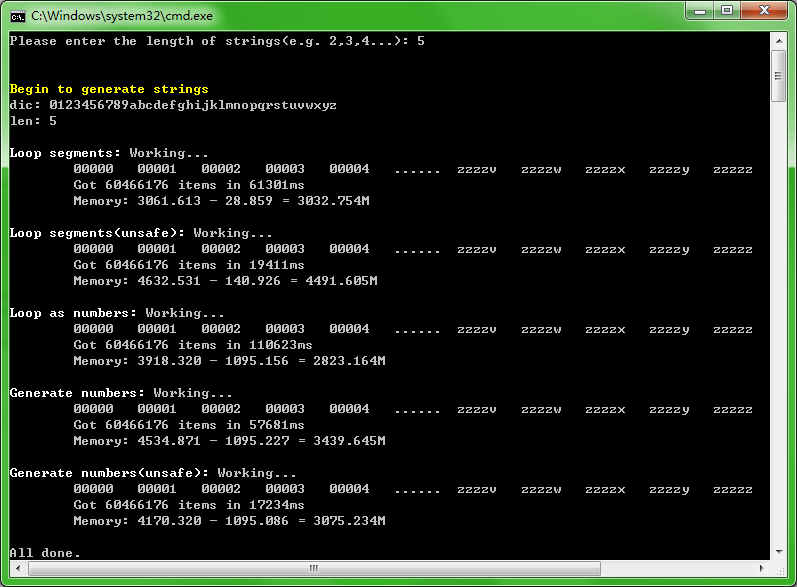
好了,算法的尝试到此为止。
下面来点无聊的东西:不动点组合子。
方案六:闲来没事(1):Fixed-point combinator
关于 Fixed-point combinator 可以参考:
http://en.wikipedia.org/wiki/Fixed-point_combinator
http://zh.wikipedia.org/wiki/%E4%B8%8D%E5%8A%A8%E7%82%B9%E7%BB%84%E5%90%88%E5%AD%90
如下只是使用 Fixed-point combinator 对上一个解决方案的改写,效率更加底下,或许有提升空间……或许……
class Generator4GenerateNumbersFixedPoint : IGenerator { public List<string> Generate(string dic, int length) { var result = new List<string>(); var seed = new string(dic[0], length); while (!string.IsNullOrEmpty(seed)) { result.Add(seed); seed = FixedPointCombinator( x => { if (x != null) { return (s, idx, idc) => { if (idx < 0 || idx >= s.Length) return null; char c = s[idx]; if (dic.IndexOf(c) < dic.Length - 1) { c = dic[dic.IndexOf(c) + 1]; s = s.Substring(0, idx) + c + s.Substring(idx + 1, s.Length - 1 - idx); } else { c = dic[0]; s = s.Substring(0, idx) + c + s.Substring(idx + 1, s.Length - 1 - idx); s = x(s, idx - 1, dic); } return s; }; } return null; })(seed, seed.Length - 1, dic); } return result; } private Func<string, int, string, string> FixedPointCombinator( Func<Func<string, int, string, string>, Func<string, int, string, string>> f1) { return (x, y, z) => f1(FixedPointCombinator(f1))(x, y, z); } public override string ToString() { return "Generate numbers(Fixed-Point Y)"; } }
注意,下面的代码可以编译通过,不过这……是个死循环,在构造 Fixed-Point Y 时,尤其需要注意:
private Func<string, int, string, string> Combinator_Recursive( Func<Func<string, int, string, string>, Func<string, int, string, string>> f1) { return f1(Combinator_Recursive(f1)); }
惯例,贴出运行结果:
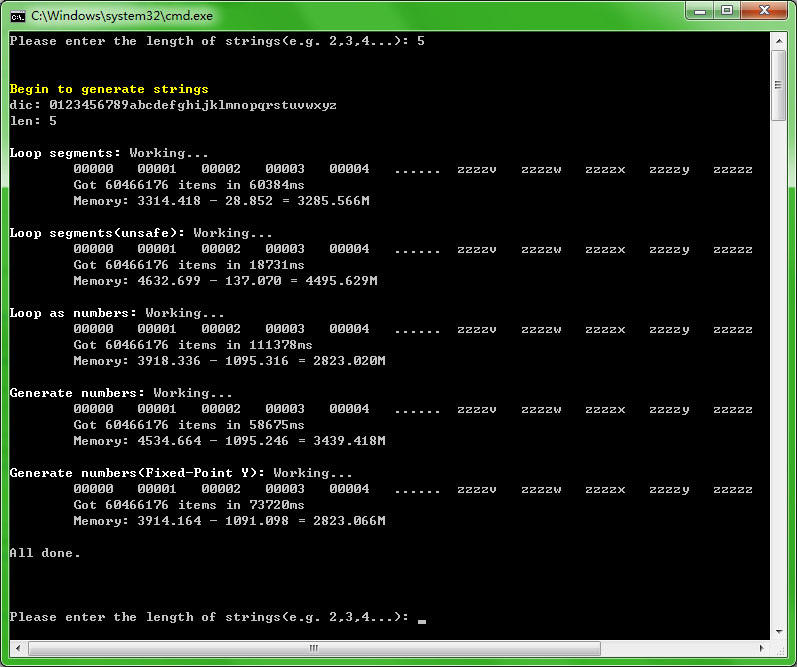
看样子,运行效果不尽人意。
方案七:闲来没事(2):Fixed-point combinator + Unsafe 双剑合璧
当然,Fixed-point combinator 也有 Unsafe 版本:
class Generator4GenerateNumbersFixedPointUnsafe : IGenerator { public List<string> Generate(string dic, int length) { var result = new List<string>(); unsafe { IntPtr p = Marshal.AllocHGlobal(sizeof(char)); var seed = (char*)p.ToPointer(); int i = 0; for (;i < length;i++) { *(seed + i) = dic[0]; } *(seed + i) = '�'; bool tmp = true; while (tmp) { result.Add(new string(seed)); tmp = FixedPointCombinator( x => { if (x != null) { return (s) => { int len = 0; for (;*(s.Seed + len) != '�';len++) { } if (s.Idx < 0 || s.Idx >= len) return false; char c = s.Seed[s.Idx]; if (s.Dic.IndexOf(c) < s.Dic.Length - 1) { c = s.Dic[s.Dic.IndexOf(c) + 1]; *(s.Seed + s.Idx) = c; return true; } else { c = s.Dic[0]; *(s.Seed + s.Idx) = c; s.Idx--; return x(s); } }; } return null; })(new Args() { Seed = seed, Idx = length - 1, Dic = dic }); } } return result; } private Func<Args, bool> FixedPointCombinator( Func<Func<Args, bool>, Func<Args, bool>> f1) { return (x) => f1(FixedPointCombinator(f1))(x); } public override string ToString() { return "Generate numbers(Fixed-Point Y, unsafe)"; } private unsafe struct Args { public char* Seed; public int Idx; public string Dic; } }
下面是运行结果:
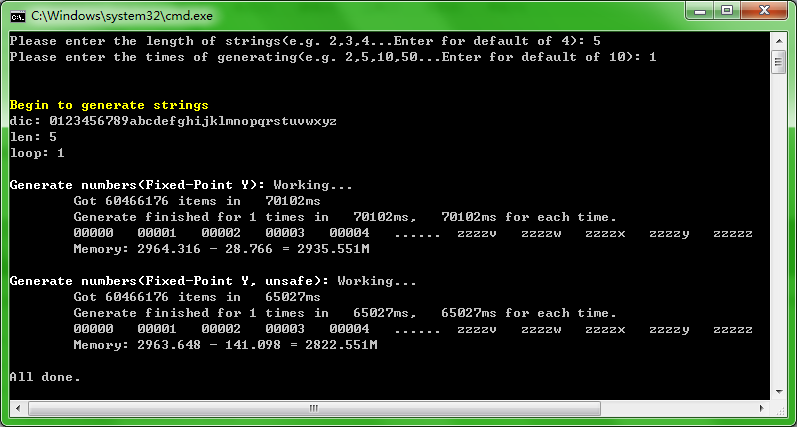
结语
OK,我们来系统地比较一下这些方案吧,由于时间关系,我测试了生成字符串长度为4,并且每个方案在Release下运行50次取平均的结果:

值得注意的是,方案二的内存占用却异常的高,难道是因为Demo代码统计的问题?针对这个,我又重新运行了方案二和方案五,并且对调了他们的执行先后顺序。如下图:
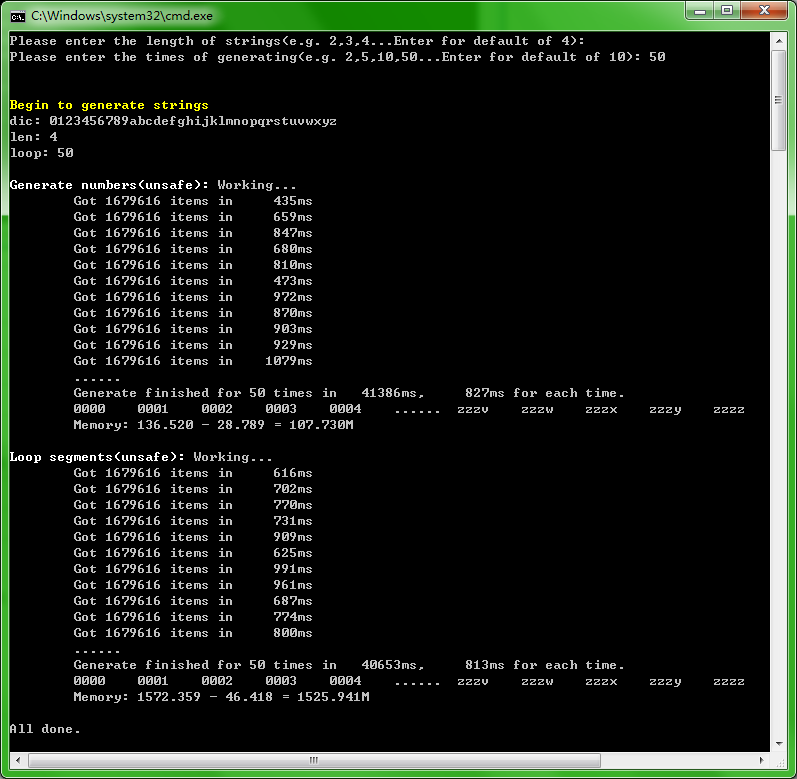
| 方案 | 平均耗时(ms) | 连续运行50次后的内存占用(M) |
| 方案一:循环拼接字符串 | 1930 | 241.852 |
| 方案二:循环拼接字符串Unsafe版 | 813 | 1546.480 |
| 方案三:字符串模拟数字依次循环进行进制转换 | 2738 | 167.105 |
| 方案四:字符串模拟数字依次自增+1 | 1550 | 93.297 |
| 方案五:字符串模拟数字依次自增+1的Unsafe版 | 853 | 74.844 |
| 方案六:不动点组合子 | 2152 | 111.406 |
| 方案七:不动点组合子Unsafe版 | 1907 | 94.691 |
从上表中可以看出:
- 非字符串方案(四、五)比字符串方案(一、二)更优;
- Unsafe版本比对应的原始版本更优,想必是Unsafe版本只处理上下文必要的相关逻辑,不用像Framework里那样考虑得面面俱到。
具体地:
- 时间消耗上,方案二和方案五不相上下,并且远低于其他方案,作为Unsafe升级版,也都差不多是原始版本的1/2;
- 内存消耗上,方案二的内存占用异常高,而同为Unsafe版本的方案五内存占用最低。
详细分析代码可以看出,方案二为了保证生成的字符串集合的顺序,因此在每一次递归时都构造了一个新的数组,并保留了原来数组(seed),而不像方案五那样,直接在每一次循环时修改指针所指变量的值。我猜想,如果将方案二中对数组的相关操作再次进行Unsafe化,比如不为扩容而另外构造新数组,而是直接将seed扩容,那内存的占用应当会降低很多。只是Array.Resize<T>并不支持 char*,得自己写一个了~
最后来看,整个七个方案中,莫过于方案五最优了:
- 它以数字自增的逻辑思路来执行,从而达到了O(1)的时间复杂度(虽然仍然用到了递归);
- 没有使用 framework 中的 string,而是直接有针对性地使用 char*,从而有效避免了不必要的字符串级别操作。
- 没有使用像方案二中的数组,而是直接改写指针所指变量的值,从而有效避免了大量的内存分配,降低了内存的占用。
综上,以下为一些心得小记:
- 对字符串的操作比想象中降性能、耗内存资源。
- 要提高执行效率,使用非安全代码改写针对字符串的算法可以以空间换时间,有效地提供执行效率。(既然是非安全代码,还需全方位测试。不过请放心,它将不会导致引用该程序集的其他程序集启用非安全代码)
- 从递归改到Fixed-Point Y的过程是个很绕脑袋的过程,我确信这是一种很重要的思想(函数式、自生成),但仍然没能明确其更具体的应用场景,何时何地它是最优的解决方案?