POJ 3070(求斐波那契数 矩阵快速幂)

题意就是求第 n 个斐波那契数。

由于时间和内存限制，显然不能直接暴力解或者打表，想到用矩阵快速幂的做法。

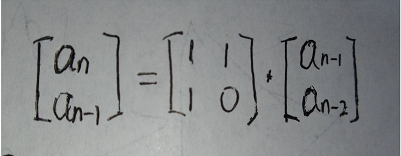
代码如下：

#include <cstdio>
using namespace std;
const int maxn = 100;
const int mod = 10000;
int a;
struct Matrix
{
    int m[maxn][maxn];
}ans,res,w,head;

Matrix mul(Matrix a,Matrix b,int n)
{
    Matrix tmp;
    for(int i = 1; i <= n; i++)
        for(int j = 1; j <= n; j++)
            tmp.m[i][j] = 0;
    for(int i = 1; i <= n; i++)
        for(int j = 1; j <= n; j++)
            for(int k = 1; k <= n; k++)
                tmp.m[i][j] += ((a.m[i][k] % mod)*(b.m[k][j] % mod))%mod;
    return tmp;
}

void quickpow(int N,int n)
{
    for(int i = 1; i <= n; i++)
        for(int j = 1; j <= n; j++)
            if(i == j)  ans.m[i][j] = 1;
            else ans.m[i][j] = 0;
    while(N)
    {
        if(N&1) ans = mul(ans,res,2);
        res = mul(res,res,2);
        N = N >>1;
    }
}

int main()
{
    while(scanf("%d",&a))
    {
        if(a == -1) break;
        else if(a == 0)
        {
            puts("0");
            continue;
        }
        head.m[1][1] = head.m[1][2] = head.m[2][2] = 0;
        head.m[2][1] = 1;
        res.m[1][1] = res.m[1][2] = res.m[2][1] = 1;
        res.m[2][2] = 0;
        quickpow(a,2);
        w = mul(ans,head,2);
        printf("%d
",w.m[1][1]);
    }
    return 0;
}