codeforces 920E Connected Components?

You are given an undirected graph consisting of n vertices and 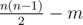 edges. Instead of giving you the edges that exist in the graph, we give you m unordered pairs (x, y) such that there is no edge between x and y, and if some pair of vertices is not listed in the input, then there is an edge between these vertices.

You have to find the number of connected components in the graph and the size of each component. A connected component is a set of vertices X such that for every two vertices from this set there exists at least one path in the graph connecting these vertices, but adding any other vertex to X violates this rule.

Input

The first line contains two integers n and m (1 ≤ n ≤ 200000, ).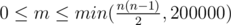

Then m lines follow, each containing a pair of integers x and y (1 ≤ x, y ≤ n, x ≠ y) denoting that there is no edge between x and y. Each pair is listed at most once; (x, y) and (y, x) are considered the same (so they are never listed in the same test). If some pair of vertices is not listed in the input, then there exists an edge between those vertices.

Output

Firstly print k — the number of connected components in this graph.

Then print k integers — the sizes of components. You should output these integers in non-descending order.

Example

input

Copy

5 5

1 2

3 4

3 2

4 2

2 5

output

2

1 4 

题目大意：

对给定的一张图，求其补图的联通块个数及大小。

用广搜，每一次枚举u的边，标记所有目标点v，然后找出所有未标记的点加入队列

这样有点慢，考虑一个点加入了联通块，那么枚举时就不要考虑，用链表遍历

用一个链表，这样每个点只会有几种情况

1.已加入联通块，已经从链表删去

2.未加入联通块，且没有边相连，进入队列，最多$O(n)$

3.有边，那么不管，最多$O(m)$

所以复杂度$O(n+m)$

#include<iostream>
#include<cstdio>
#include<cstring>
#include<algorithm>
#include<cmath>
#include<vector>
#include<queue>
using namespace std;
int n,m,nxt[200001],lst[200001],ans[200001],cnt;
bool vis[200001],pd[200001];
queue<int>Q;
vector<int>G[200001];
void delet(int x)
{
    nxt[lst[x]]=nxt[x];
    lst[nxt[x]]=lst[x];
}
int main()
{int i,u,v,j;
    cin>>n>>m;
    for (i=1;i<=m;i++)
    {
        scanf("%d%d",&u,&v);
        G[u].push_back(v);
        G[v].push_back(u);
    }
    for (i=1;i<n;i++) nxt[i]=i+1,lst[i+1]=i;
    nxt[0]=1;
    for (i=1;i<=n;i++)
    if (vis[i]==0)
    {
        ans[++cnt]=1;vis[i]=1;
        Q.push(i);delet(i);
        while (Q.empty()==0)
        {
            int u=Q.front();
            Q.pop();
            for (j=0;j<G[u].size();j++)
            if (vis[G[u][j]]==0) pd[G[u][j]]=1;
            for (j=nxt[0];j;j=nxt[j])
            {
                if (pd[j]==0) 
                {
                    vis[j]=1;
                    ans[cnt]++;
                    Q.push(j);
                    delet(j);
                }
                else pd[j]=0;
            }
        }
    }
    sort(ans+1,ans+cnt+1);
    cout<<cnt<<endl;
    for (i=1;i<cnt;i++)
    printf("%d ",ans[i]);
    cout<<ans[cnt]<<endl;
}