Count（广工14届竞赛）

题目链接：http://acm.hdu.edu.cn/showproblem.php?pid=6470

这道题目题解就扔了个矩阵快速幂啥都没写。。。。。这题解是太看得懂我这个弱鸡了。

既然是矩阵快速幂那么先扔个矩阵快速幂的学习链接：https://www.luogu.org/problemnew/show/P3390

废话不说上图。这个是斐波那契数列的矩阵的推导。

既然有这种骚东西，肯定有其他的递推式。然后就是我们JX大佬给的神图，我研究半天才懂

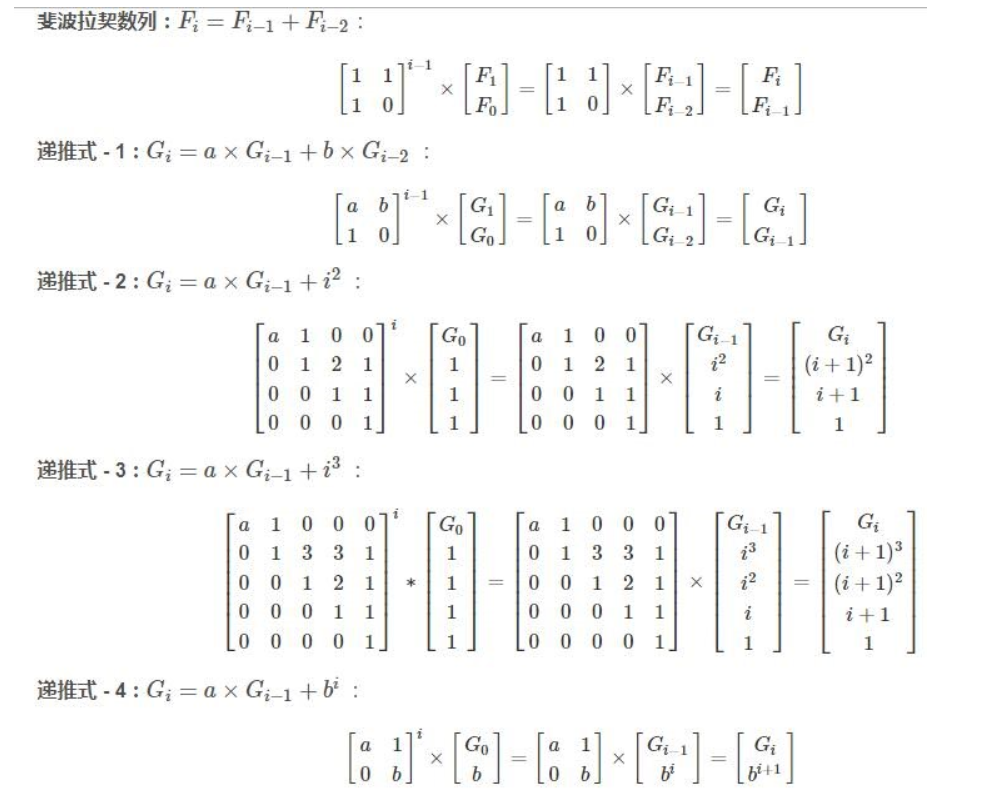
 有了这些工具，那么这道题目就可以解决了，继续上我的推导过程。

 很明显，这道题n从3开始，那么快速幂矩阵时的次方应该是n-2。好了，重点都讲完了，上代码。。。

#include <bits/stdc++.h>
using namespace std;
#define re register
#define ll long long
const int mod=123456789;
void read(int &a)
{
    a=0;
    int d=1;
    char ch;
    while(ch=getchar(),ch>'9'||ch<'0')
        if(ch=='-')
            d=-1;
    a=ch-'0';
    while(ch=getchar(),ch>='0'&&ch<='9')
        a=a*10+ch-'0';
    a*=d;
}
void write(int x)
{
    if(x<0)
        putchar(45),x=-x;
    if(x>9)
        write(x/10);
    putchar(x%10+'0');
}
int n=6;
struct note
{
    int a[10][10];
};
note ans,a,b;
void init()
{
    a.a[1][1]=1;
    a.a[1][2]=2;
    a.a[1][3]=1;
    a.a[1][4]=3;
    a.a[1][5]=3;
    a.a[1][6]=1;
    a.a[2][1]=1;
    a.a[2][2]=0;
    a.a[2][3]=0;
    a.a[2][4]=0;
    a.a[2][5]=0;
    a.a[2][6]=0;
    a.a[3][1]=0;
    a.a[3][2]=0;
    a.a[3][3]=1;
    a.a[3][4]=0;
    a.a[3][5]=0;
    a.a[3][6]=0;
    a.a[4][1]=0;
    a.a[4][2]=0;
    a.a[4][3]=1;
    a.a[4][4]=1;
    a.a[4][5]=0;
    a.a[4][6]=0;
    a.a[5][1]=0;
    a.a[5][2]=0;
    a.a[5][3]=1;
    a.a[5][4]=2;
    a.a[5][5]=1;
    a.a[5][6]=0;
    a.a[6][1]=0;
    a.a[6][2]=0;
    a.a[6][3]=1;
    a.a[6][4]=3;
    a.a[6][5]=3;
    a.a[6][6]=1;
    b.a[1][1]=2;
    b.a[1][2]=1;
    b.a[1][3]=1;
    b.a[1][4]=2;
    b.a[1][5]=4;
    b.a[1][6]=8;
}
note Mat(note x,note y)
{
    note c;
    for(re int i=1;i<=6;i++)
        for(re int j=1;j<=6;j++)
            c.a[i][j]=0;
    for(re int i=1;i<=6;i++)
        for(re int j=1;j<=6;j++)
            for(re int k=1;k<=6;k++)
                c.a[i][j]=(c.a[i][j]+1ll*x.a[i][k]*y.a[k][j]%mod)%mod;
    return c;
}
int main()
{
    int T;
    read(T);
    while(T--)
    {
        ll n;
        scanf("%lld",&n);
        n-=2;
        init();
        for(re int i=1;i<=6;i++)
            for(re int j=1;j<=6;j++)
                ans.a[i][j]=0;
        for(re int i=1;i<=6;i++)
            ans.a[i][i]=1;
        while(n)
        {
            if(n&1)
                ans=Mat(ans,a);
            a=Mat(a,a);
            n>>=1;
        }
        int sum=0;
        for(re int i=1;i<=6;i++)
            sum=(sum+1ll*ans.a[1][i]*b.a[1][i]%mod)%mod;
        write(sum);
        putchar('
');
    }
    return 0;
}

 代码应该很好懂，我就不注释了 Orz