【434】COMP9024 Exercises Revision

目录：

1. Week01
2. Week02
3. Week03
4. Week04
5. Week05
6. Week06
7. Week07
8. Week08
9. Week09
10. Week10

---

01. Week01

• 数字通过 #define 来定义，可读性更高，另外就是修改方便
  不要忘记 return EXIT_SUCCESS; or return 0;
  fiveDigit.c
  
  
• 计算矩阵內积的时候，其实看上去蛮复杂的
  方法：将计算过程写出来，自己找到规律，找到遍历的逻辑
  innerProdFun.c
  matrixProdFun.c
  
  
• 可以通过表达式来实现 if 语句
  

   int fastMax(int a, int b, int c) {
      int d = a * (a >= b) + b * (a < b);   // d is max of a and b
      return  c * (c >= d) + d * (c < d);   // return max of c and d
   }
  

  fastMax

02. Week02

• 测试系统
  int：4 bytes
  float：4 bytes
  pointer：8 bytes
  不足 4 bytes 的补齐
  opal.c （注意struct分级构建，重命名之类的）
  注意字符串数组保留最后一位
    3. The data structure can be improved in various ways:
       * encode both origin and destination (''from'' and ''to'') using
         Sydney Transport's unique stop IDs along with a lookup table that
         links e.g. 203311 to "Anzac Pde Stand D at UNSW"
       * use a single integer to encode the possible "Fare Applied" entries
       * avoid storing redundant information like the weekday, which can be
         derived from the date itself
    */
  
  
• fprintf 的下一句，可以是 return 1 or exit(1)
  最简单的 Makefile
  sumnum: sumnum.c
      gcc sumnum.c -o sumnum
      
  clean:
      rm -f sumnum
  sumnum.c
• 递归的思路就是找出递推公式，然后找到第一项的成立条件
  类似以前学的递推数列的思路，肯定会给一个关系和前几个值
  recount+.c

03. Week03

• atoi() does no error detection: it simply returns 0 if its argument is non-integer.
  sum3argB.c
  
  
• getchar() 一个字符一个字符读取，按照 不等于 EOF 可以判断是否到达文件结尾
  scanf() 可以读取一行
  如果读取多行的字符串，只需利用while循环即可，知道无法获取合理的字符串为止

	char str[10];
	while (scanf("%s", str) == 1) {
		printf("%s
", str);
	}

04. Week04

• 数组的地址运算，不是直接加数字，需要乘以数据类型本身占据的空间(bytes)
  int nums[12] = {5,3,6,2,7,4,9,1,8};
  and assuming that &nums[0] is 0xbabeface, what are the values of the following expressions?
  nums + 4
  类似 *(nums + 4) 表示 index 为 4 的元素
  所以 nums + 4 == 0xbabeface + 4*4bytes == 0xbabefade
  pointers.txt
  
  
• Albert 给的答案中，都是用“==”来表示结果的
  因为在 C 语言中 = 表示赋值
  == 表示等于的判断
  另外在写的时候，可以使用制表符，这样可能看上去舒服一点
  structs.txt
• 关于 stderr 的下一句
  可以是 exit(1);
  return 1;
  return EXIT_FAILURE;
  为了统一起见，按照老师代码规则，尽量使用 return
  因为 Albert 后面的练习答案也是用的 return EXIT_FAILURE;
  base2.c
• 判断字符串的结尾 '' 或者 0，两者一样，一个是 char 一个是 int 表示一个东西
  prefixes.c
  这道题目，Albert 的解法真是厉害，通过对于一个字符串，分别获取第一个字符的和最后一个字符的指针
  然后将最后一个字符逐渐向前移动，并且每次重新赋值最后一个字符为 ''
  然后分别打印字符串，每次字符串不同，每次都是逐渐变短
  Hilarious: 每次通过 sscanf 读取字符串，其实字符可以直接用，毕竟参数就是字符串 argv[]

 

05. Week05

• 用 malloc 分配内存的时候，可以使用 struct student 也可以用 typedef 的名称 如 Student，以下等效
  • malloc(sizeof(struct student))
  • malloc(sizeof(Student))
• 所有的指针都需要分配空间，并且后面都需要释放空间 free 指针，一一对应
• 对于 string 分配空间，需要增加一个位置给 ''
  
  • malloc(sizeof(char)*(strlen(name)+1))
    
    
• scanf 读取字符串时候，定义的字符串需要使用数组形式，或者malloc
  我自己理解：如果不能直接为字符串赋值，或者不等引用地址的话，就需要malloc分配空间，切记!!!

06. Week06

• 字符串比较使用 strcmp()，不能直接通过 == 来比较，后者是比较地址
  args2heap.c
• isalnum() ctype.h 用来判断字符是否为字母数字
  cHeap.c

07. Week07

• Hamilton cycle and path 没有判断定理
  只能自己来具体看
  有 Hamilton cycle 肯定有 Hamilton path
  Eulerian cycle：度都是偶数
  Eulerian path：两个度是奇数，其他都是偶数
  HamiltonEuler.txt
• 使用 Edge e 的时候，需要判断是否有效，因为如果数字过大，会引起错误的
  对于 Graph 或者 LinkedList 等，再补充的时候
  要特别注意判断是否为 NULL （虽然基本都不是）
• Notice removeE() is static, which mean it is allowed to be called only from within the ADT.
  同时需要将 g->nE 减少一个
  删除 链表 node 的时候，要考虑是不是删除 head，需要特别考虑（if...else...）
  
  

08. Week08

• Spnanning Tree：通过图生成的树，没有回路，必须经过所有顶点

• a. path

  • smallest: any path with one edge (e.g. a-b or g-m)

  • largest: some path including all nodes (e.g. m-g-h-k-i-j-a-b-c-d-e-f)

• b. cycle

  • smallest: need at least 3 nodes (e.g. i-j-k-i or h-i-k-h)

  • largest: path including most nodes (e.g. g-h-k-i-j-a-b-c-d-e-f-g) (can't involve m)

• c. spanning tree（连接所有顶点的树）

  • smallest: any spanning tree must include all nodes (the largest path above is an example)

  • largest: same

• d. vertex degree

  • smallest: there is a node that has degree 1 (vertex m)

  • largest: in this graph, 5 (b or f)

• e. clique

  • smallest: any vertex by itself is a clique of size 1（任何一个点都满足）

  • largest: this graph has a clique of size 5 (nodes b,c,d,e,f)
    
    GraphFundamentals.txt

• 邻接矩阵 Adjacent Matrix
• 邻接链表 Adjacent List
  

  a.
   nV     |6|     Adjacency Matrix
   nE     |6|     [0] [1] [2] [3] [4] [5]
   edges  |.|----> 0   1   1   0   0   0
                   1   0   0   1   0   0
                   1   0   0   1   1   0
                   0   1   1   0   0   0
                   0   0   1   0   0   1
                   0   0   0   0   1   0
  				 
  				 
  b.
   nV     |6|
   nE     |6|      Adjacency List
   edges	|.|----> |.|--> 1 --> 2 
                   |.|--> 0 --> 3 --> 4
                   |.|--> 0 --> 3 
                   |.|--> 1 --> 2
                   |.|--> 2 --> 5
                   |.|--> 4 
  

  GraphRepresentations.txt　　

• GraphStorageCosts.txt

• For the purposes of this exercise you may assume:

  • a pointer is 8 bytes long, an integer is 4 bytes
  • a vertex is an integer
  • a linked list node stores an integer
  • an adjacency matrix element is an integer

• For a given graph:

  1. Analyse the precise storage cost for the adjacency matrix (AM) representation of a graph of V vertices and E edges.

  2. Do the same analysis for the adjacency list (AL) representation.

  3. Determine the approximate V:E ratio at which point it is more storage efficient to use an AM representation than an AL representation.

     • If a graph has 100 vertices, how many edges should it have for AM to be more storage efficient than AL?

  4. You can save space by making the adjacency matrix an array of bytes instead of integers. What difference does that make to the ratio? 

• 【注意】写数学表达式的时候注意乘号，类似编程一样
  另外，有些答案好像写反了。。
  

  a. 
  An AM representation requires a V*V matrix of 4 bytes each, regardless of the number of edges.
  It also requires an array of V pointers.
  This gives a size of 4*V*V + 8*V.
  不需要考虑 edges 本身的地址，因为其与 edges[0] 共用一个地址
  
  b.
  An AL representation requires an array of V pointers (the starts of the lists),
  and then one node for each edge in each list.
  The total number of edges is 2*E.
  A node is an integer plus a pointer, which is 4+8 bytes.
  This gives 8*V + 12*2*E.
  点会重复，因此需要乘以2
  
  c.
  So if 8*V + 12*2*E < 4*V*V + 8*V then the AL version requires less storage.
  Simplifying, if E < V*V/6 then AL requires less storage.
  只需要建立不等式即可
  
  If you have a largish graph of 100 vertices, then up to about 1666 edges (which is a lot)
  it is better to use AL if storage is the main issue.
  As V gets very large, the factor 6 becomes insignificant.
  Hence asymptotically if the number of edges is less than V*V then AL is more space efficient than AM.
  解不等式即可
  
  d.
  If the adjacency matrix is just 1 byte instead of 4 bytes, then the formula is E < V*V/24.
  原来的4变成1
  

• DepthAndBreadthFirst.txt

• Consider the following graph:

  

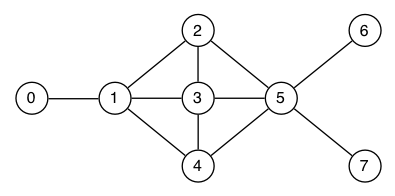
  Show the contents of the stack or queue in a 'search' traversal of the graph using:

  1. depth-first search starting at vertex 0

  2. depth-first search starting at vertex 3

  3. breadth-first search starting at vertex 0

  4. breadth-first search starting at vertex 3 

• 对于 stack 和 queue
  stack 是 左边压入 左边弹出，push，pop
  queue 是 右边压入 左边弹出，qush，pop
  stack 是 从大到小 push
  queue 是 从小到大 qush
  最终目的就是让小的数据先被 pop

• 
  对于 DFS，算法不会加入前面已经 Pop 的点
  但是还是会加入存在 stack 内部的点
  然后是 top 在左，从 top 往里面放入，从大到小，显示的效果就是小的在上面
  从左边压入数据，从左边弹出数据
  
  

  a. DFS starting at 0:
     Pop      Stack (top at left)
     -                  0
     0                  1.
     1              2 3 4.
     2                3 5. 3 4
     3                4 5. 5 3 4
     4                  5. 5 5 3 4
     5                6 7. 5 5 3 4
     6                   . 7 5 5 3 4
     7                   .   5 5 3 4
                         .
     where above the '.' shows the newly pushed adjacent vertices
  
  b. DFS starting at 3:
     Pop    Stack (top at left)
     -                3
     3          1 2 4 5.
     1            0 2 4. 2 4 5
     0                 . 2 4 2 4 5
     2                5. 4 2 4 5
     5            4 6 7. 4 2 4 5
     4                 . 6 7 4 2 4 5
     6                 . 7 4 2 4 5
     7                 . 4 2 4 5
                       .
     where I've left out the all visited pops
  

  
  对于 BFS 算法，算法不会加入前面出现过的点
  然后是 head 在左，从右边压入数据，从左边弹出
  压入数据也是从小到大，显示效果就是小的在前面

  c. BFS starting at 0:
     Pop    Queue (head at left)
     -              0
     0              . 1
     1              . 2 3 4
     2           3 4. 5
     3           4 5.
     4             5.
     5              . 6 7
     6             7.
     7              .
  
  d. BFS starting at 3:
     Pop    Queue (head at left)
     -                3
     3                 . 1 2 4 5
     1            2 4 5. 0
     2            4 5 0.
     4              5 0.
     5                0. 6 7
     0              6 7.
     6                7.
     7                 . 
  

• VisitedArray.txt

• Consider the following 2 graphs (the right graph is missing edge 7-8):

      0--1--2             0--1--2
      |  |  |             |  |  |
      3--4--5             3--4--5
      |  |  |             |  |  |
      6--7--8             6--7  8
  

  Starting at vertex 0, and using a depth-first search (assume the smallest vertex is selected):

  1. compute the visited array for the graph on the left

     • can you deduce a path from vertex 0 to vertex 8 from the visited array?

  2. compute the visited array for the graph on the right

     • can you deduce a path from vertex 0 to vertex 8 from the visited array?

  Can you draw any conclusions? 

• {{{
      0--1--2             0--1--2
      |  |  |             |  |  |
      3--4--5             3--4--5
      |  |  |             |  |  |
      6--7--8             6--7  8
  }}}
   a. DFS visited = {0, 1, 2, 5, 4, 3, 6, 7, 8}
    It is tempting to say that the path is indicated by the visited array, but this is wrong,
    as we shall see.
   b. DFS visited = {0, 1, 2, 5, 4, 3, 6, 7, 8}
    This array is the same as the previous. Clearly the path from vertex 0 to 8 cannot
    include edge 7--8, so the visited array does not indicate a path.
  
  The visited arrays are the same because 'backtracking' is not visible in the visited array:
   * when DFS reaches vertex 5, it places vertex 8 on the stack, and continues with vertex 4
   * when DFS reaches vertex 7, this branch traversal concludes, and it continues with
     the vertex 8 that remains on the stack
   * it does not matter whether there is an edge 7-8 or not, the visited arrays are identical
  
  Conclusion: a visited array does not show the path
  

09. Week09

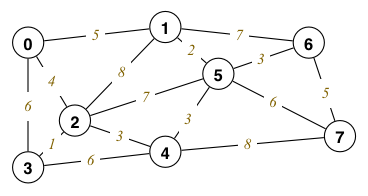
• Dijkstra.txt

• Dijkstra's algorithm computes the minimum path costs from the source node 0 to all the other vertices, resulting in the Shortest Path Tree (SPT). For the following graph:

  • 

  1. show the order that vertices are visited, and the path cost pacost[] of each of the vertices

     • (when faced with a choice, select the edge to the lowest vertex)

  2. there are 4 vertices that undergo non-trivial edge relaxation. What are they, and what is the reduction in cost for each vertex?

  3. draw the SPT 

• a. 按照访问顺序填写，pacost[0] = 0.00，另外注意是 float
  b. non-trivial 表示具体数字的变化，注意符号 ==>，以及 float
  c. 绘制 SPT 的时候，尽量按照具体数字顺序，小的在左，大的在右
  
  

  Actions taken by Dijkstra's Algorithm
  
  a. The visit order of the vertices, together with the path costs
  
     visit 0, pacost[0] = 0.00
     visit 2, pacost[2] = 4.00
     visit 1, pacost[1] = 5.00
     visit 3, pacost[3] = 5.00
     visit 4, pacost[4] = 7.00
     visit 5, pacost[5] = 7.00
     visit 6, pacost[6] = 10.00
     visit 7, pacost[7] = 13.00
  
  b. The 4 vertices that are relaxed are 3, 5, 6 and 7
     pacost[3] = 6.00  ==> 5.00
     pacost[5] = 11.00  ==> 7.00
     pacost[6] = 12.00  ==> 10.00
     pacost[7] = 15.00  ==> 13.00
  
  c. The SPT is:
  
                   0
                 /   
                1     2
                |    / 
                5   3   4
               / 
              6   7
  

• Prim.txt

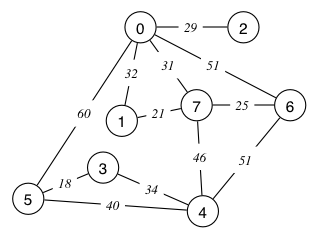
• Prim's Algorithm generates a Minimum Spanning Tree (MST). For the following graph:

  • 

  1. by hand, use the sets mst and rest to build an MST one vertex at a time Do this in the form of a table, where the first 2 lines have been completed for you.

     mst	rest vertex	cost
     {0}	2	29
     {0,2}	7	31
     ...	...	...

  2. draw the MST
  3. how many edges in the MST?
  4. what is the cost of the MST? 

• Actions taken by Prim's Algorithm using sets MST and REST
  
  a.
                    new
   MST             vertex   cost
   {0}                2      29
   {0,2}              7      31
   {0,2,7}            1      21
   {0,2,7,1}          6      25
   {0,2,7,1,6}        4      46
   {0,2,7,1,6,4}      5      18
   {0,2,7,1,6,4,5}
  
  b.
        0
      /   
     2     7
         / | 
        1  6  4
              |
              3
              |
              5
  
  c. 7 edges
  
  d. cost is 204
  

• Kruskal.txt

• Kruskal's Algorithm also generates an MST. For the following graph:

  • 

  1. show which edges are considered at each step and ...
     • whether the edge gets accepted, or rejected (because it causes a cycle)
  2. draw the MST.
     • is the MST the same of different to the MST generated by Prim's algorithm? 

• 【注意】cost 用 float 表示，另外就是格式
  还有就是 Prim 和 Kruskal 算法的结果一致
  
  

  Actions taken by Kruskal's algorithm
  
  a.
    Accept 3-5: 18.00
    Accept 1-7: 21.00
    Accept 6-7: 25.00
    Accept 0-2: 29.00
    Accept 0-7: 31.00
                     Reject 0-1: 32.00
    Accept 3-4: 34.00
                     Reject 4-5: 40.00
    Accept 4-7: 46.00
  
  b. Same as Prim's MST.
  

• stdin 的时候，就是 通过 scanf() 或者 getchar() 等按照顺序读取
  对于第一次开始的 white space 不能直接跳过，通过下面代码跳过
  

  char space[100];
  scanf("%[ /t/n]", space);

  上面的代码可以跳过开头不确定的 空格、制表符、回车键 等。
  然后继续读取井号，通过 getchar() 实现
  一次只读取一个字符，然后通过 scanf() 每次读取一对数据，这地方对于格式有要求
  判断条件可以是 scanf("%d %d", &v1, &v2) != EOF
  也可以是 scanf("%d %d", &v1, &v2) == 2
  经验证，两种方法均可
  

     #6
     0 1 0 2 1 2 3 4 3 5 4 5

• 实现代码：

• // By Alex
  
  int read_num() {
  	char space[100];
  	scanf("%[ /t/n]", space);
  	getchar();
  	int num;
  	scanf("%d", &num);
  	return num;
  }
  
  Graph read_graph(int nV) {
  	int v, w;
  	Graph g;
  	g = newGraph(nV);
  	while (scanf("%d %d", &v, &w) != EOF) {
  	//while (scanf("%d %d", &v, &w) == 2) {
  		Edge e;
  		e = newE(v, w);
  		insertE(g, e);
  	}
  	return g;
  }
  

  　　

• // By Albert
  
  #define WHITESPACE 100
  
  int readNumV(void) { // returns the number of vertices numV or -1
     int numV;
     char w[WHITESPACE];
     scanf("%[ 	
  ]s", w);  // skip leading whitespace
     if ((getchar() != '#') ||
         (scanf("%d", &numV) != 1)) {
         fprintf(stderr, "missing number (of vertices)
  ");
         return -1;
     }
     return numV;
  }
  
  int readGraph(int numV, Graph g) { // reads number-number pairs until EOF
     int success = true;             // returns true if no error
     int v1, v2;
     while (scanf("%d %d", &v1, &v2) != EOF && success) {
         if (v1 < 0 || v1 >= numV || v2 < 0 || v2 >= numV) {
            fprintf(stderr, "unable to read edge
  ");
            success = false;
         }
         else {
            insertE(g, newE(v1, v2));
         }
     }
     return success;
  }
  

  　　

• 使用 iteration 实现 unreachable
  思路，首先将 visited[0] = 1，其他均为访问
  然后无限循环，从里面找一个未访问的节点，然后便利其邻接点，若存在访问过的节点
  则标记一下，另外就是标记这个点访问过，直到退出循环
  

  // By Alex
  
  int main() {
  	int nV = read_num();
  	Graph g = read_graph(nV);
  	showGraph(g);
  	
  	int visited[nV];
  	visited[0] = 1;
  	for (int i = 1; i < nV; i++) {
  		visited[i] = 0;
  	}
  	
  	int one_graph_all_vis = 0;
  	
  	while (!one_graph_all_vis) {
  		one_graph_all_vis = 1;
  		for (int v = 0; v < nV; v++) {
  			if (!visited[v]) {
  				for (int w = 0; w < nV; w++) {
  					if (visited[w] && isEdge(g, newE(v, w))) {
  						visited[v] = 1;
  						one_graph_all_vis = 0;
  					}
  				}
  			}
  		}
  	}
  	
  	int unreachable = 0;
  	for (int i = 0; i < nV; i++) {
  		if (!visited[i]) {
  			unreachable = 1;
  		}
  	}
  	
  	if (unreachable) {
  		printf("Unreachable vertices = ");
  		for (int i = 0; i < nV; i++) {
  			if (!visited[i]) {
  				printf("%d ", i);
  			}
  		}
  		putchar('
  ');
  	}
  	else{
  		printf(" Unreachable vertices = none
  ");
  	}
  	
  	return 0;
  }
  

  　　

10. Week10

• Heap-Order Property (sometimes called HOP)
  Complete-Tree Property (sometimes called CTP)
  

  a. BST because it has Left-Right order
  b. Heap because it satisfies HOP and CTP
  c. Heap because it satisfies HOP and CTP
  d. It is neither a BST nor a heap. It is just a binary tree.
  e. Could be either. It has L-R order, and it satisfies the HOP and CTP.
  f. Could be either. It has L-R order, and it satisfies the HOP and CTP
  

• BSTrootleafinsert.txt

• Consider an initially empty BST and the sequence of values 1 2 3 4 5 6.

  • Show the tree resulting from inserting these values "at the leaf". What is its height?

  • Show the tree resulting from inserting these values "at the root". What is its height?

  • Show the tree resulting from an alternate at-root-insertion and at-leaf-insertion, starting with at-the-leaf. What is its height?

• 说明：at the leaf：说明直接插入到 leaf 即可，不需要移动

  at the root：说明需要调整到 root，但是一个一个调整
  
  

• Splaying.txt
• Given an initially empty splay tree:

  1. show the changes in the splay tree after each splay-insertion of the node values 5 3 8 7 4

• Given an initially empty splay tree:

• 1. show the changes in the splay tree after each splay-insertion of the node values b c d e f g ...

  2. and then the splay tree after a search for node a ...

  3. and then the splay tree after a search for node d

  说明：如果 search 不到元素，就将那个元素 splay，按照 zigzag 或者 zigzig 规则操作