HDU 1506 Largest Rectangle in a Histogram

Problem Description

A histogram is a polygon composed of a sequence of rectangles aligned at a common base line. The rectangles have equal widths but may have different heights. For example, the figure on the left shows the histogram that consists of rectangles with the heights 2, 1, 4, 5, 1, 3, 3, measured in units where 1 is the width of the rectangles:

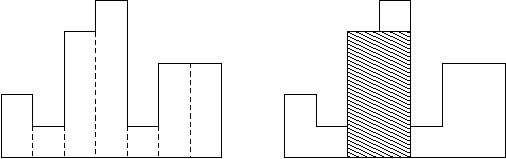
Usually, histograms are used to represent discrete distributions, e.g., the frequencies of characters in texts. Note that the order of the rectangles, i.e., their heights, is important. Calculate the area of the largest rectangle in a histogram that is aligned at the common base line, too. The figure on the right shows the largest aligned rectangle for the depicted histogram.

 

Input

The input contains several test cases. Each test case describes a histogram and starts with an integer n, denoting the number of rectangles it is composed of. You may assume that 1 <= n <= 100000. Then follow n integers h1, ..., hn, where 0 <= hi <= 1000000000. These numbers denote the heights of the rectangles of the histogram in left-to-right order. The width of each rectangle is 1. A zero follows the input for the last test case.

 

Output

For each test case output on a single line the area of the largest rectangle in the specified histogram. Remember that this rectangle must be aligned at the common base line.

 

Sample Input

7 2 1 4 5 1 3 3
4 1000 1000 1000 1000
0

 

Sample Output

8
4000

 

Source

University of Ulm Local Contest 2003

 

事实上也不难理解，策略：对于每个点都要记录当前的高度a[i],然后就是左右延伸比当前高度a[i]高的

最远位子，即l[i],r[i];

#include<iostream>
#include<cstdio>
#include<cstring>
#include<algorithm>
#include<limits.h>
using namespace std;
const int maxn=100000+100;
long long a[maxn],n;
long long l[maxn],r[maxn];//r[i]记录比当前点大右边的位子，l[i]记录比当前点大的左边的位子。
int main()
{
    while(~scanf("%I64d",&n)&&n)
    {
        for(int i=1;i<=n;i++)
           scanf("%I64d",&a[i]);
        l[1]=1;
        r[n]=n;
        for(int i=2;i<=n;i++)
        {
            int t=i;
            while(t>1&&a[i]<=a[t-1])
                t=l[t-1];
            l[i]=t;
        }
        for(int i=n-1;i>=1;i--)
        {
            int t=i;
            while(t<n&&a[i]<=a[t+1])
                t=r[t+1];
            r[i]=t;
        }
        long long ans=-1;
        for(int i=1;i<=n;i++)
        {
            if((r[i]-l[i]+1)*a[i]>ans)
                ans=(r[i]-l[i]+1)*a[i];
        }
        printf("%I64d
",ans);
    }
    return 0;
}