CodeForces 344C Rational Resistance

C. Rational Resistance 

time limit per test 

1 second

memory limit per test 

256 megabytes

input 

standard input

output 

standard output

Mad scientist Mike is building a time machine in his spare time. To finish the work, he needs a resistor with a certain resistance value.

However, all Mike has is lots of identical resistors with unit resistance R0 = 1. Elements with other resistance can be constructed from these resistors. In this problem, we will consider the following as elements:

1. one resistor;
2. an element and one resistor plugged in sequence;
3. an element and one resistor plugged in parallel.

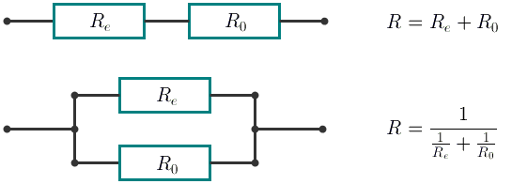
With the consecutive connection the resistance of the new element equals R = Re + R0. With the parallel connection the resistance of the new element equals 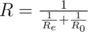. In this case Re equals the resistance of the element being connected.

Mike needs to assemble an element with a resistance equal to the fraction . Determine the smallest possible number of resistors he needs to make such an element.

Input

The single input line contains two space-separated integers a and b (1 ≤ a, b ≤ 10^18). It is guaranteed that the fraction  is irreducible. It is guaranteed that a solution always exists.

Output

Print a single number — the answer to the problem.

Please do not use the %lld specifier to read or write 64-bit integers in С++. It is recommended to use the cin, cout streams or the%I64d specifier.

Sample test(s)

input

1 1

output

1

input

3 2

output

3

input

199 200

output

200

Note

In the first sample, one resistor is enough.

In the second sample one can connect the resistors in parallel, take the resulting element and connect it to a third resistor consecutively. Then, we get an element with resistance 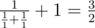. We cannot make this element using two resistors.

题意：给出a,b，问最少用多少阻值为1的电阻通过串并联的方式得到阻值为a/b的电阻，a/b是最简形式

分析：如果a==b，既然是最简，那么就只有等于1的情况

　　　如果a>b，那么化成假分数，整数部分用阻值为1的电阻串联即可，剩下就得到a'<b

　　　如果a<b，有个结论，引用一下：如果最少用K个电阻构成a/b Ω电阻,那么b/a也需K个(只需改变所有的串并联关系即可)

那么分好情况就好做了，注意结果可能会超int

#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<cstdio>
#include<string>
#include<iostream>
#include<cstring>
#include<cmath>
#include<stack>
#include<queue>
#include<vector>
#include<map>
#include<stdlib.h>
#include<algorithm>
#define LL __int64
using namespace std;
int main()
{
    LL a,b,ans=0;
    scanf("%I64d %I64d",&a,&b);
    if(a==b) printf("1
");
    else
    {
        while(a!=b)
        {
            if(a==0) break;
            if(a<b) swap(a,b);
            ans+=a/b;
            a%=b;
        }
        printf("%I64d
",ans);
    }
    return 0;
}