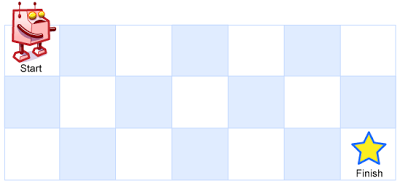
A robot is located at the top-left corner of a m x n grid (marked 'Start' in the diagram below).
The robot can only move either down or right at any point in time. The robot is trying to reach the bottom-right corner of the grid (marked 'Finish' in the diagram below).
Now consider if some obstacles are added to the grids. How many unique paths would there be?
An obstacle and empty space is marked as 1 and 0 respectively in the grid.
Note: m and n will be at most 100.
Example 1:
Input: [ [0,0,0], [0,1,0], [0,0,0] ] Output: 2 Explanation: There is one obstacle in the middle of the 3x3 grid above. There are two ways to reach the bottom-right corner: 1. Right -> Right -> Down -> Down 2. Down -> Down -> Right -> Right
解答:
这道题和62很相似,区别在于多了障碍物,不过仍然是用动态对规划的方式,有障碍物的位置对路线数量的贡献为0,更新方法仍然类似,不过可以直接在原来数组上进行更新,不需要新开辟空间
代码如下:
1 class Solution { 2 public: 3 int uniquePathsWithObstacles(vector<vector<int>>& obstacleGrid) { 4 if (obstacleGrid[0][0] == 1) 5 return 0; 6 int row = obstacleGrid.size(); 7 int col = obstacleGrid[0].size(); 8 obstacleGrid[0][0] = 1; 9 for (int i = 1; i < row; i++) 10 { 11 if (obstacleGrid[i][0] == 0) 12 obstacleGrid[i][0] = obstacleGrid[i - 1][0]; 13 else 14 obstacleGrid[i][0] = 0; 15 } 16 for (int i = 1; i < col; i++) 17 { 18 if (obstacleGrid[0][i] == 0) 19 obstacleGrid[0][i] = obstacleGrid[0][i - 1]; 20 else 21 obstacleGrid[0][i] = 0; 22 } 23 for (int i = 1; i < row; i++) 24 for (int j = 1; j < col; j++) 25 { 26 if (obstacleGrid[i][j] == 0) 27 obstacleGrid[i][j] = obstacleGrid[i - 1][j] + obstacleGrid[i][j - 1]; 28 else 29 obstacleGrid[i][j] = 0; 30 } 31 return obstacleGrid[row - 1][col - 1]; 32 33 } 34 };
时间复杂度:O(m*n)
空间复杂度:O(1)