hdu1710 Binary Tree Traversals（二叉树）

Binary Tree Traversals

Time Limit: 1000/1000 MS (Java/Others)    Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 15904    Accepted Submission(s): 6652

Problem Description

A binary tree is a finite set of vertices that is either empty or consists of a root r and two disjoint binary trees called the left and right subtrees. There are three most important ways in which the vertices of a binary tree can be systematically traversed or ordered. They are preorder, inorder and postorder. Let T be a binary tree with root r and subtrees T1,T2.

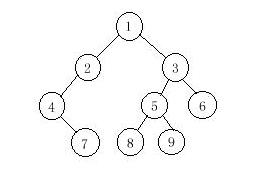
In a preorder traversal of the vertices of T, we visit the root r followed by visiting the vertices of T1 in preorder, then the vertices of T2 in preorder.

In an inorder traversal of the vertices of T, we visit the vertices of T1 in inorder, then the root r, followed by the vertices of T2 in inorder.

In a postorder traversal of the vertices of T, we visit the vertices of T1 in postorder, then the vertices of T2 in postorder and finally we visit r.

Now you are given the preorder sequence and inorder sequence of a certain binary tree. Try to find out its postorder sequence.

 

Input

The input contains several test cases. The first line of each test case contains a single integer n (1<=n<=1000), the number of vertices of the binary tree. Followed by two lines, respectively indicating the preorder sequence and inorder sequence. You can assume they are always correspond to a exclusive binary tree.

 

Output

For each test case print a single line specifying the corresponding postorder sequence.

 

Sample Input

9 1 2 4 7 3 5 8 9 6 4 7 2 1 8 5 9 3 6

 

Sample Output

7 4 2 8 9 5 6 3 1

 

Source

HDU 2007-Spring Programming Contest

 

Recommend

lcy   |   We have carefully selected several similar problems for you:  1711 1708 1707 1709 1509 

 

 

 

题意：给出二叉树的先序与中序遍历，输出后续遍历

 

 

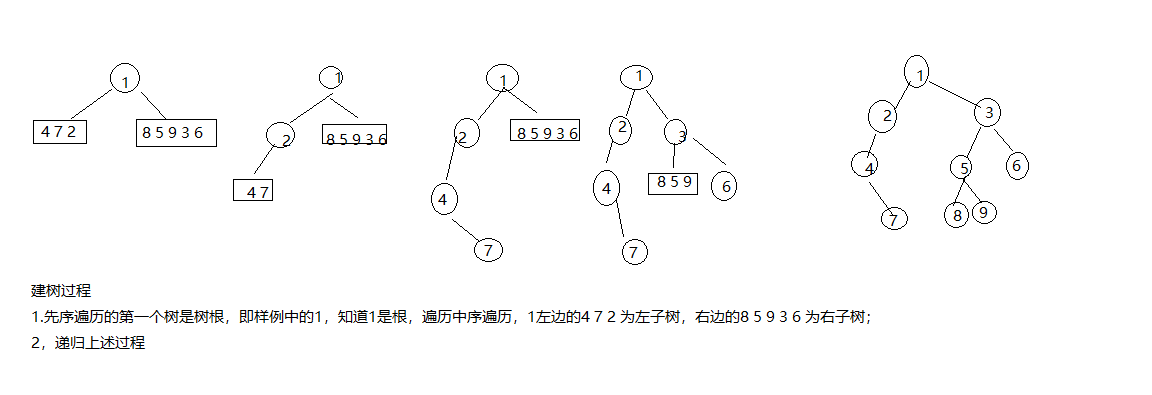
代码：

#include<bits/stdc++.h>
using namespace std;
const int N=1010;
int pre[N],in[N],post[N];//分别储存先，中，后续
int k;
struct node{
 int value;
 node *l,*r;
 node(int value=0,node *l=NULL,node *r=NULL):value(value),l(l),r(r){
 };
};
void buildtree(int l, int r, int &t, node* &root) { //建树
    int flag = -1;
    for(int i = l; i <= r; i++) //先序的第一个是根，找到对应的中序的位置
        if(in[i] == pre[t]){
            flag = i; break;
        }
    if(flag == -1) return;       //结束
    root = new node(in[flag]);   //新建结点
    t++;
    if(flag > l)  buildtree(l, flag - 1, t, root ->l);
    if(flag < r)  buildtree(flag + 1, r, t, root ->r);
}
void add(node * &root ,int & t ){
    if(root == NULL){
        root = new node;
        root->value=t;
        root->l=root->r=NULL;
    }else{
        if( t > root->value)
            add( root->r,t);
        else
            add(root->l,t);
    }
    
}
void preorder (node *root){       //求先序序列
    if(root != NULL){
        post[k++] = root ->value;  //输出
        preorder (root ->l);
        preorder (root ->r);
    }
}
void inorder (node *root){        //求中序序列
    if(root != NULL){
        inorder (root ->l);
        post[k++] = root ->value;  //输出
        inorder (root ->r);
    }
}
void postorder (node *root){      //求后序序列
    if( root != NULL){
        postorder (root ->l);
        postorder (root ->r);
        post[k++] = root ->value;  //输出
    }
}
void remove_tree(node *root){      //释放空间
    if(root == NULL) return;
    remove_tree(root->l);
    remove_tree(root->r);
    delete root;
}

int main(){
 int n;
 while(~scanf("%d",&n)){
  for(int i=1;i<=n;i++)scanf("%d",&pre[i]);
  for(int j=1;j<=n;j++)scanf("%d",&in[j]);
  node *root;
  int t=1;
  buildtree(1,n,t,root);
  k=0;
  postorder(root);
  for(int i=0;i<k;i++)printf("%d%c",post[i],i==k-1?' ':' ');
  remove_tree(root);
 }
    return 0;
}