一、数据结构介绍

二、列表结构
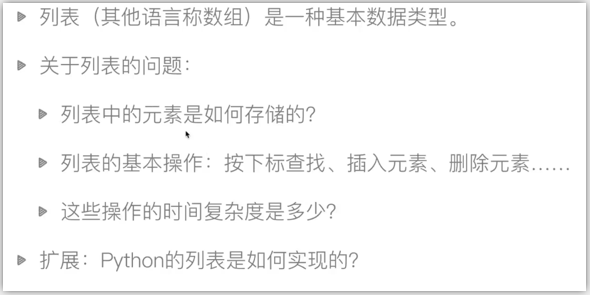
数组与列表两点不同:
1、数组元素类型要相同
2、数组长度固定(定义好就不变了)
列表里面存的是地址
列表可以增删
三、栈的结构----先进后出
递归的数据结构就是栈

取栈顶,只是确定值,不是取出来
class Stack: def __init__(self): self.stack = [] def push(self,element): self.stack.append(element) def pop(self): # pop() 函数用于移除列表中的一个元素(默认最后一个元素),并且返回该元素的值。 return self.stack.pop() def get_top(self): if len(self.stack)>0: return self.stack[-1] else: return None stack = Stack() stack.push(1) stack.push(2) stack.push(3) print(stack.pop()) print(stack.__dict__)
3
{'stack': [1, 2]}

栈的应用:
括号匹配问题

class Stack: def __init__(self): self.stack = [] def push(self,element): self.stack.append(element) def pop(self): # pop() 函数用于移除列表中的一个元素(默认最后一个元素),并且返回该元素的值。 return self.stack.pop() def get_top(self): if len(self.stack)>0: return self.stack[-1] else: return None def is_empty(self): return len(self.stack)==0
def brace_match(s): math = {'}':'{',']':'[',')':'('} stack = Stack() for ch in s: if ch in {'(','[','{'}: stack.push(ch) else: if stack.is_empty(): return False elif stack.get_top()==math[ch]: stack.pop() else: return False if stack.is_empty(): return True else: return False print(brace_match('[{()}{}]'))
队列的介绍----先进先出

front 英 /frʌnt/ 美 /frʌnt/ 前
rear 英 /rɪə/ 美 /rɪr/ 后
环形队列


class Queue: def __init__(self,size=100): self.queue = [0 for _ in range(size)] self.size = size self.rear = 0 # 队尾 self.front = 0 # 队首 def push(self,element): if not self.is_filled(): self.rear = (self.rear+1) % self.size self.queue[self.rear] = element else: raise IndexError('Queue is filled') def pop(self): if not self.is_empty(): self.front = (self.front+1) %self.size return self.queue[self.front] else: raise IndexError('Queue is empty') # 判断队空 def is_empty(self): return self.rear==self.front # 判断队满 def is_filled(self): return (self.rear+1)%self.size==self.front q = Queue(5) for i in range(4): q.push(i) print(q.pop()) q.push(5)
队列的内置模块
双向队列

from collections import deque # deque([iterable[, maxlen]]) --> deque object q = deque([1,2,3,4,5],5) q.append(6) # 队尾进队 print(q.popleft()) # 队首出队 # 用于双向队列 # q.appendleft(1) # 队首进队 # q.pop()
栈和队列的应用:迷宫问题

栈---深度优先(一条道走到黑)

maze 英 /meɪz/ 美 /mez/ 迷宫

maze = [ [1, 1, 1, 1, 1, 1, 1, 1, 1, 1], [1, 0, 0, 1, 0, 0, 0, 1, 0, 1], [1, 0, 0, 1, 0, 0, 0, 1, 0, 1], [1, 0, 0, 0, 0, 1, 1, 0, 0, 1], [1, 0, 1, 1, 1, 0, 0, 0, 0, 1], [1, 0, 0, 0, 1, 0, 0, 0, 0, 1], [1, 0, 1, 0, 0, 0, 1, 0, 0, 1], [1, 0, 1, 1, 1, 0, 1, 1, 0, 1], [1, 1, 0, 0, 0, 0, 0, 0, 0, 1], [1, 1, 1, 1, 1, 1, 1, 1, 1, 1] ] dirs = [ lambda x,y: (x+1,y), lambda x,y: (x-1,y), lambda x,y: (x,y-1), lambda x,y: (x,y+1) ] # 起点:(x1,y1);终点:(x2,y2) def maze_path(x1,y1,x2,y2): stack = [] stack.append((x1, y1)) while(len(stack)>0): curNode = stack[-1] # 当前的节点 if curNode[0] == x2 and curNode[1] == y2: # 走到终点了 for p in stack: print(p) return True # x,y 四个方向 x-1,y; x+1,y; x,y-1; x,y+1 # 得出结论:for 循环正常执行结束后,else 语句里面的内容也会正常执行。 for dir in dirs: nextNode = dir(curNode[0], curNode[1]) # 如果下一个节点能走 if maze[nextNode[0]][nextNode[1]] == 0: stack.append(nextNode) # 已经走过的标记一下 maze[nextNode[0]][nextNode[1]] = 2 # 2表示为已经走过 break else: maze[nextNode[0]][nextNode[1]] = 2 stack.pop() else: print("没有路") return False # (1,1)为0 (8,8)为0 -对角线 maze_path(1,1,8,8)
使用队列解决迷宫问题
队列----广度优先
先到的排序时间最短


from collections import deque maze = [ [1, 1, 1, 1, 1, 1, 1, 1, 1, 1], [1, 0, 0, 1, 0, 0, 0, 1, 0, 1], [1, 0, 0, 1, 0, 0, 0, 1, 0, 1], [1, 0, 0, 0, 0, 1, 1, 0, 0, 1], [1, 0, 1, 1, 1, 0, 0, 0, 0, 1], [1, 0, 0, 0, 1, 0, 0, 0, 0, 1], [1, 0, 1, 0, 0, 0, 1, 0, 0, 1], [1, 0, 1, 1, 1, 0, 1, 1, 0, 1], [1, 1, 0, 0, 0, 0, 0, 0, 0, 1], [1, 1, 1, 1, 1, 1, 1, 1, 1, 1] ] dirs = [ lambda x, y: (x + 1, y), lambda x, y: (x - 1, y), lambda x, y: (x, y - 1), lambda x, y: (x, y + 1) ] def print_r(path): curNode = path[-1] realpath = [] while curNode[2] == -1: realpath.append(curNode[0:2]) curNode = path[curNode[2]] realpath.append(curNode[0:2]) # 起点 realpath.reverse() for node in realpath: print(node) def maze_path_queue(x1, y1, x2, y2): queue = deque() queue.append((x1, y1, -1)) path = [] while len(queue) > 0: curNode = queue.pop() path.append(curNode) if curNode[0] == x2 and curNode[1] == y2: # 终点 print_r(path) return True for dir in dirs: nextNode = dir(curNode[0], curNode[1]) if maze[nextNode[0]][nextNode[1]] == 0: queue.append((nextNode[0], nextNode[1], len(path) - 1)) # 后续节点进队,记录哪个节点带他来的 maze[nextNode[0]][nextNode[1]] = 2 # 标记为已经走过 else: print("没有路") return False maze_path_queue(1, 1, 8, 8)
链表

node 英 /nəʊd/ 美 /nod/ 节点
链表的创建和遍历
头插法-------------------------------尾插法
class Node:
def __init__(self,item):
self.item = item
self.next = Node
def create_linklist_head(li): head = Node(li[0]) for element in li[1:]: node = Node(element) node.next = head head = node print(head.__dict__) return head # 尾插入 def create_linklist_tail(li): head = Node(li[0]) tail = head for element in li[1:]: node = Node(element) tail.next = node tail = node return head def print_linklist(lk): while lk: print(lk.item,end=',') lk= lk.next # 头插法 lk = create_linklist_head([1,2,3]) print(lk.__dict__) print_linklist(lk) # print(lk.next.item)
{'item': 2, 'next': <__main__.Node object at 0x0000000002258C50>}
{'item': 3, 'next': <__main__.Node object at 0x000000000225EAC8>}
{'item': 3, 'next': <__main__.Node object at 0x000000000225EAC8>}
3,2,1,
链表的插入和删除
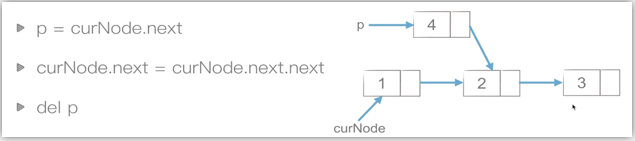
单链表只能从前往后找
双链表
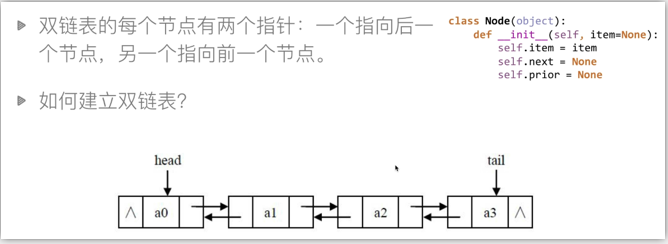
总结


