The Bottom of a Graph（tarjan + 缩点）

The Bottom of a Graph

Time Limit: 3000MS		Memory Limit: 65536K
Total Submissions: 9139		Accepted: 3794

Description

We will use the following (standard) definitions from graph theory. Let V be a nonempty and finite set, its elements being called vertices (or nodes). Let E be a subset of the Cartesian product V×V, its elements being called edges. Then G=(V,E) is called a directed graph. 
Let n be a positive integer, and let p=(e₁,...,e_n) be a sequence of length n of edges e_i∈E such that e_i=(v_i,v_i+1) for a sequence of vertices (v₁,...,v_n+1). Then p is called a path from vertex v₁ to vertex v_n+1in G and we say that v_n+1 is reachable from v₁, writing (v₁→v_n+1). 
Here are some new definitions. A node v in a graph G=(V,E) is called a sink, if for every node w in G that is reachable from v, v is also reachable from w. The bottom of a graph is the subset of all nodes that are sinks, i.e., bottom(G)={v∈V|∀w∈V:(v→w)⇒(w→v)}. You have to calculate the bottom of certain graphs.

Input

The input contains several test cases, each of which corresponds to a directed graph G. Each test case starts with an integer number v, denoting the number of vertices of G=(V,E), where the vertices will be identified by the integer numbers in the set V={1,...,v}. You may assume that 1<=v<=5000. That is followed by a non-negative integer e and, thereafter, e pairs of vertex identifiers v₁,w₁,...,v_e,w_e with the meaning that (v_i,w_i)∈E. There are no edges other than specified by these pairs. The last test case is followed by a zero.

Output

For each test case output the bottom of the specified graph on a single line. To this end, print the numbers of all nodes that are sinks in sorted order separated by a single space character. If the bottom is empty, print an empty line.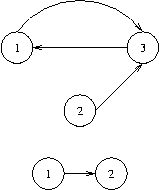

Sample Input

3 3
1 3 2 3 3 1
2 1
1 2
0

Sample Output

1 3
2

Source

Ulm Local 2003

参考代码这里：http://blog.csdn.net/ehi11/article/details/7884851

缩点：（这个概念也是看了别人的理解）先求有向图的强连通分量 ， 如果几个点同属于一个强连通 ， 那就给它们标上相同的记号 ， 这样这几个点的集合就形成了一个缩点。

 题目大意：求出度为0的强连通分量

#include<stdio.h>
#include<queue>
#include<string.h>
using namespace std;
const int M = 50010 ;
int n , m ;
int stack [M] , top = 0 , index = 0;
bool instack [M] ;
int dfn[M] , low[M] ;
int cnt = 0 ;
vector <int> e[M] ;
int belong[M] ;
int out[M] ;

void init (int n)
{
    top = 0 ;
    cnt = 0 ;
    index = 0 ;
    memset (stack , -1 , sizeof(stack)) ;
    memset (instack , 0 , sizeof(instack)) ;
    memset (dfn , -1 , sizeof(dfn)) ;
    memset (low , -1 , sizeof(low)) ;
    for (int i = 0 ; i <= n ; i++)
        e[i].clear () ;
    memset (belong , -1 , sizeof(belong)) ;
    memset (out , 0 , sizeof(out)) ;
}

void tarjan (int u)
{
    int v ;
    dfn[u] = low[u] = index++ ;
    instack[u] = true ;
    stack[++top] = u ;
    for (int i = 0 ; i < e[u].size () ; i++) {
        v = e[u][i] ;
        if (dfn[v] == -1) {
            tarjan (v) ;
            low[u] = min (low[u] , low[v]) ;
        }
        else if (instack[v])
            low[u] = min (low[u] , dfn[v]) ;
    }
    if (low[u] == dfn[u]) {
        cnt++ ;
        do {
            v = stack[top--] ;
            instack[v] = false ;
            belong[v] = cnt ;
        } while (u != v) ;
    }
}

int main ()
{
   //freopen ("a.txt" , "r" , stdin) ;
    int u , v ;
    while (~ scanf ("%d" ,&n)) {
        if (n == 0)
            break ;
        init (n) ;
        scanf ("%d" , &m) ;
        while (m--) {
            scanf ("%d%d" , &u , &v) ;
            e[u].push_back (v) ;
        }
        for (int i = 1 ; i <= n ; i++) {
            if (dfn[i] == -1)
                tarjan (i) ;
        }
        for (int i = 1 ; i <= n ; i++) {
            for (int j = 0 ; j < e[i].size () ; j++) {
                if (belong [i] != belong[e[i][j]])
                    out[belong[i]] ++;
            }
        }
        int k = 0 ;
        for (int i = 1 ; i <= n ; i++) {
            if (out[belong[i]] == 0) {
                if (k++)
                    printf (" ") ;
                printf ("%d" , i) ;
            }
        }
        puts ("") ;
    }
    return 0 ;
}