Weekly Contest 131

1021. Remove Outermost Parentheses

A valid parentheses string is either empty (""), "(" + A + ")", or A + B, where A and B are valid parentheses strings, and +represents string concatenation.  For example, "", "()", "(())()", and "(()(()))" are all valid parentheses strings.

A valid parentheses string S is primitive if it is nonempty, and there does not exist a way to split it into S = A+B, with A and Bnonempty valid parentheses strings.

Given a valid parentheses string S, consider its primitive decomposition: S = P_1 + P_2 + ... + P_k, where P_i are primitive valid parentheses strings.

Return S after removing the outermost parentheses of every primitive string in the primitive decomposition of S.

Example 1:

Input: "(()())(())"
Output: "()()()"
Explanation: 
The input string is "(()())(())", with primitive decomposition "(()())" + "(())".
After removing outer parentheses of each part, this is "()()" + "()" = "()()()".

Example 2:

Input: "(()())(())(()(()))"
Output: "()()()()(())"
Explanation: 
The input string is "(()())(())(()(()))", with primitive decomposition "(()())" + "(())" + "(()(()))".
After removing outer parentheses of each part, this is "()()" + "()" + "()(())" = "()()()()(())".

Example 3:

Input: "()()"
Output: ""
Explanation: 
The input string is "()()", with primitive decomposition "()" + "()".
After removing outer parentheses of each part, this is "" + "" = "".

Note:

1. S.length <= 10000
2. S[i] is "(" or ")"
3. S is a valid parentheses string

Approach #1: Stack. [C++]

class Solution {
public:
    string removeOuterParentheses(string S) {
        stack<char> sta;
        int left = 0, right = 0;
        string ans = "";
        for (int i = 0; i < S.length(); ++i) {
            if (sta.empty()) {
                right = i - 1;
                if (right - left - 1 >= 2) {
                    string s = S.substr(left+1, right-left-1);
                    ans += s;
                }
                left = i;
                sta.push(S[i]);
            } else {
                if (sta.top() == '(' && S[i] == ')') {
                    sta.pop();
                } else {
                    sta.push(S[i]);
                }
            }
        }
        string s = S.substr(left+1, S.length()-left-2);
        ans += s;
        
        return ans;
    }
};

　　

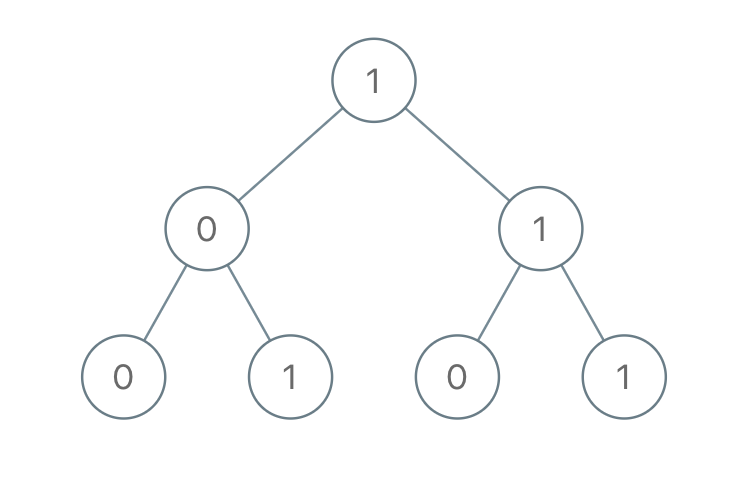
1022. Sum of Root To Leaf Binary Numbers

Given a binary tree, each node has value 0 or 1.  Each root-to-leaf path represents a binary number starting with the most significant bit.  For example, if the path is 0 -> 1 -> 1 -> 0 -> 1, then this could represent 01101 in binary, which is 13.

For all leaves in the tree, consider the numbers represented by the path from the root to that leaf.

Return the sum of these numbers.

Example 1:

Input: [1,0,1,0,1,0,1]
Output: 22
Explanation: (100) + (101) + (110) + (111) = 4 + 5 + 6 + 7 = 22

Note:

1. The number of nodes in the tree is between 1 and 1000.
2. node.val is 0 or 1.
3. The answer will not exceed 2^31 - 1.

Approach #1: [C++]

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode(int x) : val(x), left(NULL), right(NULL) {}
 * };
 */
class Solution {
public:
    int sumRootToLeaf(TreeNode* root, int val = 0) {
        const static int mod = 1000000007;
        if (!root) return 0;
        val = val * 2 + root->val;
        return (root->left == root->right ? val : sumRootToLeaf(root->left, val) + sumRootToLeaf(root->right, val)) % mod;
    }
};

　　

1023. Camelcase Matching

A query word matches a given pattern if we can insert lowercase letters to the pattern word so that it equals the query. (We may insert each character at any position, and may insert 0 characters.)

Given a list of queries, and a pattern, return an answer list of booleans, where answer[i] is true if and only if queries[i] matches the pattern.

Example 1:

Input: queries = ["FooBar","FooBarTest","FootBall","FrameBuffer","ForceFeedBack"], pattern = "FB"
Output: [true,false,true,true,false]
Explanation: 
"FooBar" can be generated like this "F" + "oo" + "B" + "ar".
"FootBall" can be generated like this "F" + "oot" + "B" + "all".
"FrameBuffer" can be generated like this "F" + "rame" + "B" + "uffer".

Example 2:

Input: queries = ["FooBar","FooBarTest","FootBall","FrameBuffer","ForceFeedBack"], pattern = "FoBa"
Output: [true,false,true,false,false]
Explanation: 
"FooBar" can be generated like this "Fo" + "o" + "Ba" + "r".
"FootBall" can be generated like this "Fo" + "ot" + "Ba" + "ll".

Example 3:

Input: queries = ["FooBar","FooBarTest","FootBall","FrameBuffer","ForceFeedBack"], pattern = "FoBaT"
Output: [false,true,false,false,false]
Explanation: 
"FooBarTest" can be generated like this "Fo" + "o" + "Ba" + "r" + "T" + "est".

Note:

1. 1 <= queries.length <= 100
2. 1 <= queries[i].length <= 100
3. 1 <= pattern.length <= 100
4. All strings consists only of lower and upper case English letters.

Approach #1: [C++]

class Solution {
public:
    vector<bool> camelMatch(vector<string>& queries, string pattern) {
        vector<bool> res;
        for (int i = 0; i < queries.size(); ++i) {
            res.push_back(judge(queries[i], pattern));
        }
        return res;
    }
    
private:
    bool judge(string str, string pattern) {
        int pnt = 0;
        for (int i = 0; i < str.length(); ++i) {
            if (str[i] == pattern[pnt]) pnt++;
            else if (islower(str[i])) continue;
            else return false;
        }
        return pnt == pattern.length();
    }
};

　　

1024. Video Stitching

You are given a series of video clips from a sporting event that lasted T seconds.  These video clips can be overlapping with each other and have varied lengths.

Each video clip clips[i] is an interval: it starts at time clips[i][0] and ends at time clips[i][1].  We can cut these clips into segments freely: for example, a clip [0, 7] can be cut into segments [0, 1] + [1, 3] + [3, 7].

Return the minimum number of clips needed so that we can cut the clips into segments that cover the entire sporting event ([0, T]).  If the task is impossible, return -1.

Example 1:

Input: clips = [[0,2],[4,6],[8,10],[1,9],[1,5],[5,9]], T = 10
Output: 3
Explanation: 
We take the clips [0,2], [8,10], [1,9]; a total of 3 clips.
Then, we can reconstruct the sporting event as follows:
We cut [1,9] into segments [1,2] + [2,8] + [8,9].
Now we have segments [0,2] + [2,8] + [8,10] which cover the sporting event [0, 10].

Example 2:

Input: clips = [[0,1],[1,2]], T = 5
Output: -1
Explanation: 
We can't cover [0,5] with only [0,1] and [0,2].

Example 3:

Input: clips = [[0,1],[6,8],[0,2],[5,6],[0,4],[0,3],[6,7],[1,3],[4,7],[1,4],[2,5],[2,6],[3,4],[4,5],[5,7],[6,9]], T = 9
Output: 3
Explanation: 
We can take clips [0,4], [4,7], and [6,9].

Example 4:

Input: clips = [[0,4],[2,8]], T = 5
Output: 2
Explanation: 
Notice you can have extra video after the event ends.

Note:

1. 1 <= clips.length <= 100
2. 0 <= clips[i][0], clips[i][1] <= 100
3. 0 <= T <= 100

Approach #1: [C++]

class Solution {
public:
    int videoStitching(vector<vector<int>>& clips, int T) {
        int n = clips.size();
        sort(clips.begin(), clips.end(), [](vector<int>& u, vector<int>& v) {
            return u[1] < v[1];
        });
        vector<int> dp(n+1, 0);
        int ret = INF;
        for (int i = 0; i < n; ++i) {
            dp[i] = (clips[i][0] == 0) ? 1 : INF;
            for (int j = 0; j < i; ++j) {
                if (clips[j][1] >= clips[i][0]) {
                    dp[i] = min(dp[j] + 1, dp[i]);
                }
            }
        }
        for (int i = 0; i < n; ++i) {
            if (clips[i][1] >= T) 
                ret = min(dp[i], ret);
        }
        
        return (ret == INF) ? -1 : ret;
    }
    
private:
    const int INF = 1 << 30;
};