cf div2 236 D

D. Upgrading Array

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

You have an array of positive integers a[1], a[2], ..., a[n] and a set of bad prime numbers b1, b2, ..., bm. The prime numbers that do not occur in the set b are considered good. The beauty of array a is the sum 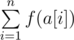, where function f(s) is determined as follows:

• f(1) = 0;
• Let's assume that p is the minimum prime divisor of s. If p is a good prime, then 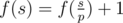, otherwise .

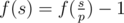You are allowed to perform an arbitrary (probably zero) number of operations to improve array a. The operation of improvement is the following sequence of actions:

• Choose some number r (1 ≤ r ≤ n) and calculate the value g = GCD(a[1], a[2], ..., a[r]).
• Apply the assignments: , , ..., .

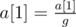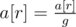What is the maximum beauty of the array you can get?

Input

The first line contains two integers n and m (1 ≤ n, m ≤ 5000) showing how many numbers are in the array and how many bad prime numbers there are.

The second line contains n space-separated integers a[1], a[2], ..., a[n] (1 ≤ a[i] ≤ 109) — array a. The third line contains m space-separated integers b1, b2, ..., bm (2 ≤ b1 < b2 < ... < bm ≤ 109) — the set of bad prime numbers.

Output

Print a single integer — the answer to the problem.

Sample test(s)

input

5 2
4 20 34 10 10
2 5

output

-2

input

4 5
2 4 8 16
3 5 7 11 17

output

10

贪心，从右往左扫gcd，若遇到好的质因子少于坏的质因子则可以更新使答案增加

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <cmath>
#include <set>

using namespace std;

#define maxn 40005
#define INF (1 << 30)

bool prime[maxn];
int ele[maxn],a[5005],g[5005],b[5005];
int len = 0,n,m,ans = 0;

int gcd(int x,int y) {
        return y ? gcd(y,x % y) : x;
}

int cal(int x) {
        int sum = 0;
        for(int i = 2; i * i <= x; ++i) {
                if(x % i == 0) {
                        int v,pos;
                        pos = lower_bound(b + 1,b + m + 1,i) - b;
                        v = b[pos] == i ? -1 : 1;
                        while(x % i == 0) {
                                sum += v;
                                x /= i;
                        }
                }
        }

        if(x != 1) {
                int pos = lower_bound(b + 1,b + m + 1,x) - b;
                sum += b[pos] == x ? -1 : 1;
        }
        return sum;
}

void solve() {
        //printf("cal = %d\n",cal(4));
        for(int i = 1; i <= n; ++i) {
                ans += cal(a[i]);
        }

        g[1] = a[1];
        for(int i = 2; i <= n; ++i) {
                g[i] = gcd(g[i - 1],a[i]);
        }

        int t = 1;
        for(int i = n; i >= 1; --i) {
                int v;
                if((v = cal(g[i] / t)) < 0) {
                        //printf("g = %d v = %d\n",g[i],v);
                        ans += (-v) * i;
                        t *= g[i] / t;
                }
        }


        printf("%d\n",ans);
}
int main() {

        //freopen("sw.in","r",stdin);

        scanf("%d%d",&n,&m);
        for(int i = 1; i <= n; ++i) scanf("%d",&a[i]);
        for(int i = 1; i <= m; ++i) {
               scanf("%d",&b[i]);
        }
        b[m + 1] = INF;

        solve();
        return 0;
}