正文
| 20182311 2019-2020-1 《数据结构与面向对象程序设计》第9周学习总结 |
教材学习内容总结
教材第15章
- 树的概述
- 定义:树是一种非线性结果,元素按分层组织。它含有结点和连接结点的边,位于树最高层的是根节点root,结点相对于根节点的位置表示结点的层。树中不含有子节点的结点为叶结点,除叶结点和根结点外的其他结点都是内节点。
- 树的阶:结点含有的最大子结点数。
- 路径长度:连接两个结点边的数量。高度:从根结点到叶结点的最长路径的长度。
- n元树:任一结点的子节点数目不多于n的
- 平衡:所有叶结点都位于同一层
- 完全:树平衡,且最底层的叶结点都在树的左边
- 树的实现:
- 数组实现策略:使用数组实现树,数组下标为n的结点的左子树下标为2n,右子树下标为2n+1。思路简单,访问迅速,但是该数组会为不含数据的树位置分配空间,浪费存储空间。
- 模拟连接策略:在数组中存入孩子的数组索引,为了节省空间会增加删除树中元素时,数组元素进行移位的成本。
- 链表实现:链表是最常见的造树方法,二叉链表可连接左右节点,三叉链表可指向父节点,多叉链表可以指向更上一级或者siblings
- 树的遍历:递归遍历比非递归遍历更简单,参照一个顺序的遍历可以写出其他顺序的遍历。
- 前序遍历:先根节点,后左子树,再右子树
- 中序遍历:先左子树,后根节点,再右子树
- 后序遍历:先左子树,后右子树,再根节点
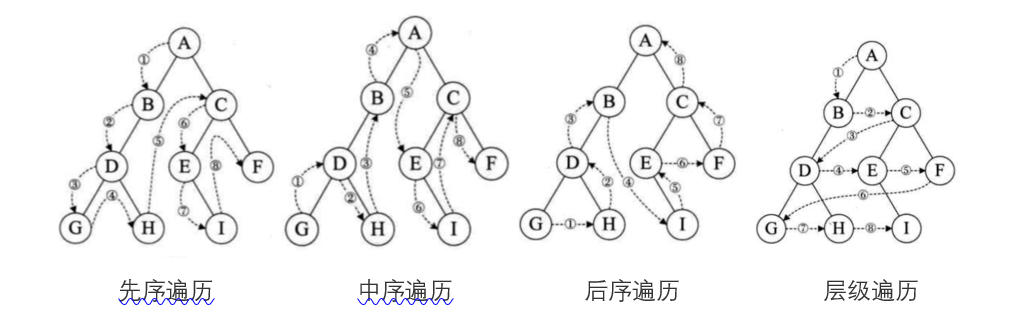
- 非递归遍历示例(先序):
public void preorderTraverse(){
//非递归实现先序遍历
Node t=root;
Node top;
Stack<Node> stack=new Stack<Node>();
while(t!=null||!stack.isEmpty()){
while(t!=null){
System.out.print(t.getData()+" ");
stack.push(t);
t=t.getLeft();
}
top=(Node)stack.peek();
stack.pop();
t=top.getRight();
}
}
- 递归遍历示例(先序):
public static void preOrderRe(Node t)
{//递归实现前序遍历
System.out.print(t.getData()+" ");
Node leftTree = t.getLeft();
if(leftTree != null){
preOrderRe(leftTree);
}
Node rightTree = t.getRight();
if(rightTree != null){
preOrderRe(rightTree);
}
}
- 非递归遍历示例(中序):
public void midorderTraverse(){
//非递归实现中序遍历
Node t=root;
Stack<Node> stack=new Stack<Node>();
while(t!=null||!stack.isEmpty()){
while(t!=null){
stack.push(t);
t=t.getLeft();
}
t=(Node)stack.peek();
stack.pop();
System.out.print(t.getData()+" ");
t=t.getRight();
}
}
- 递归遍历示例(中序):
public static void midOrderRe(Node t)
{//递归实现中序遍历
Node leftTree = t.getLeft();
if(leftTree != null){
midOrderRe(leftTree);
}
System.out.print(t.getData()+" ");
Node rightTree = t.getRight();
if(rightTree != null){
midOrderRe(rightTree);
}
}
- 非递归遍历示例(后序):
public void lasorderTraverse(){
//非递归实现后序遍历
Node t=root;
Node top,last=null;
Stack<Node> stack=new Stack<Node>();
while(t!=null||!stack.isEmpty()){
while(t!=null){
stack.push(t);
t=t.getLeft();
}
top=(Node)stack.peek();
if(top.getRight()==null||top.getRight()==last){
stack.pop();
System.out.print(top.getData()+" ");
last=top;
}
else{
t=top.getRight();
}
}
}
- 递归遍历示例(后序):
public static void lasOrderRe(Node t)
{//递归实现后序遍历
Node leftTree = t.getLeft();
if(leftTree != null){
lasOrderRe(leftTree);
}
Node rightTree = t.getRight();
if(rightTree != null){
lasOrderRe(rightTree);
}
System.out.print(t.getData()+" ");
}
- 层序遍历:
public void seqTraverse(Node t){
//利用队列先进先出实现层级遍历
Queue<Node> queue=new LinkedList<Node>();
queue.add(t);
while(!queue.isEmpty()){
Node q=queue.peek();
queue.remove();
if(q.getLeft()!=null){
queue.add(q.getLeft());
}
if(q.getRight()!=null){
queue.add(q.getRight());
}
System.out.print(q.getData()+" ");
}
}
- 决策树:基于链树实现,将决策信息填入结点中,定义一个访问方法,设置判定条件为输入n即访问左子树,输入y即访问右子树。
教材第16章
- 二叉查找树的概述:
- 定义:二叉查找树在二叉树的基础上规定了,左孩子小于父节点,父节点又小于等于右孩子。
- 具体过程:

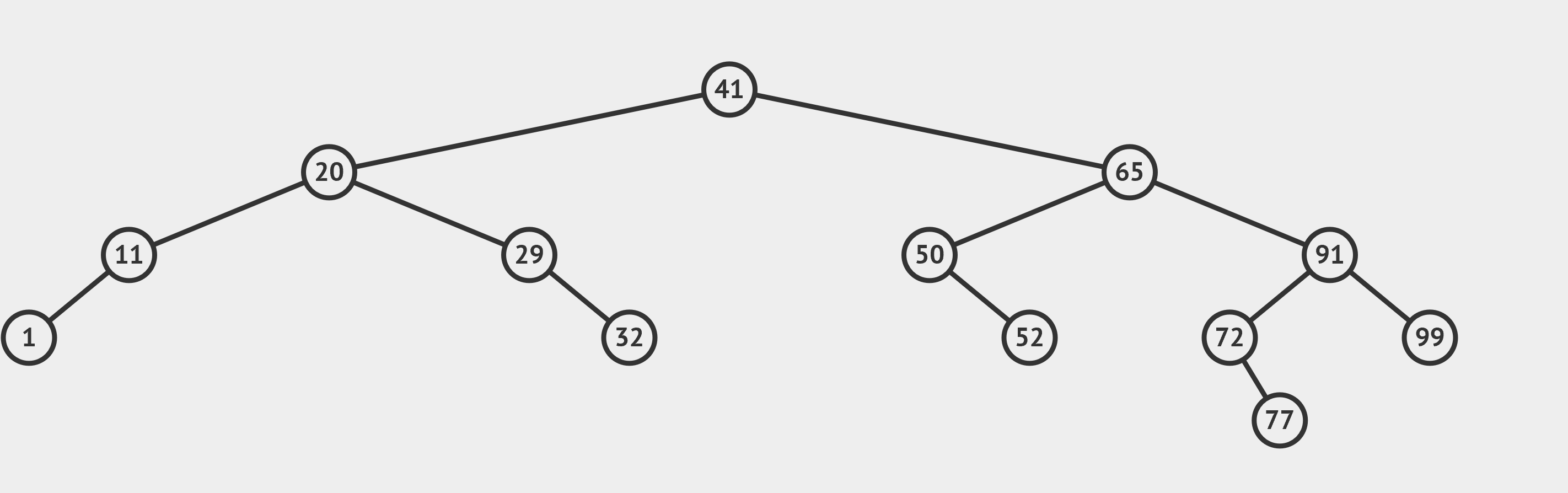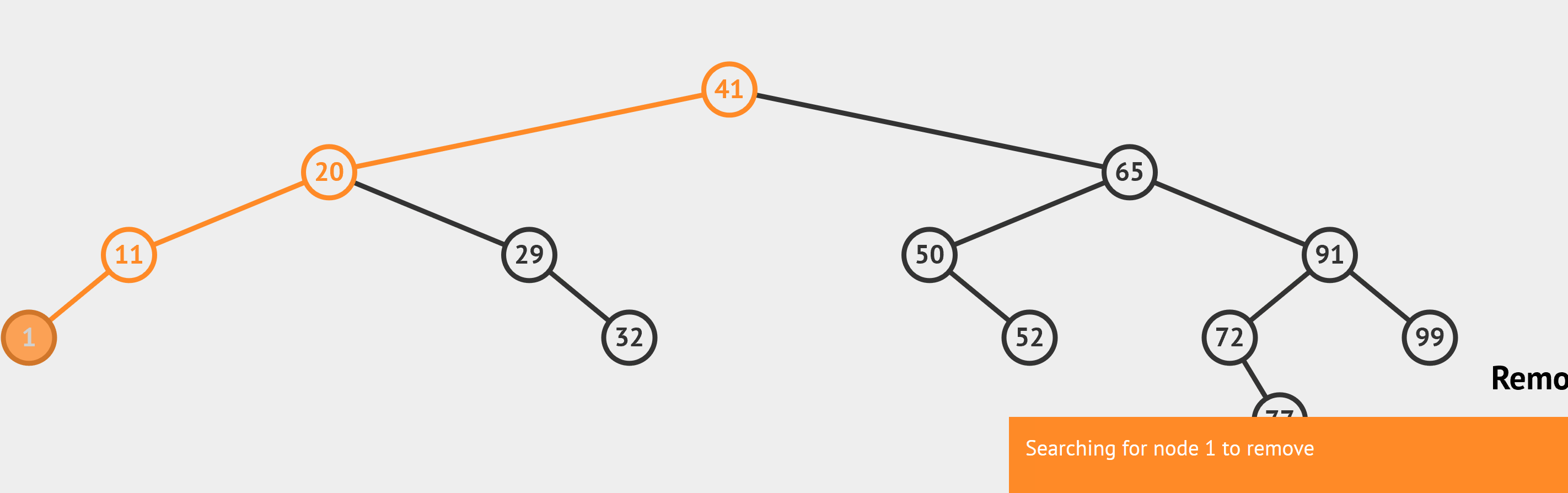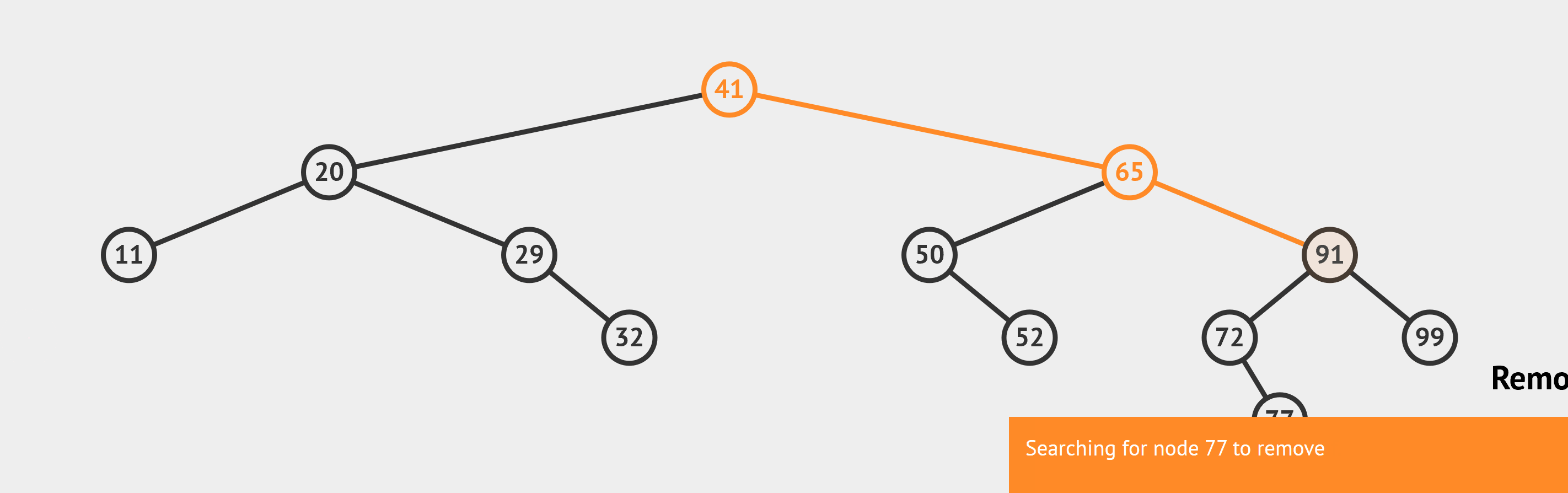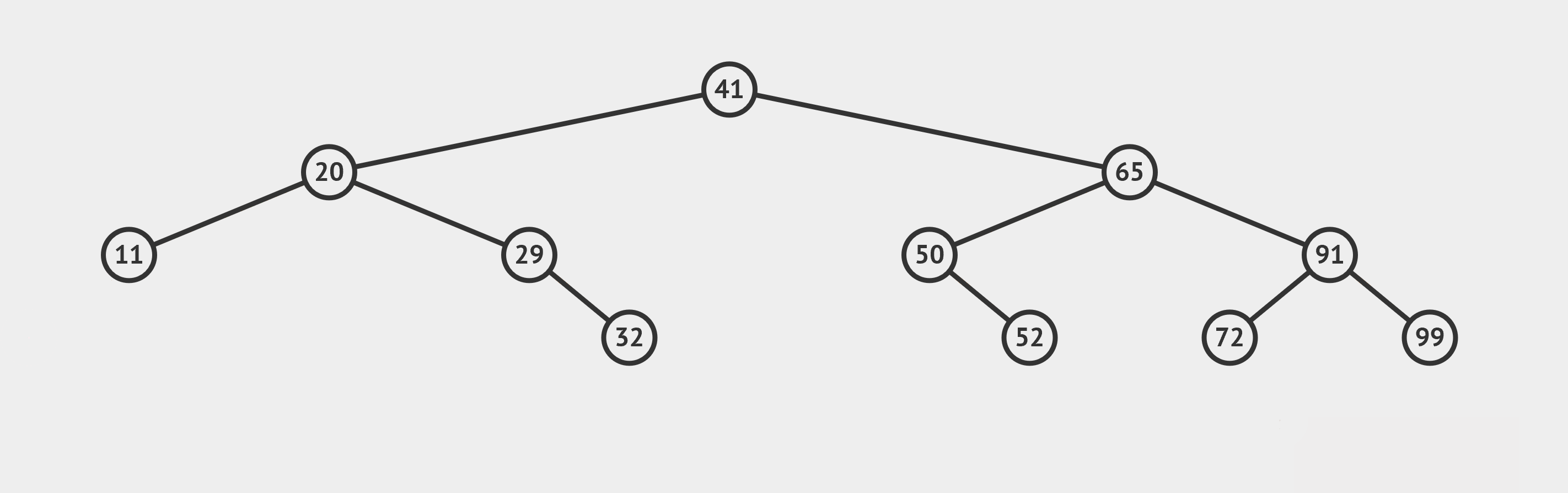
- 一些方法:addElement、removeElement(不能简单的删除节点的相关引用指针,而是要寻找替代点),replacement(寻找替代点的方法,若结点没有孩子,返回空;若结点只有一个孩子,返回这个孩子;若结点有两个孩子,返回他的后继/前继结点),后继就是按顺序排列后他的后面一位,前继就是按顺序排列后他的前面一位。这两个结点一定没有孩子,否则就不是前继/后继结点(分别位于左子树的最右边和右子树的最左边)
- 一些异常:树中元素不是Comparable,addElemnt会抛出NoComparableElementException异常。
教材学习中的问题和解决过程
- 问题1:怎样实现从子结点返回到根结点?或者说建立虚空结点树的时候,遇到#返回null以后怎么回到根节点重新赋值?
- 问题1解决方案:尝试了两种方法,一种是建立三叉链表,能实现就是有点麻烦。递归的思想其实就包括返回上一级,所以直接使用递归就能实现。
代码调试中的问题和解决过程
-
问题1:返回空指针异常和0>1错误
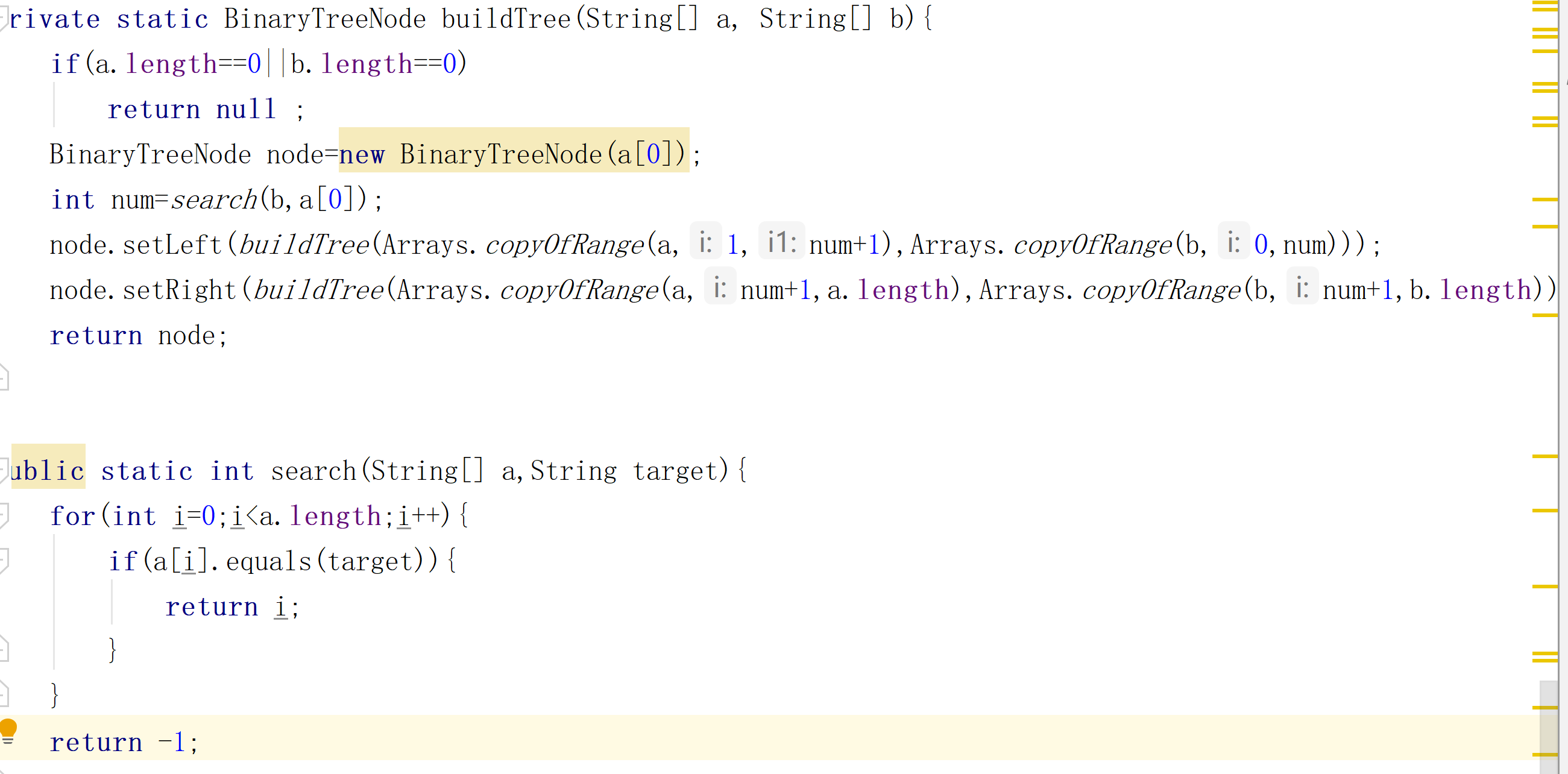
-
问题1解决方案:习惯规定search方法未找到元素返回-1,造成copyOf里面会出现(0,1)的情况。把未找到元素的返回值设置为1即可。
代码托管
上周错题总结
- One of the uses of trees is to provide simpler implementations of other collections.
A .True
B .Flase - 正确答案: B 我的答案: A
- To maintain the completeness of the tree, there is only one valid element to replace the root, and that is the element stored in the first leaf in the tree.
A .True
B .Flase - 正确答案: B 我的答案: A 。
- 用最后一个叶子节点替代。
- The removeMin operation for both the linked and array implementations is O(log n).
A.True
B .Flase - 正确答案: A 我的答案: B。
- 链表实现的需要遍历到最小值移除,数组实现的应该要考虑删除最小值后的移位。
- In a linked implementation of a stack, a pushed element should be added to the end of the list.
A .true
B .false - 正确答案:B 我的答案:A。
- 在堆栈的链接实现中,应该在列表的前面添加一个push元素。如果元素被添加到列表的末尾,那么pop操作将需要线性时间,因为我们需要遍历整个元素以获得指向列表中最后一个元素的指针。链接实现和数组实现有一定区别。
结对及互评
点评过的同学博客和代码
- 本周结对学习情况
学习进度条
| 代码行数(新增/累积) | 博客量(新增/累积) | 学习时间(新增/累积) | 重要成长 | |
|---|---|---|---|---|
| 目标 | 10000行 | 30篇 | 400小时 | |
| 第一周 | 246/246 | 2/2 | 30/30 | 初步掌握linux命令、java小程序和jdb调试 |
| 第二周 | 73/319 | 3/3 | 30/60 | |
| 第三周 | 906/1225 | 3/6 | 20/80 | |
| 第四周 | 748/1973 | 2/8 | 20/100 | |
| 第五周 | 849/2822 | 2/10 | 20/120 | |
| 第六周 | 962/ 3784 | 2/12 | 30/150 | |
| 第七周 | 1883/5668 | 3/15 | 50/200 | |
| 第八周 | 579/6247 | 1/16 | 30/230 | |
| 第九周 | 1195/7442 | 3/19 | 30/260 |