CodeForces 414B Mashmokh and ACM（dp）

B. Mashmokh and ACM

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

Mashmokh's boss, Bimokh, didn't like Mashmokh. So he fired him. Mashmokh decided to go to university and participate in ACM instead of finding a new job. He wants to become a member of Bamokh's team. In order to join he was given some programming tasks and one week to solve them. Mashmokh is not a very experienced programmer. Actually he is not a programmer at all. So he wasn't able to solve them. That's why he asked you to help him with these tasks. One of these tasks is the following.

A sequence of l integers b₁, b₂, ..., b_l (1 ≤ b₁ ≤ b₂ ≤ ... ≤ b_l ≤ n) is called good if each number divides (without a remainder) by the next number in the sequence. More formally 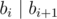for all i (1 ≤ i ≤ l - 1).

Given n and k find the number of good sequences of length k. As the answer can be rather large print it modulo 1000000007 (10⁹ + 7).

Input

The first line of input contains two space-separated integers n, k (1 ≤ n, k ≤ 2000).

Output

Output a single integer — the number of good sequences of length k modulo 1000000007 (10⁹ + 7).

Examples

Input

Copy

3 2

Output

Copy

5

Input

Copy

6 4

Output

Copy

39

Input

Copy

2 1

Output

Copy

2

Note

In the first sample the good sequences are: [1, 1], [2, 2], [3, 3], [1, 2], [1, 3].

题意:给你1~n之间的数，让你组成一个长度为k序列，使得后一个的数mod前一个数为0.

思路:后一个数只与前一个数有关，设dp[i][j]表示长度为i的序列中最后一个数字为j，则dp[i][l] = dp[i][l] + dp[i-1][j],其中l%j == 0，l是j的倍数；

#include <bits/stdc++.h>
#define int long long
#define rad rand()%mod+1
using namespace std;
const int maxn = 1e6+5;
const int maxm = 2e3+5;
const int mod = 1e9+7;
int dp[maxm][maxm];
signed main()
{
    int n, k;
    while(~scanf("%lld %lld", &n, &k))
    {
        memset(dp, 0, sizeof dp);
        for(int i=1; i<=n; i++)
            dp[1][i] = 1;
        for(int i=1; i<=k; i++)
        {
            for(int j=1; j<=n; j++)
            {
                for(int l=j; l<=n; l += j)
                    dp[i][l] = (dp[i][l] + dp[i-1][j]) % mod;
            }
        }
        int ans = 0;
        for(int i=1; i<=n; i++)
        {
            ans += dp[k][i];
            ans %= mod;
        }
        printf("%lld
", ans);
    }
    return 0;
}
/***


***/