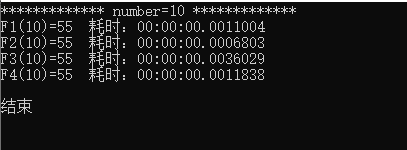
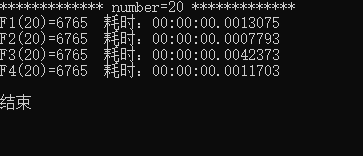
递归算法在实际应用中司空见惯,斐波那契数列是具体体现之一,递归算法是基本技能,今天是周六,再动一下大脑~
F1:迭代法,F2:直接法,F3矩阵法,F4:通项公式
using System; using System.Diagnostics; namespace Fibonacci { class Program { static void Main(string[] args) { ulong result; int number = 10; Console.WriteLine("************* number={0} *************", number); Stopwatch watch1 = new Stopwatch(); watch1.Start(); result = F1(number); watch1.Stop(); Console.WriteLine("F1({0})=" + result + " 耗时:" + watch1.Elapsed, number); Stopwatch watch2 = new Stopwatch(); watch2.Start(); result = F2(number); watch2.Stop(); Console.WriteLine("F2({0})=" + result + " 耗时:" + watch2.Elapsed, number); Stopwatch watch3 = new Stopwatch(); watch3.Start(); result = F3(number); watch3.Stop(); Console.WriteLine("F3({0})=" + result + " 耗时:" + watch3.Elapsed, number); Stopwatch watch4 = new Stopwatch(); watch4.Start(); double result4 = F4(number); watch4.Stop(); Console.WriteLine("F4({0})=" + result4 + " 耗时:" + watch4.Elapsed, number); Console.WriteLine(); Console.WriteLine("结束"); Console.ReadKey(); } /// <summary> /// 迭代法 /// </summary> /// <param name="number"></param> /// <returns></returns> private static ulong F1(int number) { if (number == 1 || number == 2) { return 1; } else { return F1(number - 1) + F1(number - 2); } } /// <summary> /// 直接法 /// </summary> /// <param name="number"></param> /// <returns></returns> private static ulong F2(int number) { ulong a = 1, b = 1; if (number == 1 || number == 2) { return 1; } else { for (int i = 3; i <= number; i++) { ulong c = a + b; b = a; a = c; } return a; } } /// <summary> /// 矩阵法 /// </summary> /// <param name="n"></param> /// <returns></returns> static ulong F3(int n) { ulong[,] a = new ulong[2, 2] { { 1, 1 }, { 1, 0 } }; ulong[,] b = MatirxPower(a, n); return b[1, 0]; } #region F3 static ulong[,] MatirxPower(ulong[,] a, int n) { if (n == 1) { return a; } else if (n == 2) { return MatirxMultiplication(a, a); } else if (n % 2 == 0) { ulong[,] temp = MatirxPower(a, n / 2); return MatirxMultiplication(temp, temp); } else { ulong[,] temp = MatirxPower(a, n / 2); return MatirxMultiplication(MatirxMultiplication(temp, temp), a); } } static ulong[,] MatirxMultiplication(ulong[,] a, ulong[,] b) { ulong[,] c = new ulong[2, 2]; for (int i = 0; i < 2; i++) { for (int j = 0; j < 2; j++) { for (int k = 0; k < 2; k++) { c[i, j] += a[i, k] * b[k, j]; } } } return c; } #endregion /// <summary> /// 通项公式法 /// </summary> /// <param name="n"></param> /// <returns></returns> static double F4(int n) { double sqrt5 = Math.Sqrt(5); return (1/sqrt5*(Math.Pow((1+sqrt5)/2,n)-Math.Pow((1-sqrt5)/2,n))); } } }