测地线又称为大地线,可以定义为空间曲面上两点的局部最短路径。测地线具有广泛的应用,例如在工业上测地线最短的性质就意味着最优最省,在航海和航空中,轮船和飞机的运行路线就是测地线。[Crane et al. 2013]提出了利用热运动方程来计算网格测地线的方法,可以想象一下,当一根烫的针尖接触到曲面上的一点时,热量会随着时间的推移而扩散,测地距离因此可以和热运动相联系。具体算法过程如下图所示:
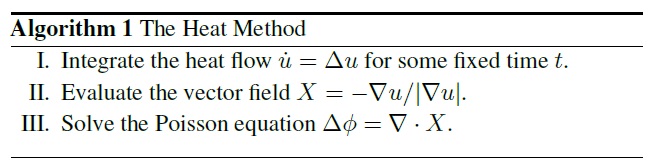
第一步:热运动方程用来描述热的传播状态:

将热运动方程离散化并整理后得到:
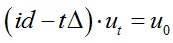
其中:id为单位矩阵,t为时间间隔,Δ为离散Laplacian算子,ut为t时刻的热状态,u0为初始时刻的热状态。
第二步:第一步计算得到的热梯度方向与测地距离的梯度方向相同,由Eikonal方程知道测地距离的梯度为单位向量,于是通过归一化热梯度我们得到测地距离的梯度:
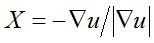
第三步:得到测地距离的梯度之后,测地线问题即变为求解以下式子:

根据变分法,上式最小化即求解泊松方程:
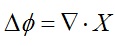
其中:Φ即为网格上顶点距离源点的测地距离。
function [D] = geodesics_in_heat(V, F, src) % choose time step c = 5; t = c * mean(doublearea(V, F))/2; %% Step 1: Integrate the heat flow for some fixed time t L = cotmatrix(V, F); M = massmatrix(V, F, 'barycentric'); nV = size(V, 1); u0 = zeros(nV, 1); u0(src) = 1; A = M - t*L; B = M*u0; nsrc = length(src); % 1.1 dirichlet condition hole = Cal_Boundary(F); if isempty(hole) boundary = []; else boundary = hole.boundary.edge(:,1)'; end b = setdiff(boundary, src); nb = [b, src]; Acons = sparse([1:length(nb)], nb, ones(1,length(nb)), length(nb), nV); Bcons = [zeros(length(b), 1); ones(nsrc, 1)]; % 硬约束 r = setdiff([1:nV], nb); uD = [A(r,:);Acons][B(r,:);Bcons]; % 1.2 neumann condition Acons = sparse([1:nsrc], src, ones(1,nsrc), nsrc, nV); Bcons = ones(nsrc, 1); % 硬约束 r = setdiff([1:nV], src); uN = [A(r,:);Acons][B(r,:);Bcons]; % averaged boundary condition u = 0.5*(uN + uD); %% Step 2: Evaluate the vector field X G = grad(V, F); % nF*3 by nV matrix(梯度算子 - 所有三角片顶点基函数) grad_u = reshape(G*u, size(F,1), 3); % nF by nV matrix(所有三角片中u的梯度) grad_u_norm = sqrt(sum(grad_u.^2, 2)); normalized_grad_u = bsxfun(@rdivide, grad_u, grad_u_norm+eps); X = -normalized_grad_u; %% Step 3: Solve the Poisson equation Div = div(V, F); % 散度算子 div_X = Div*X(:); % #nV by #nF*3 Lcons = sparse([1:nsrc], src, ones(1,nsrc), nsrc, nV); div_Xcons = zeros(nsrc, 1); % 硬约束 r = setdiff([1:nV], src); D = [L(r,:);Lcons][div_X(r,:);div_Xcons]; D = D - min(D); end
效果: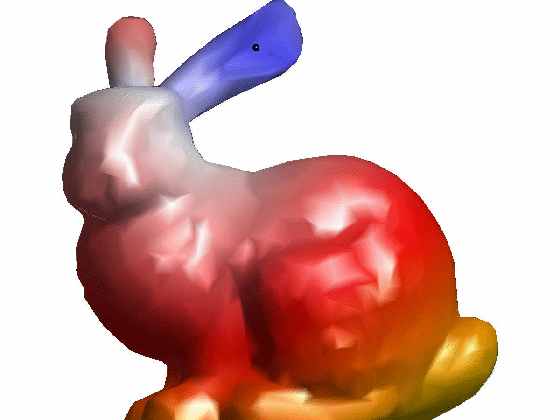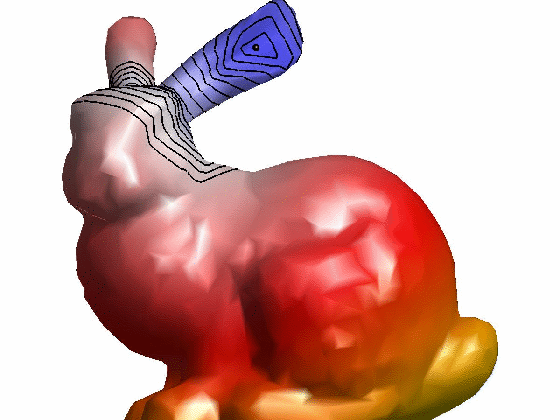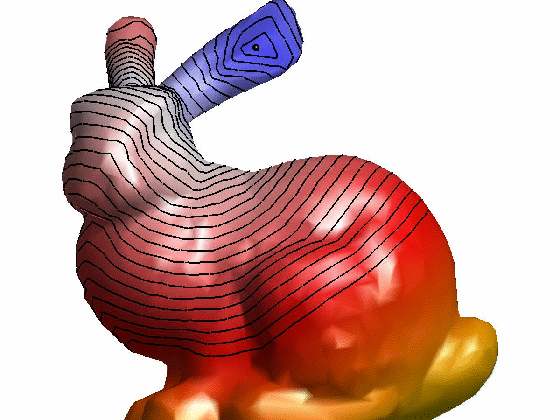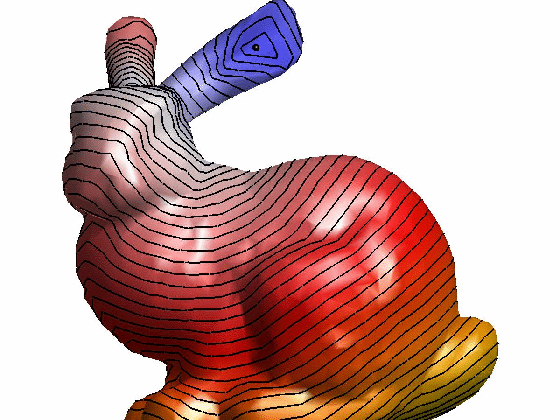


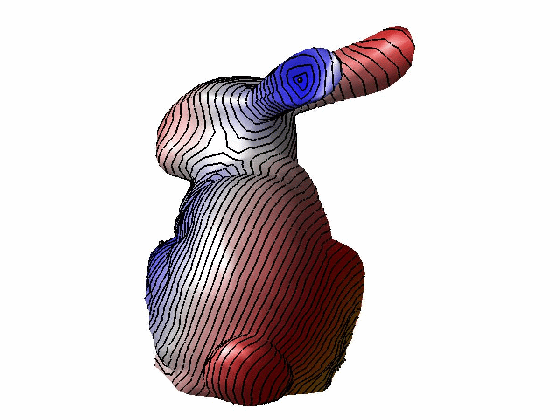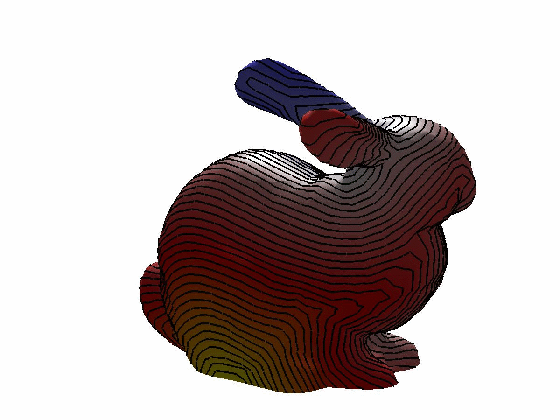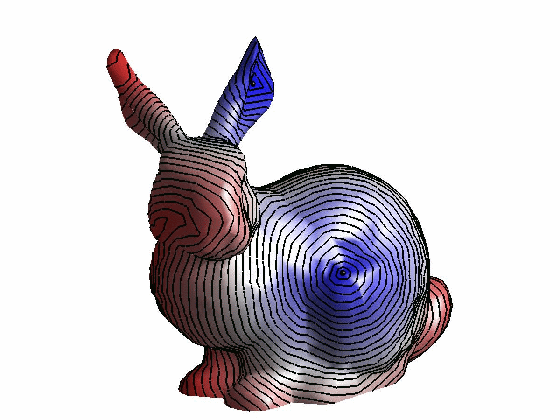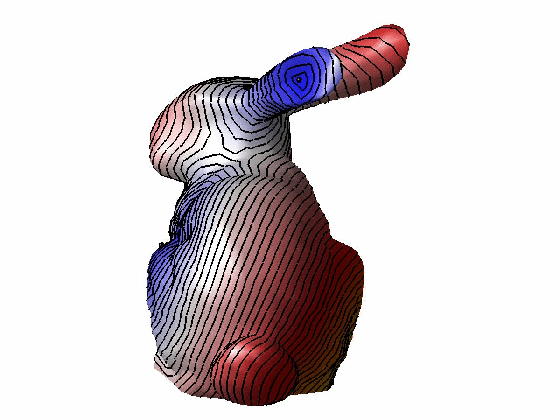
本文为原创,转载请注明出处:http://www.cnblogs.com/shushen。
参考文献:
[1] Keenan Crane, Clarisse Weischedel, and Max Wardetzky. 2013. Geodesics in heat: A new approach to computing distance based on heat flow. ACM Trans. Graph. 32, 5, Article 152 (October 2013), 11 pages.