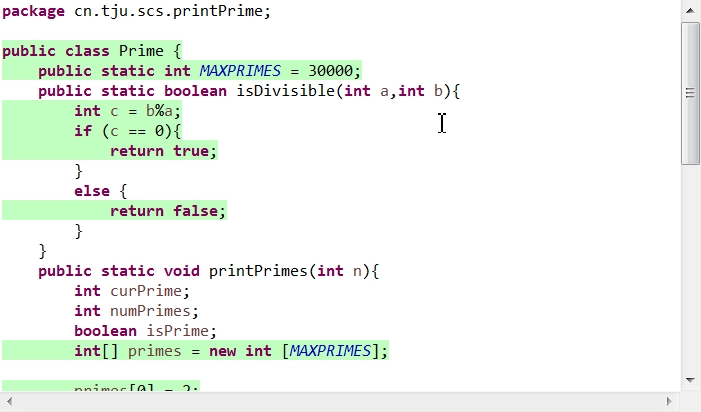
题目的源代码如下:
1 package cn.tju.scs.printPrime; 2 3 public class Prime { 4 public static int MAXPRIMES = 30000; 5 public static boolean isDivisible(int a,int b){ 6 int c = b%a; 7 if (c == 0){ 8 return true; 9 } 10 else { 11 return false; 12 } 13 } 14 public static void printPrimes(int n){ 15 int curPrime; 16 int numPrimes; 17 boolean isPrime; 18 int[] primes = new int [MAXPRIMES]; 19 20 primes[0] = 2; 21 numPrimes = 1; 22 curPrime = 2; 23 while(numPrimes < n){ 24 curPrime++; 25 isPrime = true; 26 for(int i = 0; i <= numPrimes - 1; i++){ 27 if(isDivisible(primes[i],curPrime)){ 28 isPrime = false; 29 break; 30 } 31 } 32 if(isPrime){ 33 primes[numPrimes] = curPrime; 34 numPrimes++; 35 } 36 } 37 38 for(int i = 0; i <= numPrimes -1; i++){ 39 System.out.println("Primes: " + primes[i]); 40 } 41 42 } 43 44 45 46 }
问题:
1、以上源代码的控制流图如下:

2、当把下面判断是不是素数的代码中的for的条件改成for(int i = 0; i <= 0 ; i++)是代码如下
for(int i = 0; i <= 0; i++){
if(isDivisible(primes[i],curPrime)){
isPrime = false;
break;
}
}
这样每次只判断是不是偶数,当t1 = (n = 3)时,算出的素数为2、3、5所得到的结果并没有发现错误;然而当t2 = (n = 5)时,所得的结果为2、3、5、7、9,我们能够看出所得的结果是错误的,所以能够检查出错误。所以测试用例有力相同的主路径,并不一定找出相同的错误。
3、当 n= 1时,是相应的测试路径访问连接While语句开始到for语句的边,而不通过while的循环体。
4、
节点覆盖:TR{1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16}
边覆盖: TR{(1,2),(2,3),(3,5),(5,6),(6,7),(7,8),(7,9),(9,6),(6,10),(8,10),(10,11),(11,12),(12,13),(11,13),(13,2),(2,4),(4,14),(14,15),(15,14),(14,16)}
主路径覆盖:TR:
[1,2,3,5,6,7,9]
[1,2,3,5,6,7,8,10,11,12,13]
[1,2,3,5,6,7,8,10,11,13]
[1,2,3,5,6,10,11,12,13]
[1,2,3,5,6,7,8,10,11,13]
[2,3,5,6,7,8,10,11,12,13,2]
[2,3,5,6,7,8,10,11,13,2]
[2,3,5,6,10,11,12,13,2]
[2,3,5,6,7,8,10,11,13,2]
[1,2,4,14,15]
[1,2,4,14,16]
[6,7,9,6]
[14,15,14]
[3,5,6,7,8,10,11,12,13,2,4,14,15]
[3,5,6,7,8,10,11,13,2,4,14,15]
[3,5,6,10,11,13,2,4,14,15]
[3,5,6,10,11,12,13,2,4,14,15]
[7,9,6,7,8,10,11,12,13,2,4,14,15]
[7,9,6,7,8,10,11,13,2,4,14,15]
[7,9,6,10,11,13,2,4,14,15]
[7,9,6,10,11,12,13,2,4,14,15]
[3,5,6,7,8,10,11,12,13,2,4,14,16]
[3,5,6,7,8,10,11,13,2,4,14,16]
[3,5,6,10,11,13,2,4,14,16]
[3,5,6,10,11,12,13,2,4,14,16]
[7,9,6,7,8,10,11,12,13,2,4,14,16]
[7,9,6,7,8,10,11,13,2,4,14,16]
[7,9,6,10,11,13,2,4,14,16]
[7,9,6,10,11,12,13,2,4,14,16]
[15,14,16]
[2,3,5,6,7,8,10,11,12,13,2]
[3,5,6,7,8,10,11,12,13,2,3]
[5,6,7,8,10,11,12,13,2,3,5]
[6,7,8,10,11,12,13,2,3,5,6]
[7,8,10,11,12,13,2,3,5,6,7]
[8,10,11,12,13,2,3,5,6,7,8]
[10,11,12,13,2,3,5,6,7,8,10]
[11,12,13,2,3,5,6,7,8,10,11]
[12,13,2,3,5,6,7,8,10,11,12]
[13,2,3,5,6,7,8,10,11,12,13]
[2,3,5,6,7,8,10,11,13,2]
[3,5,6,7,8,10,11,13,2,3]
[5,6,7,8,10,11,13,2,3,5]
[6,7,8,10,11,13,2,3,5,6]
[7,8,10,11,13,2,3,5,6,7]
[8,10,11,13,2,3,5,6,7,8]
[10,11,13,2,3,5,6,7,8,10]
[11,13,2,3,5,6,7,8,10,11]
[13,2,3,5,6,7,8,10,11,13]
[2,3,5,6,10,11,12,13,2]
[3,5,6,10,11,12,13,2,3]
[5,6,10,11,12,13,2,3,5]
[6,10,11,12,13,2,3,5,6]
[10,11,12,13,2,3,5,6,10]
[11,12,13,2,3,5,6,10,11]
[12,13,2,3,5,6,10,11,12]
[13,2,3,5,6,10,11,12,13]
[2,3,5,6,7,8,10,11,13,2]
[3,5,6,7,8,10,11,13,2,3]
[5,6,7,8,10,11,13,2,3,5]
[6,7,8,10,11,13,2,3,5,6]
[7,8,10,11,13,2,3,5,6,7]
[8,10,11,13,2,3,5,6,7,8]
[10,11,13,2,3,5,6,7,8,10]
[11,13,2,3,5,6,7,8,10,11]
[13,2,3,5,6,7,8,10,11,13]
[6,7,9,6]
[7,9,6,7]
[9,6,7,9]
[14,15,14]
[15,14,15]
5、测试方法的实现

1 package cn.tju.scs.printPrime; 2 3 import static org.junit.Assert.*; 4 5 import org.junit.Before; 6 import org.junit.Test; 7 8 public class PrimeTest { 9 10 @Before 11 public void setUp() throws Exception { 12 } 13 14 @Test 15 public void test() { 16 Prime.printPrimes(3); 17 Prime.printPrimes(4); 18 Prime.printPrimes(1); 19 Prime p = new Prime(); 20 } 21 22 }
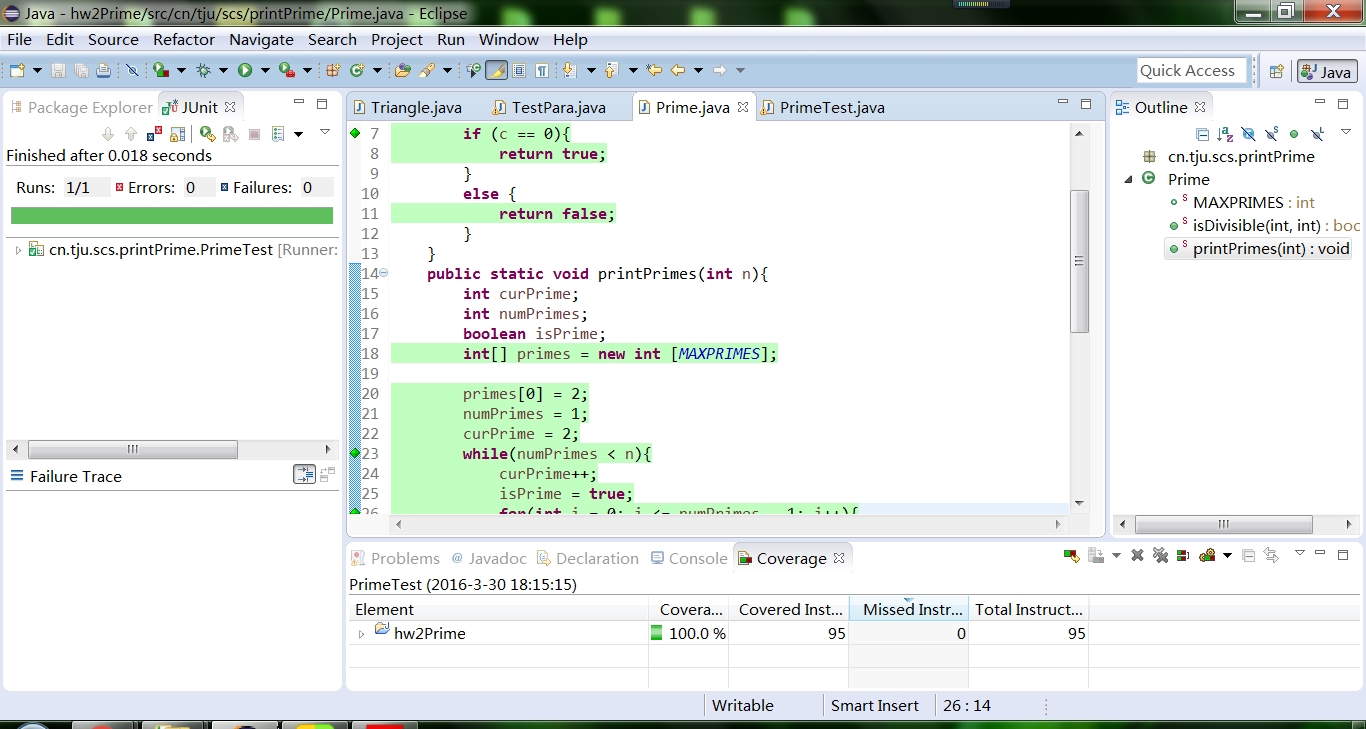
测试效果如下图所示: