lintcode ：最长公共子串

题目

最长公共子串

给出两个字符串，找到最长公共子串，并返回其长度。

样例

给出A=“ABCD”，B=“CBCE”，返回 2

注意

子串的字符应该连续的出现在原字符串中，这与子序列有所不同。

解题

注意：

子序列：这个序列不是在原字符串中连续的位置，而是有间隔的，如：ABCDE  和AMBMCMDMEM 最长公共子序列是ADCDE

子串：子串一定在原来字符串中连续存在的。如：ABCDEF 和SSSABCDOOOO最长公共子串是ABCD

参考链接，讲解很详细

根据子串定义，暴力破解

public class Solution {
    /**
     * @param A, B: Two string.
     * @return: the length of the longest common substring.
     */
    public int longestCommonSubstring(String A, String B) {
        // write your code here
        if(A == null || B == null ||A.length() ==0 || B.length() ==0)
            return 0;
        int lenA = A.length();
        int lenB = B.length();
        int longest = -1;
        
        for(int i=0 ; i <lenA ;i++){
            for(int j=0; j <lenB ;j++){
                int m = i;
                int n = j;
                int sublongest = 0;
                while(m<lenA && n < lenB){
                    char ch1 = A.charAt(m);
                    char ch2 = B.charAt(n);
                    if(ch1 == ch2){
                        m++;
                        n++;
                        sublongest += 1;
                    }else{
                        break;
                    }
                }
                longest = Math.max(longest,sublongest);
            }
        }
        return longest;
        
    }
}

时间复杂度O(N²M²)

该解法的思路就如前所说，以字符串中的每个字符作为子串的端点，判定以此为开始的子串的相同字符最长能达到的长度。其实从表层上想，这个算法的复杂度应该只有O(n2)因为该算法把每个字符都成对相互比较一遍，但关键问题在于比较两个字符串的效率并非是O(1)，这也导致了实际的时间复杂度应该是满足O(n2)和O(n3)。

上面博客中给了第一种动态规划的解法

定义数组C[lenA+1][lenB+1] C[i][j] 表示字符串A 以A[i-1] 结束 B以B[j-1] 最大相同子串的长度

当A[i-1] ==B[j-1] 的时候 C[i][j] = C[i-1][j-1] + 1 理解了子串的定义就很显然的，”连续字符串“

当A[i-1] !=B[j-1] 的时候  C[i][j] = 0 

数组中的最大值就是答案了

public class Solution {
    /**
     * @param A, B: Two string.
     * @return: the length of the longest common substring.
     */
    public int longestCommonSubstring(String A, String B) {
        // write your code here
        // String A = "cpoe.com code";
        // String B = "ccht.com code";
        if(A == null || B == null ||A.length() ==0 || B.length() ==0)
            return 0;
        int lenA = A.length();
        int lenB = B.length();
        int [][] C = new int[lenA + 1][lenB + 1];
        int longest = -1;
        for(int i=1;i<= lenA;i++){
            for(int j= 1;j<= lenB;j++){
                char ch1 = A.charAt(i-1);
                char ch2 = B.charAt(j-1);
                if( ch1 == ch2){
                    C[i][j] = C[i-1][j-1] + 1;
                }else{
                    C[i][j] = 0;
                }
                longest = Math.max(C[i][j],longest);
            }
        }
        
        return longest;
        
    }
}

在求最长公共子序列的题目中我考虑过利用数组进行求解，但是好的解法都没有直接用到我定义的那么简单的数组

对于这个题目，字符串数组表示为：相同字符是 1

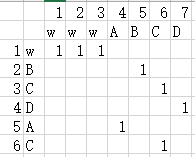
你一定会发现：子串一定在对角线上，连续对角线上1最多的那个就是最长的子串，子串的起始位置就是左上的第一个1，结束位置就是右下的最后一个一，暴力方法就是对每一个点开始的对角线1的个数，最大值就是答案，这个方法其实和上面的暴力方法一个意思的。

public class Solution {
    /**
     * @param A, B: Two string.
     * @return: the length of the longest common substring.
     */
    public int longestCommonSubstring(String A, String B) {
        // write your code here
        if(A == null || B == null ||A.length() ==0 || B.length() ==0)
            return 0;
        int lenA = A.length();
        int lenB = B.length();
        int [][] C = new int[lenA ][lenB ];
        int longest = -1;
        for(int i=0;i<lenA;i++){
            for(int j= 0;j< lenB;j++){
                char ch1 = A.charAt(i);
                char ch2 = B.charAt(j);
                if( ch1 == ch2){
                    C[i][j] = 1;
                }else{
                    C[i][j] = 0;
                }
               
            }
        }
        for(int i =0;i< lenA;i++){
            for(int j=0;j<lenB;j++){
                int m = i;
                int n = j;
                int sublongest = 0;
                while(m<lenA && n <lenB){
                    if(C[m][n] ==1){
                        sublongest +=1;
                        m++;
                        n++;
                    }else{
                        break;
                    }
                }
                longest = Math.max(longest,sublongest);
            }
        }
        
        return longest;
        
    }
}

上面的动态规划解法是在求出数组的同时求对角线上1的个数，上面的分开计算更容易理解，更容易想到动态规划的解法。

上面博客还要其他方法，待更新。。。