题目:
| Time Limit: 2000MS | Memory Limit: 65536K | |
| Total Submissions: 14437 | Accepted: 7001 |
Description
Calculate the number of toys that land in each bin of a partitioned toy box.
Mom and dad have a problem - their child John never puts his toys away when he is finished playing with them. They gave John a rectangular box to put his toys in, but John is rebellious and obeys his parents by simply throwing his toys into the box. All the toys get mixed up, and it is impossible for John to find his favorite toys.
John's parents came up with the following idea. They put cardboard partitions into the box. Even if John keeps throwing his toys into the box, at least toys that get thrown into different bins stay separated. The following diagram shows a top view of an example toy box.
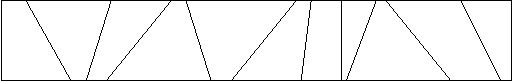
For this problem, you are asked to determine how many toys fall into each partition as John throws them into the toy box.
Mom and dad have a problem - their child John never puts his toys away when he is finished playing with them. They gave John a rectangular box to put his toys in, but John is rebellious and obeys his parents by simply throwing his toys into the box. All the toys get mixed up, and it is impossible for John to find his favorite toys.
John's parents came up with the following idea. They put cardboard partitions into the box. Even if John keeps throwing his toys into the box, at least toys that get thrown into different bins stay separated. The following diagram shows a top view of an example toy box.

For this problem, you are asked to determine how many toys fall into each partition as John throws them into the toy box.
Input
The input file contains one or more problems. The first line of a problem consists of six integers, n m x1 y1 x2 y2. The number of cardboard partitions is n (0 < n <= 5000) and the number of toys is m (0 < m <= 5000). The coordinates of the upper-left corner and the lower-right corner of the box are (x1,y1) and (x2,y2), respectively. The following n lines contain two integers per line, Ui Li, indicating that the ends of the i-th cardboard partition is at the coordinates (Ui,y1) and (Li,y2). You may assume that the cardboard partitions do not intersect each other and that they are specified in sorted order from left to right. The next m lines contain two integers per line, Xj Yj specifying where the j-th toy has landed in the box. The order of the toy locations is random. You may assume that no toy will land exactly on a cardboard partition or outside the boundary of the box. The input is terminated by a line consisting of a single 0.
Output
The output for each problem will be one line for each separate bin in the toy box. For each bin, print its bin number, followed by a colon and one space, followed by the number of toys thrown into that bin. Bins are numbered from 0 (the leftmost bin) to n (the rightmost bin). Separate the output of different problems by a single blank line.
Sample Input
5 6 0 10 60 0 3 1 4 3 6 8 10 10 15 30 1 5 2 1 2 8 5 5 40 10 7 9 4 10 0 10 100 0 20 20 40 40 60 60 80 80 5 10 15 10 25 10 35 10 45 10 55 10 65 10 75 10 85 10 95 10 0
Sample Output
0: 2 1: 1 2: 1 3: 1 4: 0 5: 1 0: 2 1: 2 2: 2 3: 2 4: 2
Hint
As the example illustrates, toys that fall on the boundary of the box are "in" the box.
思路:题目其实就是判断点在凸多边形内,直接用叉积二分判断即可。
代码:

1 #include<stdio.h> 2 /* 二维几何 */ 3 /* 需要包含的头文件 */ 4 #include <cmath > 5 6 /* 常用的常量定义 */ 7 const double INF = 1e200; 8 const double EP = 1e-10; 9 const double PI = acos(-1.0); 10 11 /* 基本几何结构 */ 12 struct Point 13 { 14 double x,y; 15 Point(double a=0, double b=0){x=a,y=b;} 16 friend Point operator+(const Point &ta,const Point &tb) 17 { 18 return Point(ta.x+tb.x,ta.y+tb.y); 19 } 20 friend Point operator-(const Point &ta,const Point &tb) 21 { 22 return Point(ta.x-tb.x,ta.y-tb.y); 23 } 24 }; 25 struct Vec2D//二维向量,*重载为点乘,/重载为叉乘 26 { 27 double x,y; 28 Vec2D(double ta,double tb){x=ta,y=tb;} 29 Vec2D(Point &ta){x=ta.x,y=ta.y;} 30 friend double operator*(const Vec2D &ta,const Vec2D &tb) 31 { 32 return ta.x*tb.x+ta.y*tb.y; 33 } 34 friend double operator/(const Vec2D &ta,const Vec2D &tb) 35 { 36 return ta.x*tb.y-ta.y*tb.x; 37 } 38 friend Vec2D operator+(const Vec2D &ta,const Vec2D &tb) 39 { 40 return Vec2D(ta.x+tb.x,ta.y+tb.y); 41 } 42 friend Vec2D operator-(const Vec2D &ta,const Vec2D &tb) 43 { 44 return Vec2D(ta.x-tb.x,ta.y-tb.y); 45 } 46 Vec2D operator=(const Vec2D &ta) 47 { 48 x=ta.x,y=ta.y; 49 return *this; 50 } 51 }; 52 struct LineSeg //线段,重载了/作为叉乘运算符,*作为点乘运算符 53 { 54 Point s,e; 55 LineSeg(){s=Point(0,0),e=Point(0,0);} 56 LineSeg(Point a, Point b){s=a,e=b;} 57 friend double operator*(const LineSeg &ta,const LineSeg &tb) 58 { 59 return (ta.e.x-ta.s.x)*(tb.e.x-tb.s.x)+(ta.e.y-ta.s.y)*(tb.e.y-tb.s.y); 60 } 61 friend double operator/(const LineSeg &ta,const LineSeg &tb) 62 { 63 return (ta.e.x-ta.s.x)*(tb.e.y-tb.s.y)-(ta.e.y-ta.s.y)*(tb.e.x-tb.s.x); 64 } 65 LineSeg operator=(const LineSeg &ta) 66 { 67 s=ta.s,e=ta.e; 68 return *this; 69 } 70 71 }; 72 struct Line // 直线的解析方程 a*x+b*y+c=0 为统一表示,约定 a >= 0 73 { 74 double a,b,c; 75 Line(double d1=1, double d2=-1, double d3=0){ a=d1,b=d2,c=d3;} 76 }; 77 78 LineSeg ls[6000]; 79 int n,m,x1,x2,y1,y2,num[6000]; 80 void sc(void) 81 { 82 LineSeg tmp; 83 tmp.e=Point(x1,y1); 84 int l=1,r=n+1,mid,ans; 85 while(l<=r) 86 { 87 mid=l+r>>1; 88 tmp.s=ls[mid].s; 89 if(ls[mid]/tmp>0) l=mid+1,ans=mid; 90 else r=mid-1; 91 } 92 num[ans]++; 93 } 94 int main(void) 95 { 96 while(scanf("%d%d%d%d%d%d",&n,&m,&x1,&y1,&x2,&y2)==6 && n) 97 { 98 ls[1]=LineSeg(Point(x1,y1),Point(x1,y2)); 99 ls[n+2]=LineSeg(Point(x2,y1),Point(x2,y2)); 100 for(int i=1;i<=n;i++) 101 scanf("%d%d",&x1,&x2),ls[i+1]=LineSeg(Point(x1,y1),Point(x2,y2)); 102 for(int i=1;i<=m;i++) 103 scanf("%d%d",&x1,&y1),sc(); 104 for(int i=1;i<=n+1;i++) 105 printf("%d: %d ",i-1,num[i]),num[i]=0; 106 printf(" "); 107 108 } 109 return 0; 110 }
