1376. Time Needed to Inform All Employees

A company has n employees with a unique ID for each employee from 0 to n - 1. The head of the company has is the one with headID.

Each employee has one direct manager given in the manager array where manager[i] is the direct manager of the i-th employee, manager[headID] = -1. Also it's guaranteed that the subordination relationships have a tree structure.

The head of the company wants to inform all the employees of the company of an urgent piece of news. He will inform his direct subordinates and they will inform their subordinates and so on until all employees know about the urgent news.

The i-th employee needs informTime[i] minutes to inform all of his direct subordinates (i.e After informTime[i] minutes, all his direct subordinates can start spreading the news).

Return the number of minutes needed to inform all the employees about the urgent news.

Example 1:

Input: n = 1, headID = 0, manager = [-1], informTime = [0]
Output: 0
Explanation: The head of the company is the only employee in the company.

Example 2:

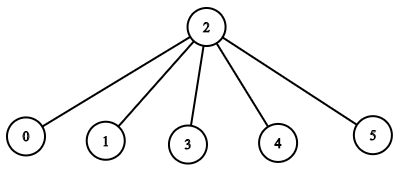
Input: n = 6, headID = 2, manager = [2,2,-1,2,2,2], informTime = [0,0,1,0,0,0]
Output: 1
Explanation: The head of the company with id = 2 is the direct manager of all the employees in the company and needs 1 minute to inform them all.
The tree structure of the employees in the company is shown.

Example 3:

Input: n = 7, headID = 6, manager = [1,2,3,4,5,6,-1], informTime = [0,6,5,4,3,2,1]
Output: 21
Explanation: The head has id = 6. He will inform employee with id = 5 in 1 minute.
The employee with id = 5 will inform the employee with id = 4 in 2 minutes.
The employee with id = 4 will inform the employee with id = 3 in 3 minutes.
The employee with id = 3 will inform the employee with id = 2 in 4 minutes.
The employee with id = 2 will inform the employee with id = 1 in 5 minutes.
The employee with id = 1 will inform the employee with id = 0 in 6 minutes.
Needed time = 1 + 2 + 3 + 4 + 5 + 6 = 21.

Example 4:

Input: n = 15, headID = 0, manager = [-1,0,0,1,1,2,2,3,3,4,4,5,5,6,6], informTime = [1,1,1,1,1,1,1,0,0,0,0,0,0,0,0]
Output: 3
Explanation: The first minute the head will inform employees 1 and 2.
The second minute they will inform employees 3, 4, 5 and 6.
The third minute they will inform the rest of employees.

Example 5:

Input: n = 4, headID = 2, manager = [3,3,-1,2], informTime = [0,0,162,914]
Output: 1076

Constraints:

• 1 <= n <= 10^5
• 0 <= headID < n
• manager.length == n
• 0 <= manager[i] < n
• manager[headID] == -1
• informTime.length == n
• 0 <= informTime[i] <= 1000
• informTime[i] == 0 if employee i has no subordinates.
• It is guaranteed that all the employees can be informed.

class Solution {
    public int numOfMinutes(int n, int headID, int[] manager, int[] informTime) {
        // 建图, sons[i] 表示节点 i 的子节点
        List<List<Integer>> sons = new ArrayList<>();
        for (int i = 0; i <= n; i++) {
            sons.add(new ArrayList<>());
        }
        // 依次添加每一条边
        for (int i = 0; i < n; i++) {
            if (manager[i] >= 0) {
                sons.get(manager[i]).add(i);
            }
        }
        // 一次遍历得到答案
        return maxLength(headID, sons, informTime);
    }

    private int maxLength(int cur, List<List<Integer>> sons, int[] informTime) {
        // 返回 cur 到叶子节点的最长路径
        int res = 0;
        for (int next : sons.get(cur)) {
            // cur 到 next 的边长为 informTime[cur]
            res = Math.max(maxLength(next, sons, informTime) + informTime[cur], res);
        }
        return res;
    }
}

DFS, 先建立起图，然后遍历每条能到叶子结点的路径，最大值就是answer