试题 算法训练 奇异的虫群 -> 矩阵快速幂

问题描述

　　在一个奇怪的星球上驻扎着两个虫群A和B，它们用奇怪的方式繁殖着，在t+1时刻A虫群的数量等于t时刻A虫群和B虫群数量之和，t+1时刻B虫群的数量等于t时刻A虫群的数量。由于星际空间的时间维度很广阔，所以t可能很大。OverMind 想知道在t时刻A虫群的数量对 p = 1,000,000,007.取余数的结果。当t=1时 A种群和B种群的数量均为1。

输入格式

　　测试数据包含一个整数t，代表繁殖的时间。

输出格式

　　输出一行，包含一个整数，表示对p取余数的结果

样例输入

　　10

样例输出

　　89

样例输入

　　65536

样例输出

　　462302286

数据规模和约定

　　对于50%的数据 t<=10^9
　　对于70%的数据 t<=10^15
　　对于100%的数据 t<=10^18

思路：求斐波那契数列第n项，但是数据范围太大，O(n)的时间复杂度会超时。

朴素的求斐波那契数列会超时

#include <iostream>
using namespace std; 

const int mod = 1000000007;

typedef long long ll;

int main() 
{
    ll n;
    cin >> n;
    ll a = 1;
    ll b = 1;
    ll ans = 1;
    for (int i = 2; i <= n; i++) 
    {
        int temp = a;
        a = (a % mod + b % mod) % mod;
        b = temp; 
    }
    cout << a << endl;
    return 0;
}
// 超时代码

这里我们先引入复习一下快速幂模板

#include <iostream>
using namespace std; 
typedef long long ll;

ll mul(ll a, ll b, ll p)
{
    ll ans = 1;
    while (b)
    {
        if (b & 1) ans = ans * a % p;
        a = a * a % p;
        b >>= 1;
    }
    return ans ;
}

int main() 
{
    ll a, b, p;
    cin >> a >> b >> p;
    cout << mul(a, b, p) << endl;
    return 0;
}

这里贴上新学习的矩阵快速幂的模板

个人认为比较好理解一些

#include <iostream>
#include <cstdio>
#include <algorithm>
#include <cstring>

using namespace std; 

typedef long long ll;

const int N = 105, mod = 1000000007;

int n;
ll k;

struct Mat
{
    ll a[N][N]; // longlong存矩阵 否则过程中会爆掉
    Mat()
    {
        memset(a, 0, sizeof a);
    }
    inline void build() //建造单位矩阵
    {
        for (int i = 1; i <= n; i ++ ) a[i][i] = 1;
    }
}mat;

Mat operator * (const Mat &x, const Mat &y) //重载运算符
{
    Mat z;
    for (int k = 1; k <= n; k ++ )
        for (int i = 1; i <= n; i ++ )
            for (int j = 1; j <= n; j ++ )
                z.a[i][j] = (z.a[i][j] + x.a[i][k] * y.a[k][j] % mod) % mod;
            
    return z;
}

int main() 
{
    cin >> n >> k;
    for (int i = 1; i <= n; i ++)
        for (int j = 1; j <= n; j ++ )
            cin >> mat.a[i][j];
    
    Mat ans; 
    ans.build(); // 实际相当于求快速幂中ans=1 这里ans为矩阵 所以初始化为单位矩阵
    while (k)
    {
        if (k & 1) ans = ans * mat;
        mat = mat * mat;
        k >>= 1;
    }
    for (int i = 1; i <= n; i ++ )
    {
        for (int j = 1; j <= n; j ++ )
            cout << ans.a[i][j] << ' ';
        cout << endl;
    }
    return 0;
}

在求斐波那契数列 可以使用矩阵快速幂

矩阵快速幂是用来求解递推式的，所以第一步先要列出递推式:

 f(n)=f(n-1)+f(n-2)

第二步是建立矩阵递推式，找到转移矩阵:

,简写成T * A(n-1)=A(n)，T矩阵就是那个2*2的常数矩阵，而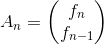

这里就是个矩阵乘法等式左边:1*f(n-1)+1*f(n-2)=f(n);1*f(n-1)+0*f(n-2)=f(n-1);

所以这里相乘就是矩阵n-1次相乘,然后输出第一行第二个元素,也就是a[0][1]；

把第一个矩阵设为A，第二个矩阵设为B，第三个矩阵设为C。

总而言之 ：最终斐波那契数列可以从矩阵中对应此公式

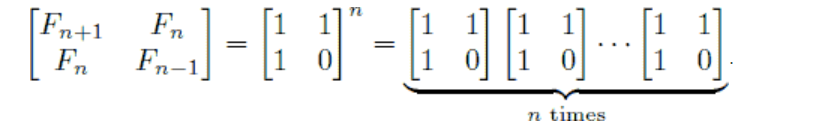
 由 F2  F1  F1 F0   组成的矩阵的n次方  的左下角就是Fn。

所以可以上代码 

注：此题和标准的斐波那契数列(1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89, 144, 233, 377, ...)有不同处 

标准的即f0=0,f1=1,f2=1,f3=2  而此题中f1=1,f2=2

所以我们最后实则应该求f的n+1项

#include <iostream>
#include <cstdio>
#include <algorithm>
#include <cstring>

using namespace std; 

typedef long long ll;

const int mod = 1000000007;

ll n, k;

struct Mat
{
    ll a[2][2];
    Mat()
    {
        memset(a, 0, sizeof a);
    }
    inline void build()
    {
        for (int i = 0; i < 2; i ++ )
            a[i][i] = 1;
    }
}base;

Mat operator * (const Mat &x, const Mat &y)
{
    Mat z;
    for (int k = 0; k < 2; k ++ )
        for (int i = 0; i < 2; i ++ )
            for (int j = 0; j < 2; j ++) 
                z.a[i][j] = (z.a[i][j] + x.a[i][k] * y.a[k][j] % mod) % mod;
    return z;
}

int main() 
{
    cin >> n;
    base.a[0][0] = 1, base.a[0][1] = 1, base.a[1][0] = 1, base.a[1][1] = 0; //将base初始化为要求n次幂的矩阵
    Mat ans;
    ans.build();
    while (n)
    {
        if (n & 1) ans = ans * base;
        base = base * base;
        n >>= 1;
    }
    cout << ans.a[0][0] << endl;
    // cout << ans.a[1][0] << endl; 注意这一道题的f1=1 f2=2 和标准的斐波那契数列有一定区别
    return 0;
}