codeforces 86D D. Powerful array(莫队算法)

题目链接：

D. Powerful array

time limit per test

5 seconds

memory limit per test

256 megabytes

input

standard input

output

standard output

An array of positive integers a1, a2, ..., an is given. Let us consider its arbitrary subarray al, al + 1..., ar, where 1 ≤ l ≤ r ≤ n. For every positive integer s denote by Ks the number of occurrences of s into the subarray. We call the power of the subarray the sum of productsKs·Ks·s for every positive integer s. The sum contains only finite number of nonzero summands as the number of different values in the array is indeed finite.

You should calculate the power of t given subarrays.

Input

First line contains two integers n and t (1 ≤ n, t ≤ 200000) — the array length and the number of queries correspondingly.

Second line contains n positive integers ai (1 ≤ ai ≤ 106) — the elements of the array.

Next t lines contain two positive integers l, r (1 ≤ l ≤ r ≤ n) each — the indices of the left and the right ends of the corresponding subarray.

Output

Output t lines, the i-th line of the output should contain single positive integer — the power of the i-th query subarray.

Please, do not use %lld specificator to read or write 64-bit integers in C++. It is preferred to use cout stream (also you may use%I64d).

Examples

input

3 2
1 2 1
1 2
1 3

output

3
6

input

8 3
1 1 2 2 1 3 1 1
2 7
1 6
2 7

output

20
20
20

Note

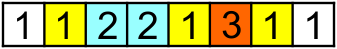
Consider the following array (see the second sample) and its [2, 7] subarray (elements of the subarray are colored):

Then K1 = 3, K2 = 2, K3 = 1, so the power is equal to 32·1 + 22·2 + 12·3 = 20.

AC代码:

#include <bits/stdc++.h>
using namespace std;
const int N=1e6+4;
int n,t;
long long a[N],num[N],ans[N];
struct node
{
   /* friend bool operator< ()
    {

    }*/
    int l,r,id,pos;
};
node qu[N];
int cmp(node x,node y)
{
    if(x.pos==y.pos)return x.r<y.r;
        return x.l<y.l;
}
void solve()
{
    long long temp=0;
    int le=1,ri=0;
    for(int i=1;i<=t;i++)
    {
        while(ri<qu[i].r)
        {
            ri++;
            temp+=((num[a[ri]]<<1)+1)*a[ri];
            num[a[ri]]++;
        }
        while(ri>qu[i].r)
        {
            num[a[ri]]--;
            temp-=((num[a[ri]]<<1)+1)*a[ri];
            ri--;
        }
        while(le<qu[i].l)
        {
            num[a[le]]--;
            temp-=((num[a[le]]<<1)+1)*a[le];
            le++;
        }
        while(le>qu[i].l)
        {
            le--;
            temp+=((num[a[le]]<<1)+1)*a[le];
            num[a[le]]++;
        }
        ans[qu[i].id]=temp;
    }
}

int main()
{
    scanf("%d%d",&n,&t);
    for(int i=1;i<=n;i++)
    {
        scanf("%I64d",&a[i]);
    }
    int sq=sqrt(n);
    for(int i=1;i<=t;i++)
    {
        scanf("%d%d",&qu[i].l,&qu[i].r);
        qu[i].id=i;
        qu[i].pos=qu[i].l/sq;
    }
    sort(qu+1,qu+t+1,cmp);
    solve();
    for(int i=1;i<=t;i++)
    {
        printf("%I64d
",ans[i]);
    }
    return 0;
}