cf437B The Child and Set

B. The Child and Set

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

At the children's day, the child came to Picks's house, and messed his house up. Picks was angry at him. A lot of important things were lost, in particular the favorite set of Picks.

Fortunately, Picks remembers something about his set S:

• its elements were distinct integers from 1 to limit;
• the value of 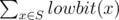 was equal to sum; here lowbit(x) equals 2k where k is the position of the first one in the binary representation of x. For example, lowbit(100102) = 102, lowbit(100012) = 12, lowbit(100002) = 100002 (binary representation).

Can you help Picks and find any set S, that satisfies all the above conditions?

Input

The first line contains two integers: sum, limit (1 ≤ sum, limit ≤ 105).

Output

In the first line print an integer n (1 ≤ n ≤ 105), denoting the size of S. Then print the elements of set S in any order. If there are multiple answers, print any of them.

If it's impossible to find a suitable set, print -1.

Sample test(s)

input

5 5

output

2
4 5

input

4 3

output

3
2 3 1

input

5 1

output

-1

Note

In sample test 1: lowbit(4) = 4, lowbit(5) = 1, 4 + 1 = 5.

In sample test 2: lowbit(1) = 1, lowbit(2) = 2, lowbit(3) = 1, 1 + 2 + 1 = 4.

贪心……从第一位向上贪心，然后每次往上合并。

#include<iostream>
#include<cstdio>
#include<cstdlib>
#include<cmath>
#include<cstring>
#include<algorithm>
using namespace std;
int bh[20][100010],fa[20][100010],gs[20];
int pre[100010];
int n,limt,sum,ans[100010];
int getw(int x)
{
    int res=0;
    while(x)
    {
       x=x>>1;res++;
    }
    return res-1;
}
void solve()
{
    int i,j;
    for(i=0;i<=18;i++) 
    {
        if((1<<i) & sum) 
        {
            if(gs[i]>0)
            {
                for(j=bh[i][gs[i]];j;j=pre[j]) ans[++ans[0]]=j;
                gs[i]--;
            }
            else {printf("-1
");return;}
        }
        for(j=1;j<=gs[i];j+=2)
        {
            if(j+1<=gs[i])
            {
                pre[fa[i][j+1]]=bh[i][j];
                gs[i+1]++;bh[i+1][gs[i+1]]=bh[i][j+1];fa[i+1][gs[i+1]]=fa[i][j];
            }
        }
    }
    printf("%d
",ans[0]);
    for(i=1;i<=ans[0];i++) printf("%d ",ans[i]);
}
int main()
{
    int i,x,y;
    cin>>sum>>limt;    
    for(i=1;i<=limt;i++)
    {
       x=(i & (-i));
       y=getw(x);
       gs[y]++;
       fa[y][gs[y]]=i;bh[y][gs[y]]=i;
    }
    solve();
    return 0;
}