SPOJ

http://www.spoj.com/problems/PGCD/en/

题意：

给出a，b区间，求该区间内满足gcd(x,y)=质数的个数。

思路：

设f（n）为 gcd（x，y）=p的个数，那么F（n）为 p | gcd（x，y）的个数，显然可得F（n）=（x/p）*（y/p）。

这道题目因为可以是不同的质数，所以需要枚举质数，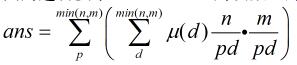

但是这样枚举太耗时，所以在这里令t=pk，

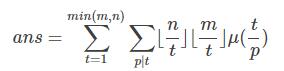
这样一来的话，我们只需要预处理u（t/p）的前缀和，之后像之前的题一样分块处理就可以了。

#include<iostream>
#include<algorithm>
#include<cstring>
#include<cstdio>
#include<sstream>
#include<vector>
#include<stack>
#include<queue>
#include<cmath>
#include<map>
#include<set>
using namespace std;
typedef long long ll;
typedef pair<int,int> pll;
const int INF = 0x3f3f3f3f;
const int maxn = 1e7 + 100;

int a, b;

bool check[maxn];
int prime[maxn];
int mu[maxn];
ll sum[maxn];

void Mobius()
{
    memset(check, false, sizeof(check));
    mu[1] = 1;
    int tot = 0;
    for (int i = 2; i <= maxn; i++)
    {
        if (!check[i])
        {
            prime[tot++] = i;
            mu[i] = -1;
        }
        for (int j = 0; j < tot; j++)
        {
            if (i * prime[j] > maxn)
            {
                break;
            }
            check[i * prime[j]] = true;
            if (i % prime[j] == 0)
            {
                mu[i * prime[j]] = 0;
                break;
            }
            else
            {
                mu[i * prime[j]] = -mu[i];
            }
        }
    }

    sum[0]=0;
    for(int i=0;i<tot;i++)
    {
        for(int j=prime[i];j<maxn;j+=prime[i])
        {
            sum[j]+=mu[j/prime[i]];
        }
    }
    for(int i=1;i<maxn;i++)
        sum[i]+=sum[i-1];
    return ;
}

ll solve(int n, int m)
{
    if(n>m)  swap(n,m);
    ll ans=0;

    for(int i=1,last=0;i<=n;i=last+1)
    {
        last=min(n/(n/i),m/(m/i));
        ans+=(sum[last]-sum[i-1])*(n/i)*(m/i);
    }
    return ans;
}


int main()
{
    //freopen("in.txt","r",stdin);
    int T;
    Mobius();

    scanf("%d",&T);
    while(T--)
    {
        scanf("%d%d",&a,&b);
        ll ans = solve(a,b);
        printf("%lld
",ans);
    }
    return 0;
}