参考资料:Palindromic Tree——回文树【处理一类回文串问题的强力工具】(请注意,其中似乎有一些错误)
回文自动机似乎和回文树是同一个东西qwq?
回文自动机(PAM)是一种处理回文串的工具。它的每个结点表示一个本质不同的回文串,转移边(c)表示在当前字符串的首尾分别加一个字符(c)。
回文自动机由两棵树组成,根结点分别称为(odd)和(even)。(even)表示空串,长度为(0),长度为偶数的回文串在它的子树上;(odd)表示一个“虚拟”的串,长度为(-1),长度为奇数的回文串在它的子树上。(odd)的直接儿子表示只有一个字母的回文串。沿着转移边(c)走一步就在当前串首尾各加上一个字符(c)。和AC自动机类似,一个结点的(fail)指针指向它的最长回文真后缀(定义(fail[even]=odd))。比如wqwqqwqwq的回文自动机长这样(数字表示结点编号,红箭头表示(fail)指针):
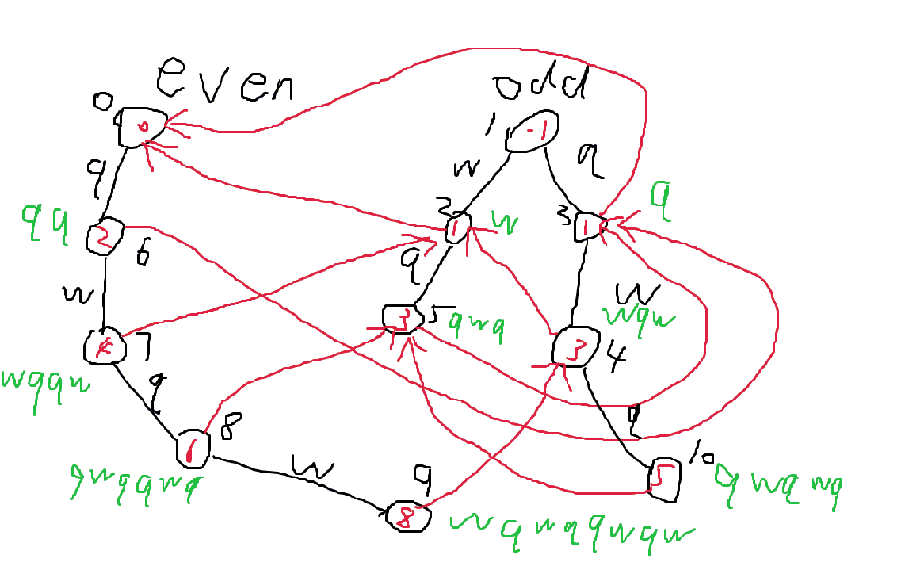
我画着画着发现这个字符串里回文串比想象的多
和后缀自动机类似,构造回文自动机也采用每次插入一个字符的方法。设原串是(S),当前位置是(pos),要加入的字符是(c),则可能会多一些以字符(c)结尾的回文串。而多的这些字符串可以看成是一个回文串([a,pos-1])满足(S_{a-1}=c)后面加一个字符(c)。于是要找到最长的这样的回文串([a,pos-1]),即从(pos-1)这个结点开始爬(fail)链,直到(p)点满足(S_{pos-len[p]-1}=S_{pos})。爬(fail)链最终会到长度为(-1)的(even),由于(pos-(-1)-1=pos),所以这个式子最终一定会成立。这个过程即代码中的(get\_fail)函数。
设第一个满足如上条件的点是(p)。如果(p)已经有了(c)字符的转移,则直接增加它的(cnt)(该字符串出现次数)即可;如果没有,则新建结点(q),(q)的长度显然是(p)的长度加(2),(q)的(fail)是从(p)的(fail)往上爬,找到第一个在后面加字符(c)仍为回文串的地方(方法同上述(get\_fail)),把它加字符(c)后转移到的点作为(q)的(fail)。
注意,如果要统计每个回文串的出现次数(即(cnt)),建完后要在(fail)树上做一遍树上递推(因为插入的时候只在当前点结尾的最长回文串的结点(cnt)上加(1)。如果一个串出现了,它的最长回文真后缀一定也出现了)。由于回文自动机是两棵树,所以不需要像后缀自动机求(Right)集合大小一样拓扑排序,只要按标号从大到小做即可。
题目:洛谷3649
把每个结点的长度(len)乘上出现次数(cnt)然后加起来就好了。
代码:
#include <cstdio>
#include <algorithm>
#include <cctype>
#include <cstring>
#include <string>
#define _ 0
using namespace std;
namespace zyt
{
const int N = 3e5 + 10;
template<typename T>
inline bool read(T &x)
{
char c;
bool f = false;
x = 0;
do
c = getchar();
while (c != EOF && c != '-' && !isdigit(c));
if (c == EOF)
return false;
if (c == '-')
f = true, c = getchar();
do
c = getchar();
while (isdigit(c));
if (f)
x = -x;
return true;
}
inline bool read(string &s)
{
static char buf[N];
if (scanf("%s", buf) == -1)
return false;
else
{
s = buf;
return true;
}
}
template<typename T>
inline void write(T x)
{
static char buf[20];
char *pos = buf;
if (x < 0)
putchar('-'), x = -x;
do
*pos++ = x % 10 + '0';
while (x /= 10);
while (pos > buf)
putchar(*--pos);
}
const int CH = 26;
typedef long long ll;
string s;
namespace Palindrome_Auto_Machine
{
struct node
{
int len, cnt, fail, s[CH];
}tree[N];
int cnt, last, odd, even, pos;
char s[N];
void init()
{
last = even = 0, odd = 1, cnt = 1, pos = 0;
s[0] = '#';
tree[odd].len = -1, tree[even].len = 0;
tree[odd].fail = tree[even].fail = odd;
}
int get_fail(int p)
{
while (s[pos - tree[p].len - 1] != s[pos])
p = tree[p].fail;
return p;
}
void insert(const char c)
{
s[++pos] = c;
int x = c - 'a', p = get_fail(last);
if (!tree[p].s[x])
{
tree[++cnt].len = tree[p].len + 2;
tree[cnt].fail = tree[get_fail(tree[p].fail)].s[x];
tree[p].s[x] = cnt;
}
last = tree[p].s[x];
++tree[last].cnt;
}
void build(const string &str)
{
for (int i = 0; i < str.size(); i++)
insert(str[i]);
for (int i = cnt; i > 0; i--)
tree[tree[i].fail].cnt += tree[i].cnt;
}
inline ll solve()
{
ll ans = 0;
for (int i = 0; i <= cnt; i++)
ans = max(ans, (ll)tree[i].cnt * tree[i].len);
return ans;
}
}
int work()
{
using Palindrome_Auto_Machine::init;
using Palindrome_Auto_Machine::build;
using Palindrome_Auto_Machine::solve;
read(s);
init();
build(s);
write(solve());
return (0^_^0);
}
}
int main()
{
return zyt::work();
}