二叉树的遍历-链式存储

二叉树的遍历-链式存储

利用指针域我们便可以完美的存储非完全二叉树，如下：

结构体

struct TreeNode
{
    TreeNode*left;
    TreeNode*right;
    char data;
};
typedef  TreeNode* BTree;

建树

BTree  CreateBTree()
{
    BTree bt = NULL;
    char ch;
    cin>>ch;
    if (ch != '#')
    {
        bt = new TreeNode;
        bt->data = ch;
        bt->left = CreateBTree();
        bt->right = CreateBTree();
    }
    return bt;
}

 

前序访问的递归写法  

void PreOrder(TreeNode*root){
    if (root==NULL)
    {
        return;            //若结点为空
    }
    cout<<root->data;         //输出根节点的值
    PreOrder(root->left);     //前序访问左子树
    PreOrder(root->right);    //前序访问右子树
}

前序访问的非递归写法   ---先根次序周游

void PreOrderLoop(TreeNode*root){
    stack<TreeNode*>s;
    TreeNode*cur,*top;
    cur = root;
    while(cur!=NULL||!s.empty()){
        while (cur!=NULL)
        {
            cout<<cur->data;
            s.push(cur);
            cur=cur->left;
        }
        top=s.top();
        s.pop();
        cur =top->right;
    }
}

 

 

中序遍历的递归写法

void InOrder(TreeNode*root){
    if(root==NULL){
       return; //判断节点是否为空
    }
    InOrder(root->left); //中序遍历左子树
    cout<<root->data; //访问节点值
    InOrder(root->right);  //中序遍历右子树
}

 

 

 

中序访问的非递归写法      ---对称周游

void InOrderLoop(TreeNode *root){
    stack<TreeNode *>s;
    TreeNode *cur;
    cur=root;
    while (cur!=NULL||!s.empty()){
        while(cur!=NULL){
            s.push(cur);
            cur=cur->left;
        }
        cur = s.top();
        s.pop();
        cout<<cur->data;
        cur=cur->right;
    }
}

 

 

后序遍历的递归写法

void PostOrder(TreeNode*root){
    if(root==NULL){
        return; //判断节点是否为空
    }
    InOrder(root->left); //后序遍历左子树
    InOrder(root->right);  //后序遍历右子树
    cout<<root->data; //访问节点值
}

 

 

后序访问的非递归写法    --后跟周游

void PostOrderLoop(TreeNode *root){
    stack<TreeNode *>s;
    TreeNode *cur,*top,*last=NULL;
    cur=root;
    while (cur!=NULL||!s.empty()){
        while(cur!=NULL){
            s.push(cur);
            cur=cur->left;
        }
        top = s.top();
        if(top->right==NULL||top->right==last){
            s.pop();
            cout<<top->data;
            last=top;
        }else{
            cur =top->right;
        }
    }
}

 

 

 

层序遍历   -- -广度优先周游

void LevelOrder(TreeNode* root){
    queue<TreeNode*>q;
    TreeNode *front;
    if(root==NULL)return;
    q.push(root);
    while (!q.empty())
    {
        front = q.front();
        q.pop();
        if (front->left)
        {
            q.push(front->left);
        }
        if (front->right)
        {
            q.push(front->right);
        }
        cout<<front->data;
        
    }
}

 

 

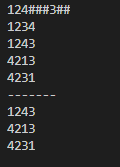
测试结果

124###3##

统计叶子结点的数目,方法一

 

int leftCount=0;
void leaf1(BTree root){
    if(root!=NULL){
        leaf1(root->left);
        leaf1(root->right);
        if(root->left==NULL&&root->right==NULL){
            leftCount++;
        }
    }
}

 

 

 

统计叶子结点的数目,方法二

int leaf2(BTree root){
    int leftC=0;
    if(root==NULL)leftCount=0;
    else if (root->left==NULL&&root->right==NULL)
    {
        leftC=1;
    }else
    {
        leftC=leaf2(root->left)+leaf2(root->right);
    }
    return leftC;
}

 

 

求二叉树的高度

 

int TreeDepth(BTree bt)
{
    int hl,hr,max;
    if(bt!=NULL)
    {
        hl=TreeDepth(bt->left);
        hr=TreeDepth(bt->right);
        max=hl>hr?hl:hr;
        return(max+1);
    }
    else return(0);
}