首先证明 $d(ij)=sum_{k|i}sum_{l|j}[gcd(k,l)==1]$
把 $i,j$ 考虑成唯一分解后的形式:$P_{i1}^{k1}P_{i2}^{k2}...P_{in}^{kn}$
对于 $i,j$ 中某个相同的质因子 $P_{x}$ ,$i=...P_{x}^{ki}...$,$j=...P_{x}^{kj}...$
如果枚举到 $j$ 中因数 $l$ 时,$l$ 有因数 $P_{x}^{t}$,不妨看成 $P_{x}^{ki+t}$,
如果这样看,那么会发现枚举 $k,l$ 时一定能把 $P_{x}$ 从 $P_{x}^{1}$ 枚举到 $P_{x}^{ki+kj}$,这样就包括了所有情况
会不会重复算呢,显然因为式子 $d(ij)=sum_{k|i}sum_{l|j}[gcd(k,l)==1]$ 要满足 $k,l$ 没有相同的质因数才有贡献
所以枚举时对于同一个质因数一定不会在既在 $k$ 中有,又在 $l$ 中有,
即不会出现枚举成 $P_{x}^{ki+t+y}$,其中 $y$ 是 $k$ 对 $P$ 的贡献(此时 $k$ 的贡献已经全在 $ki$ 中了,导致不合法)
要么是 $P_{x}^{ki+t}$ 要么是 $P_{x}^{y}$
所以最后我们证明了 $d(ij)=sum_{k|i}sum_{l|j}[gcd(k,l)==1]$
那么 $Ans=sum_isum_jd(ij)=sum_{k|i}sum_{l|j}[gcd(k,l)==1]$
有 $gcd$ 了,显然莫比乌斯反演:
设 $f[x]=sum_isum_jsum_{k|i}sum_{l|j}[gcd(k,l)==x]$,答案即为 $f[1]$
设 $F[x]=sum_isum_jsum_{k|i}sum_{l|j}[x|gcd(k,l)]$,那么有 $F[x]=sum_{x|d}f[d]$,直接反演得到
$f[1]=sum_dmu(d)sum_isum_jsum_{k|i}sum_{l|j}[d|gcd(k,l)]$
变成先枚举 $k,l$ 再枚举 $k,l$ 的倍数 $i',j'$,使得 $ki'=i,kj'=j$,变成
$f[1]=sum_dmu(d)sum_ksum_l[d|gcd(k,l)]sum_{i'}^{left lfloor frac{N}{k} ight floor}sum_{j'}^{left lfloor frac{M}{l} ight floor}\=sum_dmu(d)sum_{k}^{N}sum_{l}^{M}[d|gcd(k,l)]left lfloor frac{N}{k} ight floorleft lfloor frac{M}{l} ight floor$
把枚举数 $k,l$ 变成枚举 $d$ 的倍数 $k',l'$,使得 $k=k'd,l=l'd$,得到
$f[1]=sum_dmu(d)sum_{k'}^{left lfloor frac{N}{d} ight floor}sum_{l'}^{left lfloor frac{M}{d} ight floor}left lfloor frac{N}{k'd} ight floorleft lfloor frac{M}{l'd} ight floor\=sum_dmu(d)(sum_{k'}^{left lfloor frac{N}{d} ight floor}left lfloor frac{N}{k'd} ight floor)(sum_{l'}^{left lfloor frac{M}{d} ight floor}left lfloor frac{M}{l'd} ight floor)\=sum_dmu(d)(sum_{k'}^{left lfloor frac{N}{d} ight floor}left lfloor frac{left lfloor frac{N}{d} ight floor}{k'} ight floor)(sum_{l'}^{left lfloor frac{M}{d} ight floor}left lfloor frac{left lfloor frac{M}{d} ight floor}{l'} ight floor)$
只要预处理出 $sum[x]=sum_{i}^{x}left lfloor frac{x}{i} ight floor$ 就好了
最后附上完整过程:

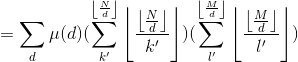
#include<iostream> #include<cstdio> #include<algorithm> #include<cstring> #include<cmath> using namespace std; typedef long long ll; inline int read() { int x=0,f=1; char ch=getchar(); while(ch<'0'||ch>'9') { if(ch=='-') f=-1; ch=getchar(); } while(ch>='0'&&ch<='9') { x=(x<<1)+(x<<3)+(ch^48); ch=getchar(); } return x*f; } const int N=2e7+7; int Q,n,m,mi,pri[N],mu[N],tot; ll sum[N],ans; bool not_pri[N]; void pre() { not_pri[1]=1; mu[1]=1; for(int i=2;i<5e4;i++) { if(!not_pri[i]) pri[++tot]=i,mu[i]=-1; for(int j=1;j<=tot;j++) { ll t=1ll*i*pri[j]; if(t>5e4) break; not_pri[t]=1; if(!(i%pri[j])) break; mu[t]=-mu[i]; } } for(int i=2;i<=5e4;i++) mu[i]+=mu[i-1]; } int main() { Q=read(); pre(); for(int i=1;i<=5e4;i++) { for(int l=1,r;l<=i;l=r+1) { r=i/(i/l); sum[i]+=1ll*(r-l+1)*(i/l); } } while(Q--) { n=read(),m=read(); mi=min(n,m); ans=0; for(int l=1,r;l<=mi;l=r+1) { r=min(n/(n/l),m/(m/l)); ans+=1ll*(mu[r]-mu[l-1])*sum[n/l]*sum[m/l]; } printf("%lld ",ans); } return 0; }