D

题目链接：http://codeforces.com/problemset/problem/599/D

D. Spongebob and Squares

time limit per test

2 seconds

memory limit per test

256 megabytes

input

standard input

output

standard output

Spongebob is already tired trying to reason his weird actions and calculations, so he simply asked you to find all pairs of n and m, such that there are exactly x distinct squares in the table consisting of n rows and m columns. For example, in a 3 × 5 table there are 15 squares with side one, 8 squares with side two and 3 squares with side three. The total number of distinct squares in a 3 × 5 table is 15 + 8 + 3 = 26.

Input

The first line of the input contains a single integer x (1 ≤ x ≤ 1018) — the number of squares inside the tables Spongebob is interested in.

Output

First print a single integer k — the number of tables with exactly x distinct squares inside.

Then print k pairs of integers describing the tables. Print the pairs in the order of increasing n, and in case of equality — in the order of increasing m.

Examples

input

Copy

26

output

Copy

6
1 26
2 9
3 5
5 3
9 2
26 1

input

Copy

2

output

Copy

2
1 2
2 1

input

Copy

8

output

Copy

4
1 8
2 3
3 2
8 1

Note

In a 1 × 2 table there are 2 1 × 1 squares. So, 2 distinct squares in total.

In a 2 × 3 table there are 6 1 × 1 squares and 2 2 × 2 squares. That is equal to 8 squares in total.

                                                                                                  

题目大意：对于给定的X，找出所有的 M*N 矩阵，使得其中恰含 X 个正方形

思路：自己推导可以发现，对于任意一个n*m的矩阵，正方形个数为 n*m个边长为1的，(n-1)(m-1)个边长为2的....

也就是

得到这个公式我们还是没法求啊 ，因为K的范围是1e18，我们不可能枚举所有的n和m吧，那么我们看看这个公式

稍作分析，其实我们可以化简得到

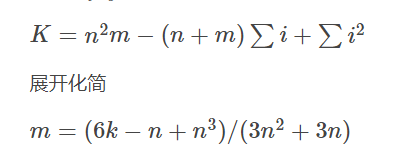
到这就好求啦，我们只要枚举n，假设所有的n<=m就行了，直接算出m的值，这样复杂度就降下来了

看代码：

#include<iostream>
#include<cstdio>
#include<algorithm>
#include<cstring>
#include<vector>
#include<stack>
#include<map>
using namespace std;
typedef long long LL;
#define sc1(a) scanf("%lld",&a);
#define pf1(a) printf("%lld
",a)
const int INF=1e9+7;
const int maxn=1e6+5;
const int maxv=1e6+5;
const int mod=998244353;
/**
m=(6x−n+n^3)/(3n^2+3n)
*/
vector<pair<LL,LL> >v;
int main()
{
//    freopen("in.txt","r",stdin);
    LL X;sc1(X);
    ///假设所有的n<=m
    for(LL i=1;;i++)
    {
        LL n=i;
        LL m=((LL)6*X-n+(LL)n*n*n)/((LL)3*n*n+3*n);
        if(n>m) break;
        if(m*((LL)3*n*n+(LL)3*n)==((LL)6*X-n+(LL)n*n*n))
        {
            v.push_back(make_pair(n,m));
        }
    }
    ///特判两个相等的情况!!!   这个要记得 反过来输出一定要特判两个数相等的情况

    LL sum=v.size()<<1;
    if(v.back().first==v.back().second) sum--;
    pf1(sum);
    for(int i=0;i<v.size();i++)
    {
        printf("%lld %lld
",v[i].first,v[i].second);
    }
    if(v.back().first==v.back().second) v.pop_back();
    for(int i=v.size()-1;i>=0;i--)
    {
        printf("%lld %lld
",v[i].second,v[i].first);
    }
    return 0;
}
/**

*/