POJ3070Fibonacci（矩阵快速幂求Fibonacci数列）

Fibonacci

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 7241		Accepted: 5131

Description

In the Fibonacci integer sequence, F₀ = 0, F₁ = 1, and F_n = F_{n − 1} + F_{n − 2} for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …

An alternative formula for the Fibonacci sequence is

.

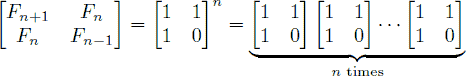Given an integer n, your goal is to compute the last 4 digits of F_n.

Input

The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.

Output

For each test case, print the last four digits of F_n. If the last four digits of F_n are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print F_n mod 10000).

Sample Input

0
9
999999999
1000000000
-1

Sample Output

0
34
626
6875

Hint

As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by

.

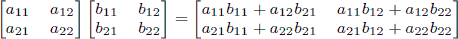Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:

.

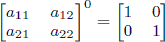Source

Stanford Local 2006

 

分析：通过这道题，不仅学会了矩阵的快速幂的做法，同时也提供了求Fibonacci的高效算法

代码一： 
   这题完全套用的是 一般的快速幂的做法，只不过改成矩阵乘法后，为了在做矩阵乘法过程中不会影响结果值，
之间要用中间变量，代码写的很难看 （没有想到可以用结构体对二维数组进行封装，可以直接返回结构体类型的数据）,
不过还是 0ms AC。 

#include <cstdio>
#include <iostream>

using namespace std;

const int MOD = 10000;

int fast_mod(int n)    // 求 (t^n)%MOD 
{
    int t[2][2] = {1, 1, 1, 0};
    int ans[2][2] = {1, 0, 0, 1};  // 初始化为单位矩阵
    int tmp[2][2];    //自始至终都作为矩阵乘法中的中间变量 
     
    while(n)
    {
        if(n & 1)  //实现 ans *= t; 其中要先把 ans赋值给 tmp，然后用 ans = tmp * t 
        {
            for(int i = 0; i < 2; ++i)
                for(int j = 0; j < 2; ++j)
                    tmp[i][j] = ans[i][j]; 
            ans[0][0] = ans[1][1] = ans[0][1] = ans[1][0] = 0;  // 注意这里要都赋值成 0 
            
            for(int i = 0; i < 2; ++i)    //  矩阵乘法 
            {
                for(int j = 0; j < 2; ++j)
                {
                    for(int k = 0; k < 2; ++k)
                        ans[i][j] = (ans[i][j] + tmp[i][k] * t[k][j]) % MOD;
                }
            }
        }
        
        //  下边要实现  t *= t 的操作，同样要先将t赋值给中间变量  tmp ，t清零，之后 t = tmp* tmp 
        for(int i = 0; i < 2; ++i)
            for(int j = 0; j < 2; ++j)
                tmp[i][j] = t[i][j];
        t[0][0] = t[1][1] = 0;
        t[0][1] = t[1][0] = 0;
        for(int i = 0; i < 2; ++i)
        {
            for(int j = 0; j < 2; ++j)
            {
                for(int k = 0; k < 2; ++k)
                    t[i][j] = (t[i][j] + tmp[i][k] * tmp[k][j]) % MOD;
            }
        }
        
        n >>= 1;
    }
    return ans[0][1];
}

int main()
{
    int n;
    while(scanf("%d", &n) && n != -1)
    {    
        printf("%d\n", fast_mod(n));
    }
    return 0;
}

代码二：用结构体封装矩阵乘法后，代码看着清晰多了

#include <cstdio>
#include <iostream>

using namespace std;

const int MOD = 10000;

struct matrix
{
    int m[2][2];
}ans, base;

matrix multi(matrix a, matrix b)
{
    matrix tmp;
    for(int i = 0; i < 2; ++i)
    {
        for(int j = 0; j < 2; ++j)
        {
            tmp.m[i][j] = 0;
            for(int k = 0; k < 2; ++k)
                tmp.m[i][j] = (tmp.m[i][j] + a.m[i][k] * b.m[k][j]) % MOD;
        }
    }
    return tmp;
}
int fast_mod(int n)  // 求矩阵 base 的  n 次幂 
{
    base.m[0][0] = base.m[0][1] = base.m[1][0] = 1;
    base.m[1][1] = 0;
    ans.m[0][0] = ans.m[1][1] = 1;  // ans 初始化为单位矩阵 
    ans.m[0][1] = ans.m[1][0] = 0;
    while(n)
    {
        if(n & 1)  //实现 ans *= t; 其中要先把 ans赋值给 tmp，然后用 ans = tmp * t 
        {
            ans = multi(ans, base);
        }
        base = multi(base, base);
        n >>= 1;
    }
    return ans.m[0][1];
}

int main()
{
    int n;
    while(scanf("%d", &n) && n != -1)
    {   
        printf("%d\n", fast_mod(n));
    }
    return 0;
}