3529: [Sdoi2014]数表
Time Limit: 10 Sec Memory Limit: 512 MBSubmit: 2321 Solved: 1187
[Submit][Status][Discuss]
Description
有一张N×m的数表，其第i行第j列（1 < =i < =礼，1 < =j < =m）的数值为
能同时整除i和j的所有自然数之和。给定a，计算数表中不大于a的数之和。
Input
输入包含多组数据。
输入的第一行一个整数Q表示测试点内的数据组数，接下来Q行，每行三个整数n，m，a(|a| < =10^9)描述一组数据。
Output
对每组数据，输出一行一个整数，表示答案模2^31的值。
Sample Input
2
4 4 3
10 10 5
4 4 3
10 10 5
Sample Output
20
148
148
HINT
1 < =N．m < =10^5 ， 1 < =Q < =2×10^4
Source
析：令F(i) 表示 i 的约数和，这个可以先预处理出来，然后先考虑没有 a 这个限制，令 g(i) 表示gcd(x,y)=i 数对(x,y)的个数，当然1<=x<=n,1<=y<=m，由莫比乌斯反演很容易就可以得到这个式子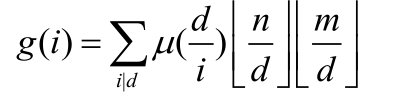
然后就能够得到
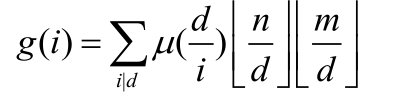然后就能够得到
枚举每一个i，暴力更新i的倍数，然后处理前缀和，这样做是O(nlogn)的，有的a的限制，我们可以先把所有的处理都离线，然后将询问按照a排序，i按照F(i)排序，每次询问将所有F(i)<=a的i按照之前的方式插入 用树状数组维护前缀和即可，最后关于取模，这是一个比较特殊的，可以用自然溢出，然后最后答案进行按位与操作也就是ans&2147483647。
代码如下：
#pragma comment(linker, "/STACK:1024000000,1024000000")
#include <cstdio>
#include <string>
#include <cstdlib>
#include <cmath>
#include <iostream>
#include <cstring>
#include <set>
#include <queue>
#include <algorithm>
#include <vector>
#include <map>
#include <cctype>
#include <cmath>
#include <stack>
#include <sstream>
#include <list>
#include <assert.h>
#include <bitset>
#include <numeric>
#define debug() puts("++++")
#define gcd(a, b) __gcd(a, b)
#define lson l,m,rt<<1
#define rson m+1,r,rt<<1|1
#define fi first
#define se second
#define pb push_back
#define sqr(x) ((x)*(x))
#define ms(a,b) memset(a, b, sizeof a)
#define sz size()
#define pu push_up
#define pd push_down
#define cl clear()
#define lowbit(x) -x&x
//#define all 1,n,1
#define FOR(i,x,n) for(int i = (x); i < (n); ++i)
#define freopenr freopen("in.txt", "r", stdin)
#define freopenw freopen("out.txt", "w", stdout)
using namespace std;
typedef long long LL;
typedef unsigned long long ULL;
typedef pair<int, int> P;
const int INF = 0x3f3f3f3f;
const LL LNF = 1e17;
const double inf = 1e20;
const double PI = acos(-1.0);
const double eps = 1e-8;
const int maxn = 1e5 + 5;
const int maxm = 2e4 + 10;
const LL mod = 1e9 + 7LL;
const int dr[] = {-1, 1, 0, 0, 1, 1, -1, -1};
const int dc[] = {0, 0, 1, -1, 1, -1, 1, -1};
const char *de[] = {"0000", "0001", "0010", "0011", "0100", "0101", "0110", "0111", "1000", "1001", "1010", "1011", "1100", "1101", "1110", "1111"};
int n, m;
const int mon[] = {0, 31, 28, 31, 30, 31, 30, 31, 31, 30, 31, 30, 31};
const int monn[] = {0, 31, 29, 31, 30, 31, 30, 31, 31, 30, 31, 30, 31};
inline bool is_in(int r, int c) {
return r >= 0 && r < n && c >= 0 && c < m;
}
bool vis[maxn];
int prime[maxn];
int mu[maxn];
void Moblus(){
mu[1] = 1;
int tot = 0;
for(int i = 2; i < maxn; ++i){
if(!vis[i]) prime[tot++] = i, mu[i] = -1;
for(int j = 0; j < tot && i * prime[j] < maxn; ++j){
int t = i * prime[j];
vis[t] = 1;
if(i % prime[j] == 0) break;
mu[t] = -mu[i];
}
}
}
struct Node{
int val, id;
bool operator < (const Node &p) const{
return val < p.val;
}
};
Node f[maxn];
struct Query{
int id, n, m, a;
bool operator < (const Query &q) const{
return a < q.a;
}
};
Query q[maxm];
void init(){
for(int i = 1; i < maxn; ++i){
f[i].id = i;
for(int j = i; j < maxn; j += i)
f[j].val += i;
}
sort(f+1, f + maxn);
}
int sum[maxn];
void add(int x, int val){
while(x < maxn){
sum[x] += val;
x += lowbit(x);
}
}
int query(int x){
int ans = 0;
while(x){
ans += sum[x];
x -= lowbit(x);
}
return ans;
}
int ans[maxn];
int solve(int n, int m){
if(n > m) swap(n, m);
int ans = 0;
for(int i = 1, det; i <= n; i = det + 1){
det = min(n/(n/i), m/(m/i));
ans += (query(det) - query(i-1)) * (n/i) * (m/i);
}
return ans & 2147483647;
}
void update(int x, int val){
for(int i = x, j = 1; i < maxn; i += x, ++j)
add(i, mu[j] * val);
}
int main(){
Moblus();
init();
int T; scanf("%d", &T);
for(int i = 0; i < T; ++i){
scanf("%d %d %d", &q[i].n, &q[i].m, &q[i].a);
q[i].id = i;
}
sort(q, q + T);
int j = 1;
for(int i = 0; i < T; ++i){
while(f[j].val <= q[i].a) update(f[j].id, f[j].val), ++j;
ans[q[i].id] = solve(q[i].n, q[i].m);
}
for(int i = 0; i < T; ++i) printf("%d
", ans[i]);
return 0;
}