Codeforces Round #250 (Div. 1) D. The Child and Sequence

D. The Child and Sequence

time limit per test

4 seconds

memory limit per test

256 megabytes

input

standard input

output

standard output

At the children's day, the child came to Picks's house, and messed his house up. Picks was angry at him. A lot of important things were lost, in particular the favorite sequence of Picks.

Fortunately, Picks remembers how to repair the sequence. Initially he should create an integer array a[1], a[2], ..., a[n]. Then he should perform a sequence of m operations. An operation can be one of the following:

1. Print operation l, r. Picks should write down the value of .
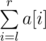2. Modulo operation l, r, x. Picks should perform assignment a[i] = a[i] mod x for each i (l ≤ i ≤ r).
3. Set operation k, x. Picks should set the value of a[k] to x (in other words perform an assignment a[k] = x).

Can you help Picks to perform the whole sequence of operations?

Input

The first line of input contains two integer: n, m (1 ≤ n, m ≤ 105). The second line contains n integers, separated by space:a[1], a[2], ..., a[n] (1 ≤ a[i] ≤ 109) — initial value of array elements.

Each of the next m lines begins with a number type .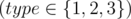

• If type = 1, there will be two integers more in the line: l, r (1 ≤ l ≤ r ≤ n), which correspond the operation 1.
• If type = 2, there will be three integers more in the line: l, r, x (1 ≤ l ≤ r ≤ n; 1 ≤ x ≤ 109), which correspond the operation 2.
• If type = 3, there will be two integers more in the line: k, x (1 ≤ k ≤ n; 1 ≤ x ≤ 109), which correspond the operation 3.

Output

For each operation 1, please print a line containing the answer. Notice that the answer may exceed the 32-bit integer.

Sample test(s)

input

5 5
1 2 3 4 5
2 3 5 4
3 3 5
1 2 5
2 1 3 3
1 1 3

output

8
5

input

10 10
6 9 6 7 6 1 10 10 9 5
1 3 9
2 7 10 9
2 5 10 8
1 4 7
3 3 7
2 7 9 9
1 2 4
1 6 6
1 5 9
3 1 10

output

49
15
23
1
9

线段树，至于区间取模，非常明显这个数会越来越小或者不变，所以我们记录下每一个区间的最大值，假设对于模mod操作发现某个区间的最大值比mod小，显然这个区间就不是必需进行操作了。
#include <stdio.h>
#include <string.h>
#include <algorithm>
using namespace std;

typedef long long ll;
#define rep(i,s,t) for(int i=s;i<t;i++)
#define red(i,s,t) for(int i=s-1;i>=t;i--)
#define max(a,b) (a>b?
a:b)
#define clr(a,v) memset(a,v,sizeof a)
#define L t<<1
#define R t<<1|1

inline int input(){
    int ret=0;bool isN=0;char c=getchar();
    while(c<'0' || c>'9'){
        if(c=='-') isN=1;
        c=getchar();
    }
    while(c>='0' && c<='9'){
        ret=ret*10+c-'0';c=getchar();
    }
    return isN?
-ret:ret;
}

inline void output(ll x){
    if(x<0){
        putchar('-');x=-x;
    }
    int len=0,data[20];
    while(x){
        data[len++]=x%10;x/=10;
    }
    if(!len)    data[len++]=0;
    while(len--)
        putchar(data[len]+48);
    putchar('
');
}

const int N=100005;
ll root[N<<2];
int Max[N<<2];
int n,m,a[N],op,x,y,z;

inline void push_up(int t){
    root[t]=root[L]+root[R];
    Max[t]=max(Max[L],Max[R]);
}

inline void build(int t,int x,int y){
    if(x==y) root[t]=Max[t]=a[x];
    else{
        int mid=(x+y)>>1;
        build(L,x,mid),build(R,mid+1,y);
        push_up(t);
    }
}

inline void setVal(int t,int l,int r,int x,int v){
    if(l==r){
        root[t]=Max[t]=v;
    }
    else{
        int mid=(l+r)>>1;
        if(x<=mid) setVal(L,l,mid,x,v);
        else setVal(R,mid+1,r,x,v);
        push_up(t);
    }
}

inline void modefiyMod(int t,int l,int r,int x,int y,int mod){
    if(Max[t]<mod) return;
    if(l==r){
        root[t]=Max[t]=root[t]%mod;return;
    }
    else{
        int mid=(l+r)>>1;
        if(y<=mid) modefiyMod(L,l,mid,x,y,mod);
        else if(x>mid) modefiyMod(R,mid+1,r,x,y,mod);
        else{
            modefiyMod(L,l,mid,x,mid,mod);
            modefiyMod(R,mid+1,r,mid+1,y,mod);
        }
        push_up(t);
    }
}

inline ll query(int t,int l,int r,int x,int y){
    if(l>=x && r<=y) return root[t];
    int mid=(l+r)>>1;
    if(y<=mid) return query(L,l,mid,x,y);
    else if(x>mid) return query(R,mid+1,r,x,y);
    else return query(L,l,mid,x,mid)+query(R,mid+1,r,mid+1,y);
}

int main(){
    n=input(),m=input();
    rep(i,1,n+1) a[i]=input();
    build(1,1,n);
    rep(i,0,m){
        op=input();
        if(op==1){
            x=input(),y=input();
            output(query(1,1,n,x,y));
        }
        else if(op==2){
            x=input(),y=input(),z=input();
            modefiyMod(1,1,n,x,y,z);
        }
        else{
            x=input(),y=input();
            setVal(1,1,n,x,y);
        }
    }
    return 0;
}