Print Article
Time Limit: 9000/3000 MS (Java/Others) Memory Limit: 131072/65536 K (Java/Others)
Total Submission(s): 11141 Accepted Submission(s): 3393
Problem Description
Zero has an old printer that doesn't work well sometimes. As it is antique, he still like to use it to print articles. But it is too old to work for a long time and it will certainly wear and tear, so Zero use a cost to evaluate this degree.
One day Zero want to print an article which has N words, and each word i has a cost Ci to be printed. Also, Zero know that print k words in one line will cost
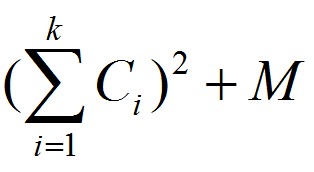
M is a const number.
Now Zero want to know the minimum cost in order to arrange the article perfectly.
One day Zero want to print an article which has N words, and each word i has a cost Ci to be printed. Also, Zero know that print k words in one line will cost
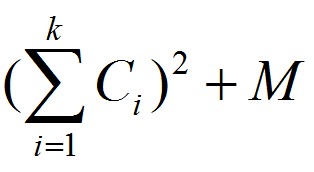
M is a const number.
Now Zero want to know the minimum cost in order to arrange the article perfectly.
Input
There are many test cases. For each test case, There are two numbers N and M in the first line (0 ≤ n ≤ 500000, 0 ≤ M ≤ 1000). Then, there are N numbers in the next 2 to N + 1 lines. Input are terminated by EOF.
Output
A single number, meaning the mininum cost to print the article.
Sample Input
5 5
5
9
5
7
5
Sample Output
230
Author
Xnozero
Source
题意:给你n个数以及M 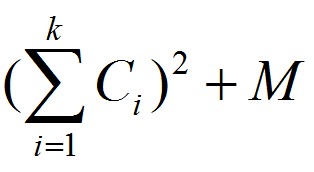 将n个数连续分成若干部分求 和的最小值
将n个数连续分成若干部分求 和的最小值
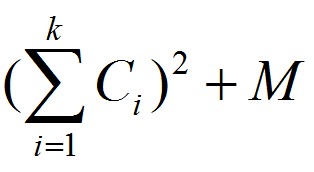 将n个数连续分成若干部分求 和的最小值
将n个数连续分成若干部分求 和的最小值题解:d[i]=dp[j]+m+(sum[i]-sum[j])*(sum[i]-sum[j]);
假设k<j<i 从j处分开比从k处分开更优则可以得到
dp[j]+m+(sum[i]-sum[j])*(sum[i]-sum[j])<dp[k]+m+(sum[i]-sum[k])*(sum[i]-sum[k]);
=>(dp[j]+sum[j]*sum[j]-(dp[k]+sum[k]*sum[k]))/2*(sum[j]-sum[k])<=sum[i] //演算一下
右侧 sum[i]是前缀和 是递增的
左侧 恒小于右式才能更新dp[i] 维护一个下凸包
1 #include <iostream> 2 #include <cstdio> 3 #include <cstdlib> 4 #include <cstring> 5 #include <algorithm> 6 #include <stack> 7 #include <queue> 8 #include <cmath> 9 #include <map> 10 #define ll __int64 11 using namespace std; 12 int dp[500005]; 13 int q[500005]; 14 int sum[500005]; 15 int head,tail,n,m; 16 int getDP(int i,int j) 17 { 18 return dp[j]+m+(sum[i]-sum[j])*(sum[i]-sum[j]); 19 } 20 int getUP(int j,int k) 21 { 22 return dp[j]+sum[j]*sum[j]-(dp[k]+sum[k]*sum[k]); 23 } 24 int getdown(int j,int k) 25 { 26 return 2*(sum[j]-sum[k]); 27 } 28 int main() 29 { 30 while(scanf("%d %d",&n,&m)==2) 31 { 32 for(int i=1; i<=n; i++) 33 scanf("%d",&sum[i]); 34 sum[0]=dp[0]=0; 35 for(int i=1; i<=n; i++) 36 sum[i]+=sum[i-1]; 37 head=tail=0; 38 q[tail++]=0; 39 for(int i=1; i<=n; i++) 40 { 41 while(head+1<tail && getUP(q[head+1],q[head])<=sum[i]*getdown(q[head+1],q[head])) 42 head++; 43 dp[i]=getDP(i,q[head]); 44 while(head+1<tail && getUP(i,q[tail-1])*getdown(q[tail-1],q[tail-2])<=getUP(q[tail-1],q[tail-2])*getdown(i,q[tail-1])) 45 tail--; 46 q[tail++]=i; 47 } 48 printf("%d ",dp[n]); 49 } 50 return 0; 51 }