Power Network
| Time Limit: 2000MS | Memory Limit: 32768K | |
| Total Submissions: 18309 | Accepted: 9643 |
Description
A power network consists of nodes (power stations, consumers and dispatchers) connected by power transport lines. A node u may be supplied with an amount s(u) >= 0 of power, may produce an amount 0 <= p(u) <= pmax(u) of power, may consume an amount 0 <= c(u) <= min(s(u),cmax(u)) of power, and may deliver an amount d(u)=s(u)+p(u)-c(u) of power. The following restrictions apply: c(u)=0 for any power station, p(u)=0 for any consumer, and p(u)=c(u)=0 for any dispatcher. There is at most one power transport line (u,v) from a node u to a node v in the net; it transports an amount 0 <= l(u,v) <= lmax(u,v) of power delivered by u to v. Let Con=Σuc(u) be the power consumed in the net. The problem is to compute the maximum value of Con.
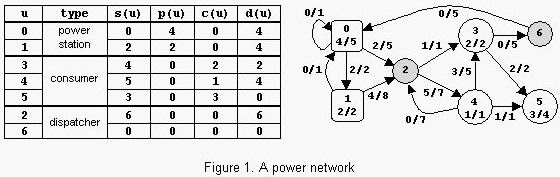
An example is in figure 1. The label x/y of power station u shows that p(u)=x and pmax(u)=y. The label x/y of consumer u shows that c(u)=x and cmax(u)=y. The label x/y of power transport line (u,v) shows that l(u,v)=x and lmax(u,v)=y. The power consumed is Con=6. Notice that there are other possible states of the network but the value of Con cannot exceed 6.

An example is in figure 1. The label x/y of power station u shows that p(u)=x and pmax(u)=y. The label x/y of consumer u shows that c(u)=x and cmax(u)=y. The label x/y of power transport line (u,v) shows that l(u,v)=x and lmax(u,v)=y. The power consumed is Con=6. Notice that there are other possible states of the network but the value of Con cannot exceed 6.
Input
There are several data sets in the input. Each data set encodes a power network. It starts with four integers: 0 <= n <= 100 (nodes), 0 <= np <= n (power stations), 0 <= nc <= n (consumers), and 0 <= m <= n^2 (power transport lines). Follow m data triplets (u,v)z, where u and v are node identifiers (starting from 0) and 0 <= z <= 1000 is the value of lmax(u,v). Follow np doublets (u)z, where u is the identifier of a power station and 0 <= z <= 10000 is the value of pmax(u). The data set ends with nc doublets (u)z, where u is the identifier of a consumer and 0 <= z <= 10000 is the value of cmax(u). All input numbers are integers. Except the (u,v)z triplets and the (u)z doublets, which do not contain white spaces, white spaces can occur freely in input. Input data terminate with an end of file and are correct.
Output
For each data set from the input, the program prints on the standard output the maximum amount of power that can be consumed in the corresponding network. Each result has an integral value and is printed from the beginning of a separate line.
Sample Input
2 1 1 2 (0,1)20 (1,0)10 (0)15 (1)20
7 2 3 13 (0,0)1 (0,1)2 (0,2)5 (1,0)1 (1,2)8 (2,3)1 (2,4)7
(3,5)2 (3,6)5 (4,2)7 (4,3)5 (4,5)1 (6,0)5
(0)5 (1)2 (3)2 (4)1 (5)4
Sample Output
15 6
Hint
The sample input contains two data sets. The first data set encodes a network with 2 nodes, power station 0 with pmax(0)=15 and consumer 1 with cmax(1)=20, and 2 power transport lines with lmax(0,1)=20 and lmax(1,0)=10. The maximum value of Con is 15. The second data set encodes the network from figure 1.
Source
网络流。
要增加源点和汇点
套模板:
/* POJ 1459 */ #include<stdio.h> #include<string.h> #include<queue> #include<algorithm> #include<iostream> using namespace std; //**************************************************** //最大流模板 //初始化:g[][],start,end //****************************************************** const int MAXN=110; const int INF=0x3fffffff; int g[MAXN][MAXN];//存边的容量,没有边的初始化为0 int path[MAXN],flow[MAXN],start,end; int n;//点的个数,编号0-n.n包括了源点和汇点。 queue<int>q; int bfs() { int i,t; while(!q.empty())q.pop();//把清空队列 memset(path,-1,sizeof(path));//每次搜索前都把路径初始化成-1 path[start]=0; flow[start]=INF;//源点可以有无穷的流流进 q.push(start); while(!q.empty()) { t=q.front(); q.pop(); if(t==end)break; //枚举所有的点,如果点的编号起始点有变化可以改这里 for(i=0;i<=n;i++) { if(i!=start&&path[i]==-1&&g[t][i]) { flow[i]=flow[t]<g[t][i]?flow[t]:g[t][i]; q.push(i); path[i]=t; } } } if(path[end]==-1)return -1;//即找不到汇点上去了。找不到增广路径了 return flow[end]; } int Edmonds_Karp() { int max_flow=0; int step,now,pre; while((step=bfs())!=-1) { max_flow+=step; now=end; while(now!=start) { pre=path[now]; g[pre][now]-=step; g[now][pre]+=step; now=pre; } } return max_flow; } int main()//多源多汇点,在前面加个源点,后面加个汇点,转成单源单汇点 { //freopen("in.txt","r",stdin); //freopen("out.txt","w",stdout); int np,nc,m; int u,v,z; while(scanf("%d%d%d%d",&n,&np,&nc,&m)!=EOF) { memset(g,0,sizeof(g)); while(m--) { while(getchar()!='('); scanf("%d,%d)%d",&u,&v,&z); u++;v++; g[u][v]=z; } while(np--) { while(getchar()!='('); scanf("%d)%d",&u,&z); u++; g[0][u]=z; } while(nc--) { while(getchar()!='('); scanf("%d)%d",&u,&z); u++; g[u][n+1]=z; } n++; start=0; end=n; printf("%d\n",Edmonds_Karp()); } return 0; }