hdu 2215 & hdu 3932(最小覆盖圆)

Maple trees

Time Limit: 1000/1000 MS (Java/Others)    Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 1847    Accepted Submission(s): 574

Problem Description

There are a lot of trees in HDU. Kiki want to surround all the trees with the minimal required length of the rope . As follow,

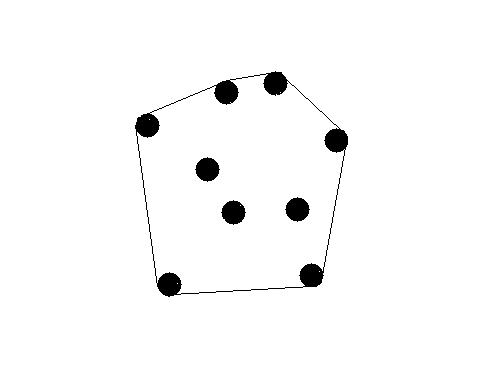
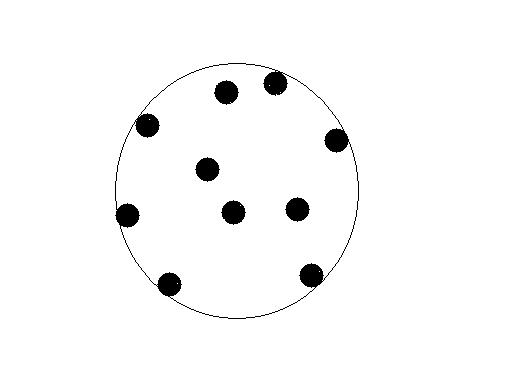
To make this problem more simple, consider all the trees are circles in a plate. The diameter of all the trees are the same (the diameter of a tree is 1 unit). Kiki can calculate the minimal length of the rope , because it's so easy for this smart girl.
But we don't have a rope to surround the trees. Instead, we only have some circle rings of different radius. Now I want to know the minimal required radius of the circle ring. And I don't want to ask her this problem, because she is busy preparing for the examination.
As a smart ACMer, can you help me ?

 

Input

The input contains one or more data sets. At first line of each input data set is number of trees in this data set n （1 <= n <= 100）, it is followed by n coordinates of the trees. Each coordinate is a pair of integers, and each integer is in [-1000, 1000], it means the position of a tree’s center. Each pair is separated by blank.
Zero at line for number of trees terminates the input for your program.

 

Output

Minimal required radius of the circle ring I have to choose. The precision should be 10^-2.

 

Sample Input

2 1 0 -1 0 0

 

Sample Output

1.50

 

题意：求将所有树包括进来的最小圆的半径,最后要加上树本身的半径(树的直径为1).

最小圆覆盖问题：
一.概念引入
最小包围圆问题：对于给定的平面上甩个点所组成的一个集合P，求出P的最小包围圆，即包含P中所有点、半径最小
的那个圆。也就是求出这个最小包围圆的圆心位置和半径。

下面是若干性质。

1.有限点集P的最小包围圆是唯一的。这里约定，若P中只有一个点v，则最小包围圆是退化的，其半径为0，圆心为点v。
2.非退化最小包围圆可以由2个或者3个边界点定义。边界上只有两个点，则必定是直径两端，其它点都在圆内部。
3.点集P中，距离最大的2个点A、B不一定都在边界上，但是必有d≥|AB|。
4.直角三角形或钝角三角形的3个顶点的最小包围圆是以最长边为直径的圆；锐角三角形3个顶点的最小包围圆是三角形
的外接圆。
5.新加入点一定在圆上。
以上资料参考:http://www.cnblogs.com/hxsyl/p/3226562.html

 hdu 2215

///最小圆覆盖问题：
/**一.概念引入
最小包围圆问题：对于给定的平面上甩个点所组成的一个集合P，求出P的最小包围圆，即包含P中所有点、半径最小
的那个圆。也就是求出这个最小包围圆的圆心位置和半径。

下面是若干性质。

1.有限点集P的最小包围圆是唯一的。这里约定，若P中只有一个点v，则最小包围圆是退化的，其半径为0，圆心为点v。
2.非退化最小包围圆可以由2个或者3个边界点定义。边界上只有两个点，则必定是直径两端，其它点都在圆内部。
3.点集P中，距离最大的2个点A、B不一定都在边界上，但是必有d≥|AB|。
4.直角三角形或钝角三角形的3个顶点的最小包围圆是以最长边为直径的圆；锐角三角形3个顶点的最小包围圆是三角形
的外接圆。
5.新加入点一定在圆上。
*/
#include <iostream>
#include <cstdio>
#include <string.h>
#include <math.h>
#include <algorithm>
using namespace std;
const int N = 105;
const double eps = 1e-8;

struct Point{
    double x,y;
}p[N];

Point c; ///这是最小圆的圆心
double r; ///这是最小圆半径
int n;

double dis(Point a,Point b){
    return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));
}
/**计算外接圆的圆心*/
Point circumcenter(Point a,Point b,Point c){
    Point ret=a;
    double a1 = (b.x-a.x),b1=(b.y-a.y),c1 = (a1*a1+b1*b1)/2;
    double a2 = (c.x-a.x),b2=(c.y-a.y),c2 = (a2*a2+b2*b2)/2;
    double d=a1*b2-a2*b1;
    ret.x+=(c1*b2-c2*b1)/d;
    ret.y+=(a1*c2-a2*c1)/d;
    return ret;

}
void min_cover_circle(){
    //random_shuffle(p,p+n); ///模板上面的这个函数是打乱点，这个题不要比要还快15MS
    c = p[0],r = 0;
    for(int i=1;i<n;i++){ ///i是第一个点
        if(dis(p[i],c)-r>eps){
            c = p[i],r=0;
            for(int j=0;j<i;j++){ ///j是第二个点
                if(dis(p[j],c)-r>eps){
                   c.x = (p[i].x+p[j].x)/2;
                   c.y = (p[i].y+p[j].y)/2;
                   r = dis(p[j],c);
                   for(int k=0;k<j;k++){ ///k是第三个点
                        if(dis(p[k],c)-r>eps){
                            c = circumcenter(p[i],p[j],p[k]);
                            r = dis(p[i],c);
                        }
                   }
                }
            }
        }
    }
}
int main()
{
    while(scanf("%d",&n)!=EOF&&n){
        for(int i=0;i<n;i++){
            scanf("%lf%lf",&p[i].x,&p[i].y);
        }
        min_cover_circle();
        printf("%.2lf
",r+0.5);
    }
    return 0;
}

 hdu 3932

///最小圆覆盖问题：
/**一.概念引入
最小包围圆问题：对于给定的平面上甩个点所组成的一个集合P，求出P的最小包围圆，即包含P中所有点、半径最小
的那个圆。也就是求出这个最小包围圆的圆心位置和半径。

下面是若干性质。

1.有限点集P的最小包围圆是唯一的。这里约定，若P中只有一个点v，则最小包围圆是退化的，其半径为0，圆心为点v。
2.非退化最小包围圆可以由2个或者3个边界点定义。边界上只有两个点，则必定是直径两端，其它点都在圆内部。
3.点集P中，距离最大的2个点A、B不一定都在边界上，但是必有d≥|AB|。
4.直角三角形或钝角三角形的3个顶点的最小包围圆是以最长边为直径的圆；锐角三角形3个顶点的最小包围圆是三角形
的外接圆。
5.新加入点一定在圆上。
*/
#include <iostream>
#include <cstdio>
#include <string.h>
#include <math.h>
#include <algorithm>
using namespace std;
const int N = 1005;
const double eps = 1e-8;

struct Point{
    double x,y;
}p[N];

Point c; ///这是最小圆的圆心
double r; ///这是最小圆半径
int n;

double dis(Point a,Point b){
    return sqrt((a.x-b.x)*(a.x-b.x)+(a.y-b.y)*(a.y-b.y));
}
/**计算外接圆的圆心*/
Point circumcenter(Point a,Point b,Point c){
    Point ret=a;
    double a1 = (b.x-a.x),b1=(b.y-a.y),c1 = (a1*a1+b1*b1)/2;
    double a2 = (c.x-a.x),b2=(c.y-a.y),c2 = (a2*a2+b2*b2)/2;
    double d=a1*b2-a2*b1;
    ret.x+=(c1*b2-c2*b1)/d;
    ret.y+=(a1*c2-a2*c1)/d;
    return ret;

}
void min_cover_circle(){
    //random_shuffle(p,p+n); ///模板上面的这个函数是打乱点，这个题不要比要还快15MS
    c = p[0],r = 0;
    for(int i=1;i<n;i++){ ///i是第一个点
        if(dis(p[i],c)-r>eps){
            c = p[i],r=0;
            for(int j=0;j<i;j++){ ///j是第二个点
                if(dis(p[j],c)-r>eps){
                   c.x = (p[i].x+p[j].x)/2;
                   c.y = (p[i].y+p[j].y)/2;
                   r = dis(p[j],c);
                   for(int k=0;k<j;k++){ ///k是第三个点
                        if(dis(p[k],c)-r>eps){
                            c = circumcenter(p[i],p[j],p[k]);
                            r = dis(p[i],c);
                        }
                   }
                }
            }
        }
    }
}
int main()
{
    int X,Y;
    while(scanf("%d%d%d",&X,&Y,&n)!=EOF){
        for(int i=0;i<n;i++){
            scanf("%lf%lf",&p[i].x,&p[i].y);
        }
        min_cover_circle();
        printf("(%.1lf,%.1lf).
%.1lf
",c.x,c.y,r);
    }
    return 0;
}