题目地址:https://leetcode-cn.com/problems/er-cha-shu-de-shen-du-lcof/
题目描述
输入一棵二叉树的根节点,求该树的深度。从根节点到叶节点依次经过的节点(含根、叶节点)形成树的一条路径,最长路径的长度为树的深度。
题目示例
例如:
给定二叉树 [3,9,20,null,null,15,7],
3
/
9 20
/
15 7
返回它的最大深度 3 。
解题思路
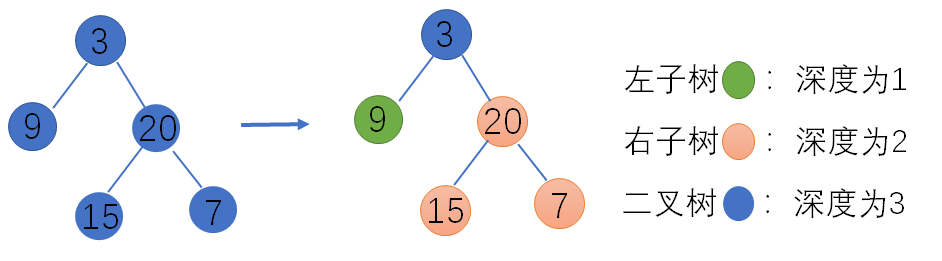
二叉树的深度为根节点到最远叶子节点的最长路径上的节点数
DFS(深度优先搜索-递归):深度优先搜索方法常常采用递归或者栈的方法实现,在这里我们使用递归的方法解决,直接返回树的深度等于左子树和右子树之中的最大值+1,即h = max(maxDepth(root->letf), maxDepth(root->right) + 1。二叉树的深度计算方法如下
- 如果二叉树为一个节点,则二叉树深度h=1
- 如果二叉树没有左子树,则二叉树深度h=右子树深度+1,反之,如果二叉树没有右子树,则二叉树深度h=左子树深度+1
- 如果二叉树既有左子树又有右子树,则则二叉树深度h=max(左子树深度,右子树深度)+1
DFS(深度优先搜索-非递归-栈):中序遍历顺序为”左子树->根节点->右子树“,在这里我们使用中序遍历方法找到二叉树的最大深度,从根节点开始,此时并不需要将根节点入栈,先遍历左子树,将左子树所有元素入栈之后,再将根节点入栈,最后,右子树入栈。
BFS(广度优先搜索):广度优先搜索方法常采用队列来实现,遍历队列中的各节点,并将其左子节点和右子节点分别加入队列。然后,对二叉树一层一层遍历,每遍历一层,深度加1。
程序源码
DFS(递归)
/** * Definition for a binary tree node. * struct TreeNode { * int val; * TreeNode *left; * TreeNode *right; * TreeNode(int x) : val(x), left(NULL), right(NULL) {} * }; */ class Solution { public: int maxDepth(TreeNode* root) { if(root == nullptr) return 0;
return max(maxDepth(root->left), maxDepth(root->right)) + 1; } };
DFS(非递归-栈)
/** * Definition for a binary tree node. * struct TreeNode { * int val; * TreeNode *left; * TreeNode *right; * TreeNode(int x) : val(x), left(NULL), right(NULL) {} * }; */ class Solution { public: int maxDepth(TreeNode* root) { if(root == nullptr) return 0; stack<pair<TreeNode*, int>> s; int deep = 0, cur_deep = 0; TreeNode* p = root; while(!s.empty() || p != nullptr) //若栈非空,则表示还有一些元素的右子树未访问,若p非空,则说明还有一些元素的左子树未访问, { while(p != nullptr) { s.push(pair<TreeNode*, int>(p, ++cur_deep)); //一直往左子树走,直到叶子节点 p = p->left; } p = s.top().first; cur_deep = s.top().second; if(cur_deep > deep) deep = cur_deep; s.pop(); p = p->right; } return deep; } };
BFS(队列)
/**
* Definition for a binary tree node.
* struct TreeNode {
* int val;
* TreeNode *left;
* TreeNode *right;
* TreeNode(int x) : val(x), left(NULL), right(NULL) {}
* };
*/
class Solution {
public:
int maxDepth(TreeNode* root) {
if(root == nullptr) return 0;
queue<TreeNode*> que;
que.push(root);
int depth = 0;
while(!que.empty()) //队列不为空,表示还有元素未访问
{
depth++;
int cur_len = que.size();
for(int i = 0; i < cur_len; i++) //一个for循环表示一层
{
TreeNode* curNode = que.front();
que.pop();
if(curNode->left != nullptr) que.push(curNode->left);
if(curNode->right != nullptr) que.push(curNode->right);
}
}
return depth;
}
};