4827: [Hnoi2017]礼物
Time Limit: 60 Sec Memory Limit: 512 MBSubmit: 727 Solved: 504
[Submit][Status][Discuss]
Description
我的室友最近喜欢上了一个可爱的小女生。马上就要到她的生日了,他决定买一对情侣手 环,一个留给自己,一
个送给她。每个手环上各有 n 个装饰物,并且每个装饰物都有一定的亮度。但是在她生日的前一天,我的室友突
然发现他好像拿错了一个手环,而且已经没时间去更换它了!他只能使用一种特殊的方法,将其中一个手环中所有
装饰物的亮度增加一个相同的自然数 c(即非负整数)。并且由于这个手环是一个圆,可以以任意的角度旋转它,
但是由于上面 装饰物的方向是固定的,所以手环不能翻转。需要在经过亮度改造和旋转之后,使得两个手环的差
异值最小。在将两个手环旋转且装饰物对齐了之后,从对齐的某个位置开始逆时针方向对装饰物编号 1,2,…,n,
其中 n 为每个手环的装饰物个数,第 1 个手环的 i 号位置装饰物亮度为 xi,第 2 个手 环的 i 号位置装饰物
亮度为 yi,两个手环之间的差异值为(参见输入输出样例和样例解释): 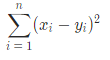 麻烦你帮他
麻烦你帮他
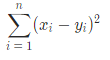 麻烦你帮他
麻烦你帮他计算一下,进行调整(亮度改造和旋转),使得两个手环之间的差异值最小, 这个最小值是多少呢?
Input
输入数据的第一行有两个数n, m,代表每条手环的装饰物的数量为n,每个装饰物的初始 亮度小于等于m。
接下来两行,每行各有n个数,分别代表第一条手环和第二条手环上从某个位置开始逆时 针方向上各装饰物的亮度。
1≤n≤50000, 1≤m≤100, 1≤ai≤m
Output
输出一个数,表示两个手环能产生的最小差异值。
注意在将手环改造之后,装饰物的亮度 可以大于 m。
Sample Input
5 6
1 2 3 4 5
6 3 3 4 5
1 2 3 4 5
6 3 3 4 5
Sample Output
1
【样例解释】
需要将第一个手环的亮度增加1,第一个手环的亮度变为: 2 3 4 5 6 旋转一下第二个手环。对于该样例,是将第
二个手环的亮度6 3 3 4 5向左循环移动 一个位置,使得第二手环的最终的亮度为
:3 3 4 5 6。 此时两个手环的亮度差异值为1。
【样例解释】
需要将第一个手环的亮度增加1,第一个手环的亮度变为: 2 3 4 5 6 旋转一下第二个手环。对于该样例,是将第
二个手环的亮度6 3 3 4 5向左循环移动 一个位置,使得第二手环的最终的亮度为
:3 3 4 5 6。 此时两个手环的亮度差异值为1。
分析:好题!
很容易想到:只需要考虑一个手环亮度的变化. 这样另一个的亮度变化就是相对于当前手环的了,和考虑2个手环亮度的变化是等价的.
题目中给了答案的计算公式,将亮度的变化c带入进式子中,得: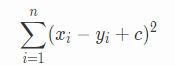 ,展开,可以得到:
,展开,可以得到:
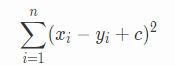 ,展开,可以得到:
,展开,可以得到: . 这种展开方式是处理复杂的带Σ式子的常用方法,即:将结构相同的单独提出来,再对每一项分别求解.
. 这种展开方式是处理复杂的带Σ式子的常用方法,即:将结构相同的单独提出来,再对每一项分别求解. 前两项维护两个前缀和就好了. 后面的有关c的式子实际上是一个二次函数ax^2 + bx. a和b都确定了,x的最值也就确定了. 关键是如何使得2Σxiyi最大.
这显然是不能O(n)出解的. 必须将每一种相对的方式都考虑到才能得到正确的答案. 也就是说,我们需要固定x,每次将y整体向后挪一位,挪n次,算出每次的最大值.
直接算是O(n^2)的,有没有什么快一点的方法呢? 其实它可以用FFT优化到O(nlogn).
看到两项相乘,又带个∑符号,复杂度要求O(nlogn)的,基本上就是FFT了. 如果y向后挪了k位,那么f[k] = Σx[i] * y[i + k]. 这就和bzoj2194是一模一样的了.
需要注意的是:本题中的数是循环的,也就是说a_i + k 可能在a_i之前(转回来了),那么就需要把a数组在其后面复制一份.(破环成链).
#include <cstdio> #include <cstring> #include <iostream> #include <algorithm> #include <cmath> using namespace std; typedef long long ll; const ll maxn = 500010,inf = 1LL << 60; const double pai = acos(-1.0); ll n,m,a[maxn],b[maxn],ans,sum1,sum2,sum11,sum22,pos1,pos2,len; struct node { double real, imag; node(double real = 0.0, double imag = 0.0) { this->real = real, this->imag = imag; } node operator - (const node&elem) const { return node(this->real - elem.real, this->imag - elem.imag); } node operator + (const node&elem) const { return node(this->real + elem.real, this->imag + elem.imag); } node operator * (const node&elem) const { return node(this->real * elem.real - this->imag * elem.imag, this->real * elem.imag + this->imag * elem.real); } void set(double real = 0.0, double imag = 0.0) { this->real = real, this->imag = imag; } } A[maxn],B[maxn]; void Swap(node &a,node &b) { node temp = a; a = b; b = temp; } void zhuan(node *y) { for (ll i = 1,j = len >> 1,k; i < len - 1; i++) { if (i < j) Swap(y[i],y[j]); k = len >> 1; while (j >= k) { j -= k; k >>= 1; } if (j < k) j += k; } } void FFT(node *y,ll op) { zhuan(y); for (ll h = 2; h <= len; h <<= 1) { node temp(cos(op * pai * 2 / h),sin(op * pai * 2 / h)); for (ll i = 0; i < len; i += h) { node W(1,0); for (ll j = i; j < i + h / 2; j++) { node u = y[j]; node v = W * y[j + h / 2]; y[j] = u + v; y[j + h / 2] = u - v; W = W * temp; } } } if (op == -1) for (ll i = 0; i < len; i++) y[i].real /= len; } void solve(node *A,node *B) { FFT(A,1); FFT(B,1); for (ll i = 0; i < len; i++) A[i] = A[i] * B[i]; FFT(A,-1); } void pre() { len = 1; while (len < (n << 1)) len <<= 1; for (ll i = 0; i < n; i++) A[i].set(a[i],0),B[i].set(b[n - i - 1],0),A[i + n].set(a[i],0); for (ll i = n; i < len; i++) B[i].set(); for (ll i = 2 * n; i < len; i++) A[i].set(); } int main() { scanf("%lld%lld",&n,&m); for (ll i = 0; i < n; i++) { scanf("%lld",&a[i]); sum1 += a[i]; sum11 += a[i] * a[i]; } for (ll i = 0; i < n; i++) { scanf("%lld",&b[i]); sum2 += b[i]; sum22 += b[i] * b[i]; } pos1 = floor((double)(sum1 - sum2) / -n),pos2 = ceil((double)(sum1 - sum2) / -n); ans = min(pos1 * pos1 * n + 2 * (sum1 - sum2) * pos1,pos2 * pos2 * n + 2 * (sum1 - sum2) * pos2); ans += sum11 + sum22; pre(); solve(A,B); ll temp = -inf; for (ll i = n - 1; i < 2 * n - 1; i++) { ll tmp = (ll)(A[i].real + 0.5); temp = max(temp,tmp); } ans -= temp * 2; printf("%lld ",ans); return 0; }