Andrew and Jerry are playing a game with Harry as the scorekeeper. The game consists of three rounds. In each round, Andrew and Jerry draw randomly without replacement from a jar containing n balls, each labeled with a distinct positive integer. Without looking, they hand their balls to Harry, who awards the point to the player with the larger number and returns the balls to the jar. The winner of the game is the one who wins at least two of the three rounds.
Andrew wins rounds 1 and 2 while Jerry wins round 3, so Andrew wins the game. However, Jerry is unhappy with this system, claiming that he will often lose the match despite having the higher overall total. What is the probability that the sum of the three balls Jerry drew is strictly higher than the sum of the three balls Andrew drew?
The first line of input contains a single integer n (2 ≤ n ≤ 2000) — the number of balls in the jar.
The second line contains n integers ai (1 ≤ ai ≤ 5000) — the number written on the ith ball. It is guaranteed that no two balls have the same number.
Print a single real value — the probability that Jerry has a higher total, given that Andrew wins the first two rounds and Jerry wins the third. Your answer will be considered correct if its absolute or relative error does not exceed 10 - 6.
Namely: let's assume that your answer is a, and the answer of the jury is b. The checker program will consider your answer correct, if 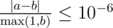 .
.
2
1 2
0.0000000000
3
1 2 10
0.0740740741
In the first case, there are only two balls. In the first two rounds, Andrew must have drawn the 2 and Jerry must have drawn the 1, and vice versa in the final round. Thus, Andrew's sum is 5 and Jerry's sum is 4, so Jerry never has a higher total.
In the second case, each game could've had three outcomes — 10 - 2, 10 - 1, or 2 - 1. Jerry has a higher total if and only if Andrew won 2 - 1 in both of the first two rounds, and Jerry drew the 10 in the last round. This has probability  .
.
题意:每人轮流从n个数中取出一个,然后放回,数大的人获胜,A胜两场,B胜一场,但是B的总和大于A,求这种可能的概率
分析:从小到大排序之后,可以求出进行一次所有的组合,然后可以求出进行两场的组合,然后在暴力求一下比进行一次要少的两次的
#include <iostream> #include <algorithm> #include <cstring> #include <cstdio> using namespace std; typedef long long LL; const int Max = 5000 + 10; int cnt[Max],a[Max]; LL A[Max * 2]; int main() { int n; scanf("%d", &n); for(int i = 1; i <= n; i++) scanf("%d", &a[i]); sort(a + 1, a + n + 1); memset(cnt, 0, sizeof(cnt)); for(int i = 1; i <= n; i++) { for(int j = i - 1; j >= 1; j--) cnt[ a[i] - a[j] ]++; //每种可能的组合个数 } int sum = n * (n - 1) / 2; //从n个数选择2个的总数 memset(A, 0, sizeof(A)); for(int i = 1; i <= 5000; i++) { for(int j = 1; j <= 5000; j++) { A[i + j] += (LL)cnt[i] * (LL)cnt[j]; //两场之后总和为i+j的组合数 } } double ans = 0; for(int i = 1; i <= 5000; i++) //胜一场的 { for(int j = i - 1; j >= 1 ; j--) // 胜两场的总和要比胜一场的小,所以往前找 { ans += 1.0 * cnt[i] / sum * A[j] / sum / sum; //胜一场除以sum,两场除以sum在除以sum } } printf("%.10lf ", ans); return 0; }