POJ 1681 Painter's Problem 高斯消元

Painter's Problem

 

Description

There is a square wall which is made of n*n small square bricks. Some bricks are white while some bricks are yellow. Bob is a painter and he wants to paint all the bricks yellow. But there is something wrong with Bob's brush. Once he uses this brush to paint brick (i, j), the bricks at (i, j), (i-1, j), (i+1, j), (i, j-1) and (i, j+1) all change their color. Your task is to find the minimum number of bricks Bob should paint in order to make all the bricks yellow. 

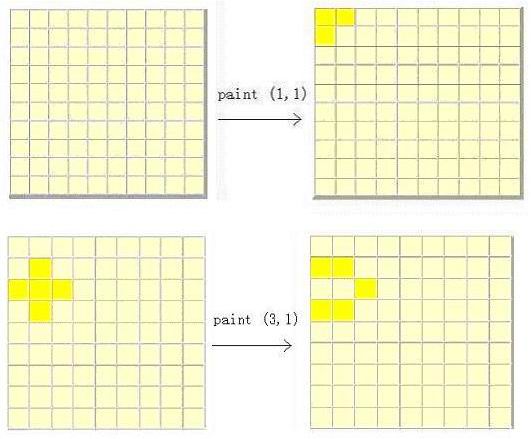
Input

The first line contains a single integer t (1 <= t <= 20) that indicates the number of test cases. Then follow the t cases. Each test case begins with a line contains an integer n (1 <= n <= 15), representing the size of wall. The next n lines represent the original wall. Each line contains n characters. The j-th character of the i-th line figures out the color of brick at position (i, j). We use a 'w' to express a white brick while a 'y' to express a yellow brick.

Output

For each case, output a line contains the minimum number of bricks Bob should paint. If Bob can't paint all the bricks yellow, print 'inf'.

Sample Input

2
3
yyy
yyy
yyy
5
wwwww
wwwww
wwwww
wwwww
wwwww

Sample Output

0
15

题意：

　　给你一个只含'Y‘  或 'W'颜色的图

　　每次你可以选择图上一个点 (i,j)

　　那么 (i,j)及四周的 点的颜色都会改变

　　给出原始图，问你最少次数的选择使得 最终图的颜色全部为 'Y'；

题解：

　　高斯消元n*n个方程

　　只有自己和四周的点的改变会使其变化

#include<iostream>
#include<cstdio>
#include<cmath>
#include<algorithm>
#include<cstring>
using namespace std;
#pragma comment(linker, "/STACK:102400000,102400000")
#define ls i<<1
#define rs ls | 1
#define mid ((ll+rr)>>1)
#define pii pair<int,int>
#define MP make_pair
typedef long long LL;
const long long INF = 1e18;
const double Pi = acos(-1.0);
const int N = 600+10, M = 1e2+11, mod = 1e9+7, inf = 0x3fffffff;

int a[N][N],x[N],free_x[N],n;
int ss[4][2] = {-1,0,0,-1,1,0,0,1};
int Guass(int equ,int var) {
    int i,j,k;
    int max_r;
    int col;
    int ta,tb;
    int LCM;
    int temp;
    int free_index;
    int num=0;
    for(int i=0;i<=var;i++)
    {
        x[i]=0;
        free_x[i]=0;
    }
    col=0;
    for(k = 0;k < equ && col < var;k++,col++)
    {
        max_r=k;
        for(i=k+1;i<equ;i++)
        {
            if(abs(a[i][col])>abs(a[max_r][col])) max_r=i;
        }
        if(max_r!=k)
        {
            for(j=k;j<var+1;j++) swap(a[k][j],a[max_r][j]);
        }
        if(a[k][col]==0)
        {
            k--;
            free_x[num++]=col;
            continue;
        }
        for(i=k+1;i<equ;i++)
        {
            if(a[i][col]!=0)
            {
                for(j=col;j<var+1;j++)
                {
                    a[i][j] ^= a[k][j];
                }
            }
        }
    }
    for (i = k; i < equ; i++)
    {
        if (a[i][col] != 0) return inf;
    }
     for(i=var-1;i>=0;i--)
    {
        x[i]=a[i][var];
        for(j=i+1;j<var;j++)
          x[i]^=(a[i][j]&&x[j]);
    }
    int cnt = 0;
    for(int i = 0; i < n*n; ++i) if(x[i]) cnt++;
    return cnt;
}
char ch[N][N];
int main() {
        int T;
        scanf("%d",&T);
        while(T--) {
            scanf("%d",&n);
            memset(a,0,sizeof(a));
            for(int i = 0; i < n; ++i) scanf("%s",ch[i]);
            for(int i = 0; i < n; ++i) {
                for(int j = 0; j < n; ++j) {
                    a[i*n+j][n*n] = 1^(ch[i][j] == 'y');
                }
            }
            for(int i = 0; i < n; ++i) {
                for(int j = 0; j < n; ++j) {
                    a[i*n+j][i*n+j] = 1;
                    for(int k = 0; k < 4; ++k) {
                        int ii = i + ss[k][0];
                        int jj = j + ss[k][1];
                        if(ii < 0 || jj < 0 || ii >= n ||jj >= n) continue;
                        a[i*n+j][ii*n+jj] = 1;
                    }
                }
            }
            int ans = Guass(n*n,n*n);
            if(ans == inf) puts("inf");
            else
            printf("%d
",ans);
        }
        return 0;
}