Interesting Fibonacci
Time Limit: 2000/1000 MS (Java/Others) Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 1071 Accepted Submission(s): 229
Problem Description
In
mathematics, the Fibonacci numbers are a sequence of numbers named
after Leonardo of Pisa, known as Fibonacci (a contraction of filius
Bonaccio, "son of Bonaccio"). Fibonacci's 1202 book Liber Abaci
introduced the sequence to Western European mathematics, although the
sequence had been previously described in Indian mathematics.
The first number of the sequence is 0, the second number is 1, and each subsequent number is equal to the sum of the previous two numbers of the sequence itself, yielding the sequence 0, 1, 1, 2, 3, 5, 8, etc. In mathematical terms, it is defined by the following recurrence relation:
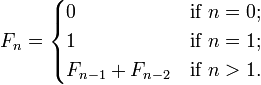
That is, after two starting values, each number is the sum of the two preceding numbers. The first Fibonacci numbers (sequence A000045 in OEIS), also denoted as F[n];
F[n] can be calculate exactly by the following two expressions:
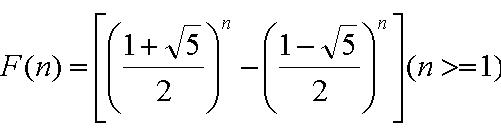
A Fibonacci spiral created by drawing arcs connecting the opposite corners of squares in the Fibonacci tiling; this one uses squares of sizes 1, 1, 2, 3, 5, 8, 13, 21, and 34;
So you can see how interesting the Fibonacci number is.
Now AekdyCoin denote a function G(n)
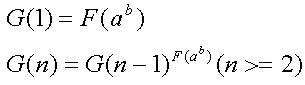
Now your task is quite easy, just help AekdyCoin to calculate the value of G (n) mod C
The first number of the sequence is 0, the second number is 1, and each subsequent number is equal to the sum of the previous two numbers of the sequence itself, yielding the sequence 0, 1, 1, 2, 3, 5, 8, etc. In mathematical terms, it is defined by the following recurrence relation:
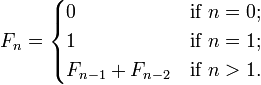
That is, after two starting values, each number is the sum of the two preceding numbers. The first Fibonacci numbers (sequence A000045 in OEIS), also denoted as F[n];
F[n] can be calculate exactly by the following two expressions:
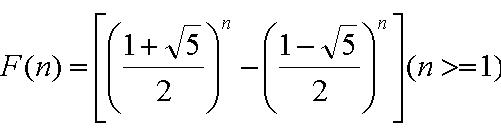
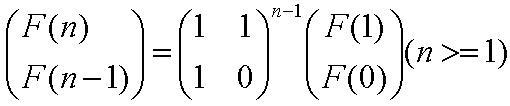
A Fibonacci spiral created by drawing arcs connecting the opposite corners of squares in the Fibonacci tiling; this one uses squares of sizes 1, 1, 2, 3, 5, 8, 13, 21, and 34;
So you can see how interesting the Fibonacci number is.
Now AekdyCoin denote a function G(n)
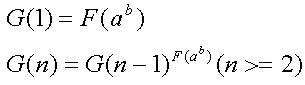
Now your task is quite easy, just help AekdyCoin to calculate the value of G (n) mod C
Input
The
input consists of T test cases. The number of test cases (T is given in
the first line of the input. Each test case begins with a line
containing A, B, N, C (10<=A, B<2^64, 2<=N<2^64,
1<=C<=300)
Output
For
each test case, print a line containing the test case number( beginning
with 1) followed by a integer which is the value of G(N) mod C
Sample Input
1
17 18446744073709551615 1998 139
Sample Output
Case 1: 120
Author
AekdyCoin
思路:欧拉函数;
G(n)= F(a^b)^((F(a^b))^(N-1));然后,找一下数列的循环节,然后应为a^b>300,所以直接用欧拉降幂,((F(a^b)^(N-1))%oula[C] + oula[C]);因为F(a^b)^(N-1) > oula[C];
这样幂数就就降下来了。
1 #include<stdio.h> 2 #include<algorithm> 3 #include<stdlib.h> 4 #include<queue> 5 #include<iostream> 6 #include<string.h> 7 #include<math.h> 8 using namespace std; 9 typedef unsigned long long LL; 10 bool prime[400]; 11 int ans[400]; 12 int oula[400]; 13 int ff[30]; 14 typedef struct node 15 { 16 LL m[2][2]; 17 node() 18 { 19 memset(m,0,sizeof(m)); 20 } 21 } maxtr; 22 int f[10000]; 23 int fin(LL n); 24 LL quick(LL n,LL m,LL mod); 25 int main(void) 26 { 27 memset(prime,0,sizeof(prime)); 28 int i,j; 29 for(i = 0; i <= 300; i++) 30 { 31 oula[i] = i; 32 } 33 int cn = 0; 34 for(i = 2; i <= 300; i++) 35 { 36 if(!prime[i]) 37 { 38 ans[cn++] = i; 39 for(j = i; (i*j) <= 300; j++) 40 { 41 prime[i*j] = true; 42 } 43 } 44 }//printf("%d ",cn); 45 for(i = 0; i < cn; i++) 46 { 47 for(j = 1; ans[i]*j <= 300; j++) 48 { 49 oula[ans[i]*j]/=ans[i]; 50 oula[ans[i]*j]*=(ans[i] - 1); 51 } 52 } 53 ff[0] = 0; 54 ff[1] = 1; 55 for(i = 2; i <= 20; i++) 56 { 57 ff[i] = ff[i-1]+ff[i-2]; 58 } 59 //printf("%d ",ff[20]); 60 LL A,B,N,C; 61 int T; 62 scanf("%d",&T); 63 int __ca = 0; 64 while(T--) 65 { 66 scanf("%llu %llu %llu %llu",&A,&B,&N,&C); 67 { 68 printf("Case %d: ",++__ca); 69 if(C == 1) 70 printf("0 "); 71 else 72 { 73 int k = fin(C); 74 LL ask = quick(A,B,(LL)k); 75 LL c = (LL)f[ask]; 76 if(c == 0) 77 printf("0 "); 78 else 79 { 80 LL v = A; 81 LL x = B; 82 int flag = 0; 83 { 84 int u = fin((LL)oula[C]); 85 LL avk = quick(A,B,(LL)u); 86 LL app = (LL)f[avk]; 87 LL ni = quick(app,N-1,(LL)oula[C]); 88 ni = ni + (LL)oula[C]; 89 printf("%llu ",quick(c,ni,C)); 90 } 91 } 92 } 93 } 94 } 95 return 0; 96 } 97 int fin(LL n) 98 { 99 f[0] = 0; 100 f[1] = 1; 101 int id; 102 int i; 103 for(i = 2; i < 5000; i++) 104 { 105 f[i] = f[i-1]+f[i-2]; 106 f[i]%=n; 107 if(f[i] == f[1]&&f[0] == f[i-1]) 108 { 109 id = i-2; 110 break; 111 } 112 }//printf("%d ",id); 113 return id+1; 114 } 115 LL quick(LL n,LL m,LL mod) 116 { 117 LL ak = 1; 118 n%=mod; 119 while(m) 120 { 121 if(m&1) 122 { 123 ak = ak*n%mod; 124 } 125 n = n*n%mod; 126 m/=2; 127 } 128 return ak; 129 }