Leetcode 5——Median of Two Sorted Arrays

题目：

There are two sorted arrays nums1 and nums2 of size m and n respectively.

Find the median of the two sorted arrays. The overall run time complexity should be O(log (m+n)).

Example 1:
nums1 = [1, 3]
nums2 = [2]

The median is 2.0
Example 2:
nums1 = [1, 2]
nums2 = [3, 4]

The median is (2 + 3)/2 = 2.5

　　即找两个有序数组的中位数，一开始想的是用第三个数组来把前面两个数组的值依次放进去，再直接找中间的数，但是对时间复杂度的要求是O(log (m+n))，所以不能用数组存。网上找的方法是比较两个数组中间的元素，如AB两个数组，如果A[mid]>B[mid]，那中位数肯定就不在B的前半段，于是缩小了范围，即B后半段加上A，然后依次查找并缩小范围，直到找到中间的数为止。

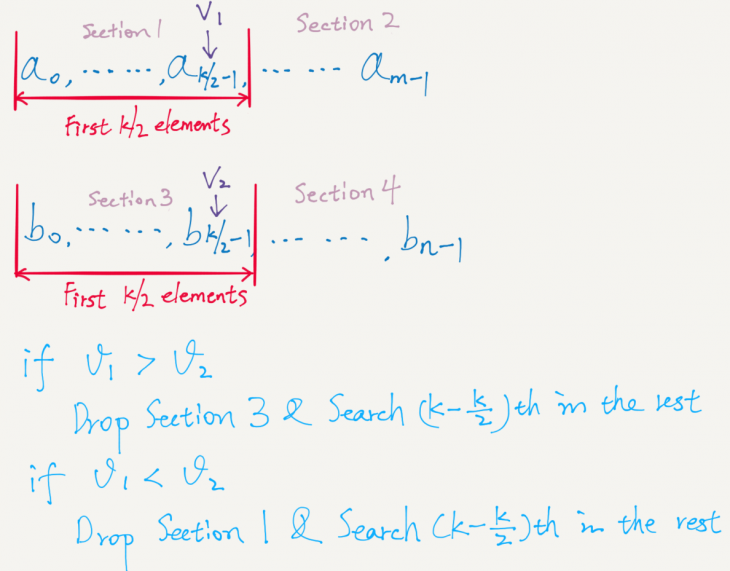
public class Solution {
    public double findMedianSortedArrays(int[] nums1, int[] nums2) {
        
        int m=nums1.length;
        int n=nums2.length;
        if((m+n)%2==0)
            return (findKMax((m+n)/2,nums1,0,nums2,0)+findKMax((m+n)/2+1,nums1,0,nums2,0))/2.0;//如果是偶数
        else 
            return findKMax((m+n)/2+1,nums1,0,nums2,0);//如果是奇数
    }
    public int findKMax(int k,int[] nums1,int start1,int[] nums2,int start2){
        if(start1>=nums1.length)//第一个数组长度为0的话，直接返回第二个数组的中位数
            return nums2[start2+k-1];
            
        if(start2>=nums2.length)//第二个数组长度为0的话，直接返回第一个数组的中位数
            return nums1[start1+k-1];
        
        if(k==1)//k=1，即找第一个，也就是nums1或nums2中最小的
            return Math.min(nums1[start1], nums2[start2]);
            
        int temp1=start1+k/2-1;
        int temp2=start2+k/2-1;
        
        int mid1=temp1<nums1.length?nums1[temp1]:Integer.MAX_VALUE;//nums1没有下标为temp1的元素，如果有，就用那个元素比较，没有就用最大int
        int mid2=temp2<nums2.length?nums2[temp2]:Integer.MAX_VALUE;

        if(mid1>=mid2)
            return findKMax(k-k/2,nums1,start1,nums2,temp2+1);//如果mid1大，也就是nums1中间的数大，那么nums1前半段不会有中位数
        else                                                  //从nums1后半段和nums1开始找
            return findKMax(k-k/2,nums1,temp1+1,nums2,start2);
            
        
    }
    
    
}