HDU 6333:Harvest of Apples

Problem B. Harvest of Apples

Time Limit: 4000/2000 MS (Java/Others)    Memory Limit: 262144/262144 K (Java/Others)
Total Submission(s): 347    Accepted Submission(s): 121

Problem Description

There are $n$ apples on a tree, numbered from $1$ to $n$.
Count the number of ways to pick at most $m$ apples.

 

Input

The first line of the input contains an integer $T$ $(1 le T le 10 ^ 5)$ denoting the number of test cases.
Each test case consists of one line with two integers $n, m$ $(1 le m le n le 10 ^ 5)$.

 

Output

For each test case, print an integer representing the number of ways modulo $10 ^ 9 + 7$.

 

Sample Input

2 5 2 1000 500

 

Sample Output

16 924129523

 

Source

2018 Multi-University Training Contest 4

 

Recommend

chendu

 

分析：这题显然暴力O(n^2)是要TLE的，比赛的时候考虑简单数学优化一下变成2.5e9，显然肯定也是要TLE的。后来考虑深度数学优化得出：

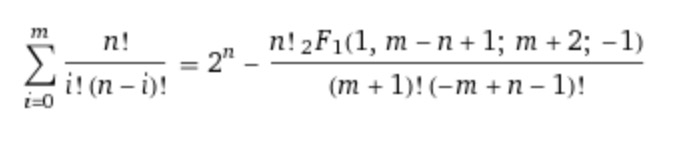
其中F1表示超几何函数。。。然而找了半个多小时没有找到C++的模板。。就很绝望

补题的时候发现可以用莫队和分块去搞，学了半天嘤嘤嘤，太菜了。

#include <iostream>
#include <string>
#include <cstdio>
#include <cmath>
#include <cstring>
#include <algorithm>
#include <vector>
#include <queue>
#include <deque>
#include <stack>
#include <map>
#define LL long long
#define elif else if
#define range(i,a,b) for(auto i=a;i<=b;++i)
#define rerange(i,a,b) for(auto i=a;i>=b;--i)
#define itrange(i,a,b) for(auto i=a;i!=b;++i)
#define fill(arr,tmp) memset(arr,tmp,sizeof(arr))
using namespace std;
int t,n,m,block[int(2e5)],interval,id=1;
struct query{
    int n,k,i;
}Q[int(1e5+5)];
vector<query>ll[int(1e5+5)];
bool cmp(query a,query b){
    return a.n<b.n;
}
const int mod=int(1e9+7);
LL fac[int(1e5+5)],inv[int(1e5+5)],ans[int(1e5+5)];
LL q_pow(LL a,LL b){
    if(b<0)return 0;
    LL ret=1;
    a%=mod;
    while(b){
        ret=b&1?ret*a%mod:ret;
        b>>=1;
        a=a*a%mod;
    }
    return ret;
}
LL C(LL n,LL m){
    if(n<m)return 0;
    return fac[n]*inv[m]%mod*inv[n-m]%mod;
}
void init(){
    fac[0]=1;
    range(i,1,int(1e5))fac[i]=fac[i-1]*i%mod;
    inv[int(1e5)]=q_pow(fac[int(1e5)],mod-2);
    rerange(i,int(1e5-1),0)inv[i]=inv[i+1]*(i+1)%mod;
    interval=int(sqrt(1e5));
    for(int i=1;i<=int(1e5);i+=interval,++id)
        for(int j=i;j<i+interval and j<=int(1e5);++j)block[j]=id;
    --id;
    scanf("%d",&t);
    range(i,1,t){
        scanf("%d%d",&(Q+i)->n,&(Q+i)->k);
        (Q+i)->i=i;
        ll[block[Q[i].k]].push_back(Q[i]);
    }
}
void solve(){
    range(i,1,id)
        if(ll[i].size()){
            sort(ll[i].begin(),ll[i].end(),cmp);
            LL val=0,in=ll[i][0].n,ik=-1;
            range(j,0,ll[i].size()-1){
                while(in<ll[i][j].n)val=((val<<1)+mod-C(in++,ik))%mod;
                while(ik<ll[i][j].k)val=(val+C(in,++ik))%mod;
                while(ik>ll[i][j].k)val=(val+mod-C(in,ik--))%mod;
                ans[ll[i][j].i]=val;
            }
        }
    range(i,1,t)printf("%lld
",ans[i]);
}
int main() {
    init();
    solve();
    return 0;
}