2021.2.7 刷题（路径总和）

1.路径总和（1）
题目链接：https://leetcode-cn.com/problems/path-sum
题目描述：
给你二叉树的根节点 root 和一个表示目标和的整数 targetSum ，判断该树中是否存在 根节点到叶子节点 的路径，这条路径上所有节点值相加等于目标和 targetSum 。
叶子节点 是指没有子节点的节点。


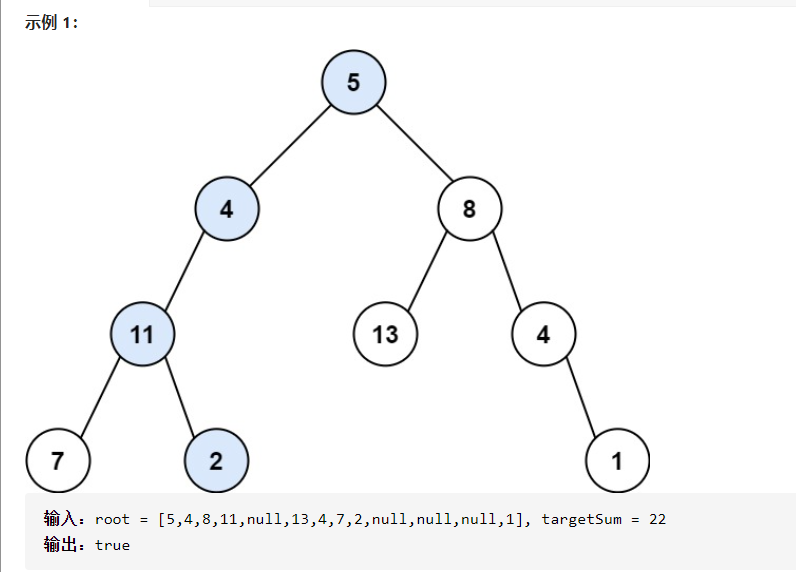
题解：
采用DFS遍历路径，使用递归方式。递归函数的参数和返回值的确定：如果需要搜索整颗二叉树，那么递归函数就不要返回值，如果要搜索其中一条符合条件的路径，递归函数就需要返回值，因为遇到符合条件的路径了就要及时返回。

题解：

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
public:
    bool travel(TreeNode* node, int count)
    {
        if(node->left == nullptr && node->right == nullptr && count == 0) //节点为叶子节点，且总和值相等
            return true;
        if(node->left)
        {
            count -= node->left->val; //递归，处理节点
            if(travel(node->left, count)) return true;
            count += node->left->val;  //回溯，撤销先前的操作
        }
        if(node->right)
        {
            count -= node->right->val;
            if(travel(node->right, count)) return true;
            count -= node->right->val; 
        }
        return false;
    }
    
    bool hasPathSum(TreeNode* root, int targetSum) {
        if(root == nullptr)
            return false;

        return travel(root, targetSum - root->val);
    }
};

2.全部路径（2）
题目链接：https://leetcode-cn.com/problems/path-sum-ii/
题目描述：

题解：
由上一题的练习，这一题的递归函数的返回值为void，参数与上一题相同，代码逻辑与上一题也基本相同，只是当遍历到一条路径后需要存放在vector中。

/**
 * Definition for a binary tree node.
 * struct TreeNode {
 *     int val;
 *     TreeNode *left;
 *     TreeNode *right;
 *     TreeNode() : val(0), left(nullptr), right(nullptr) {}
 *     TreeNode(int x) : val(x), left(nullptr), right(nullptr) {}
 *     TreeNode(int x, TreeNode *left, TreeNode *right) : val(x), left(left), right(right) {}
 * };
 */
class Solution {
    vector<vector<int>> result;
    vector<int> vec;
public:
    void travel(TreeNode* node, int count)
    {
        if(node->left == nullptr && node->right == nullptr && count == 0)
        {
            result.push_back(vec);            //找到一条符合要求的路径，存入结果vector中
        }
        if(node->left)
        {
            vec.push_back(node->left->val); //迭代，节点操作
            count -= node->left->val;       //迭代，节点操作
            travel(node->left, count);
            count += node->left->val;      //回溯，撤销节点操作
            vec.pop_back();                 //回溯，撤销节点操作
        }
        if(node->right)
        {
            vec.push_back(node->right->val);
            count -= node->right->val;
            travel(node->right, count);
            count += node->right->val;
            vec.pop_back();
        }
    }
    vector<vector<int>> pathSum(TreeNode* root, int targetSum) 
    {
        if(root == nullptr)
            return result;
        vec.push_back(root->val);
        travel(root, targetSum - (root->val));
        return result;
    }
};