Power Network（网络流最大流 & dinic算法 + 优化）

Power Network

Time Limit: 2000MS		Memory Limit: 32768K
Total Submissions: 24019		Accepted: 12540

Description

A power network consists of nodes (power stations, consumers and dispatchers) connected by power transport lines. A node u may be supplied with an amount s(u) >= 0 of power, may produce an amount 0 <= p(u) <= p_max(u) of power, may consume an amount 0 <= c(u) <= min(s(u),c_max(u)) of power, and may deliver an amount d(u)=s(u)+p(u)-c(u) of power. The following restrictions apply: c(u)=0 for any power station, p(u)=0 for any consumer, and p(u)=c(u)=0 for any dispatcher. There is at most one power transport line (u,v) from a node u to a node v in the net; it transports an amount 0 <= l(u,v) <= l_max(u,v) of power delivered by u to v. Let Con=Σ_uc(u) be the power consumed in the net. The problem is to compute the maximum value of Con. 

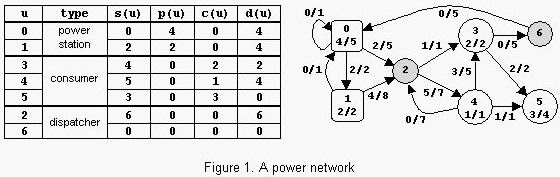
An example is in figure 1. The label x/y of power station u shows that p(u)=x and p_max(u)=y. The label x/y of consumer u shows that c(u)=x and c_max(u)=y. The label x/y of power transport line (u,v) shows that l(u,v)=x and l_max(u,v)=y. The power consumed is Con=6. Notice that there are other possible states of the network but the value of Con cannot exceed 6. 

Input

There are several data sets in the input. Each data set encodes a power network. It starts with four integers: 0 <= n <= 100 (nodes), 0 <= np <= n (power stations), 0 <= nc <= n (consumers), and 0 <= m <= n^2 (power transport lines). Follow m data triplets (u,v)z, where u and v are node identifiers (starting from 0) and 0 <= z <= 1000 is the value of l_max(u,v). Follow np doublets (u)z, where u is the identifier of a power station and 0 <= z <= 10000 is the value of p_max(u). The data set ends with nc doublets (u)z, where u is the identifier of a consumer and 0 <= z <= 10000 is the value of c_max(u). All input numbers are integers. Except the (u,v)z triplets and the (u)z doublets, which do not contain white spaces, white spaces can occur freely in input. Input data terminate with an end of file and are correct.

Output

For each data set from the input, the program prints on the standard output the maximum amount of power that can be consumed in the corresponding network. Each result has an integral value and is printed from the beginning of a separate line.

Sample Input

2 1 1 2 (0,1)20 (1,0)10 (0)15 (1)20
7 2 3 13 (0,0)1 (0,1)2 (0,2)5 (1,0)1 (1,2)8 (2,3)1 (2,4)7
         (3,5)2 (3,6)5 (4,2)7 (4,3)5 (4,5)1 (6,0)5
         (0)5 (1)2 (3)2 (4)1 (5)4

Sample Output

15
6

Hint

The sample input contains two data sets. The first data set encodes a network with 2 nodes, power station 0 with pmax(0)=15 and consumer 1 with cmax(1)=20, and 2 power transport lines with lmax(0,1)=20 and lmax(1,0)=10. The maximum value of Con is 15. The second data set encodes the network from figure 1.

Source

Southeastern Europe 2003

dinic算法：79ms ：http://www.cnblogs.com/jackge/archive/2013/04/14/3019811.html

Push-Relabel算法 157ms :

#include<iostream>
#include<stdio.h>
#include<string.h>
using namespace std;
#define MAXN 202
const int INT_MAX = 0x3f3f3f3f ;
int s, t;
int n, np, nc, m;
char str[50];
int c[MAXN][MAXN];
int f[MAXN][MAXN];
int e[MAXN];
int h[MAXN];
void push(int u, int v)
{
    int d = min(e[u], c[u][v] - f[u][v]);
    f[u][v] += d;
    f[v][u] = -f[u][v];
    e[u] -= d;
    e[v] += d;
}
bool relabel(int u)
{
    int mh = INT_MAX;
    for(int i=0; i<n+2; i++)
    {
        if(c[u][i] > f[u][i])
            mh = min(mh, h[i]);
    }
    if(mh == INT_MAX)
        return false; //残留网络中无从u出发的路
    h[u] = mh + 1;
    for(int i=0; i<n+2; i++)
    {
        if(e[u] == 0) //已无余流，不需再次push
            break;
        if(h[i] == mh && c[u][i] > f[u][i]) //push的条件
            push(u, i);
    }
    return true;
}
void init_preflow()
{
    memset(h, 0, sizeof(h));
    memset(e, 0, sizeof(e));
    h[s] = n+2;
    for(int i=0; i<n+2; i++)
    {
        if(c[s][i] == 0)
            continue;
        f[s][i] = c[s][i];
        f[i][s] = -f[s][i];
        e[i] = c[s][i];
        e[s] -= c[s][i];
    }
}
void push_relabel()
{
    init_preflow();
    bool flag = true; //表示是否还有relabel操作
    while(flag)
    {
        flag = false;
        for(int i=0; i<n; i++)
            if(e[i] > 0)
                flag = flag || relabel(i);
    }
}
int main()
{
    while(scanf("%d%d%d%d", &n, &np, &nc, &m) != EOF)
    {
        s = n; t = n+1;
        memset(c, 0, sizeof(c));
        memset(f, 0, sizeof(f));
        while(m--)
        {
            scanf("%s", &str);
            int u=0, v=0, z=0;
            sscanf(str, "(%d,%d)%d", &u, &v, &z);
            c[u][v] = z;
        }
        for(int i=0; i<np+nc; i++)
        {
            scanf("%s", &str);
            int u=0, z=0;
            sscanf(str, "(%d)%d", &u, &z);
            if(i < np)
                c[s][u] = z;
            else if(i >= np && i < np + nc)
                c[u][t] = z;
        }
        push_relabel();
        printf("%d
", e[t]);
    }
}

dinic递归 110ms / 非递归 110 或 94 ms：http://hefeijack.iteye.com/blog/1885944

dinic 63ms/SAP 63 ms:http://www.cnblogs.com/kuangbin/archive/2012/09/11/2680908.html 

dinic 63ms :http://blog.csdn.net/u011721440/article/details/38611197

初学者可以先去poj1273试试水=。=

这道题只要加一个 源点 和 汇点 ， 就万事大吉了。

自己写了一遍，用dinic的递归形式，一开始tle，但就加了一句话，813msAC，

貌似是一种优化，但为啥不清楚。

#include<stdio.h>
#include<string.h>
#include<queue>
#include<algorithm>
using namespace std;
const int M = 210 , inf = 0x3f3f3f3f ;
int m , n ;
int map[M][M] ;
int dis[M];

bool bfs ()
{
    queue <int> q ;
    while (!q.empty ())
        q.pop () ;
    memset (dis , 0xff , sizeof(dis)) ;
    dis[1] = 0 ;
    q.push (1) ;
    while (!q.empty () ) {
        int u = q.front () ;
        q.pop () ;
        for (int v = 1 ; v <= n ; v++) {
            if (map[u][v] && dis[v] == -1) {
                dis[v] = dis[u] + 1 ;
                q.push (v) ;
            }
        }
    }
    if (dis[n] > 0)
        return true ;
    return false ;
}

int find (int u , int low)
{
    int a = 0 ;
    if (u == n)
        return low ;
    for (int v = 1 ; v <= n ; v++) {
        if (map[u][v] && dis[v] == dis[u] + 1 && (a = find (v , min(map[u][v] , low)))) {
            map[u][v] -= a ;
            map[v][u] += a ;
            return a ;
        }
    }
    dis[u] = -1 ;//就这句
    return 0 ;
}

int main ()
{
    //freopen ("b.txt" , "r" , stdin) ;
    int u , v , w ;
    int ans ;
    while (~ scanf ("%d%d" , &m , &n)) {
        memset (map , 0 , sizeof(map)) ;
        while (m--) {
            scanf ("%d%d%d" , &u , &v , &w) ;
            map[u][v] += w ;
        }
        int maxn = 0 ;
        while (bfs()) {
            if (ans = find(1 , inf))
                maxn += ans ;
        }
        printf ("%d
" , maxn) ;
    }
    return 0 ;
}

 用邻接表可以进一步优化（但上面那句话仍要加）：因为用矩阵的话在find 和 bfs 时找点都要遍历一遍所有的点，但用邻接表的话可以很快找到。

110msAC：

#include<stdio.h>
#include<string.h>
#include<queue>
#include<algorithm>
using namespace std;
const int M = 110 , inf = 0x3f3f3f3f ;
struct edge
{
    int u , v , time_u ;
    int w ;
}e[M * M * 2];
int n , np , nc , m ;
int src , des ;
int cnt = 0 ;
int dis[M] ;
char st[200] ;
int head[M * M * 2] ;

void addedge (int u , int v , int w)
{
    e[cnt].u = u ; e[cnt].v = v ; e[cnt].w = w ; e[cnt].time_u = head[u] ;
    head[u] = cnt++ ;
    e[cnt].v = u ; e[cnt].u = v ; e[cnt].w = 0 ; e[cnt].time_u = head[v] ;
    head[v] = cnt++ ;
}
bool bfs ()
{
    queue <int> q ;
    while (!q.empty ())
        q.pop () ;
    memset (dis , -1 , sizeof(dis)) ;
    dis[src] = 0 ;
    q.push (src) ;
    while (!q.empty ()) {
        int u = q.front () ;
        q.pop () ;
        for (int i = head[u] ; i != -1 ; i = e[i].time_u) {
            int v = e[i].v ;
            if (dis[v] == -1 && e[i].w > 0) {
                dis[v] = dis[u] + 1 ;
                q.push (v) ;
            }
        }
    }
    if (dis[des] > 0)
        return true ;
    return false ;
}

int find (int u , int low)
{
    int a = 0 ;
    if (u == des)
        return low ;
    for (int i = head[u] ; i != -1 ; i = e[i].time_u) {
        int v = e[i].v ;
        if (e[i].w > 0 && dis[v] == dis[u] + 1 && (a = find(v , min (low , e[i].w)))) {
            e[i].w -= a ;
            e[i^1].w += a ;
            return a ;
        }
    }
    dis[u] = -1  ;
    return false ;
}

int main ()
{
   // freopen ("a.txt" , "r" , stdin) ;
    int u , v , w ;
    while (~ scanf ("%d%d%d%d" , &n , &np , &nc , &m)) {
        src = n ;
        des = n + 1 ;
        cnt = 0 ;
        memset (head , -1 , sizeof(head)) ;
        while (m--) {
            scanf ("%s" , st) ;
            sscanf (st , "(%d,%d)%d" , &u , &v , &w) ;
            addedge(u , v , w) ;
        }
        while (np--) {
            scanf ("%s" , st) ;
            sscanf (st , "(%d)%d" , &v , &w) ;
            addedge (src , v , w) ;
        }
        while (nc--) {
            scanf ("%s" , st) ;
            sscanf (st , "(%d)%d" , &u , &w) ;
            addedge (u , des , w) ;
        }

        int ans = 0 , res = 0 ;
        while (bfs()) {
            while (1) {
                if (ans = find(src , inf))
                    res += ans ;
                else
                    break ;
            }
        }
        printf ("%d
" , res) ;
    }
    return 0 ;
}

head[u]用来存放点u最新出现的时间 ，感觉和tarjan算法中的dfn[u]差不多；

time_u则保存上一次点u出现的时间 。