POJ 1741 Tree

http://poj.org/problem?id=1741

题目

给一个有N个节点的树，每条边都有一个长度。两个节点之间的路径长度就是树上最短路径长度。问长度不超过k的路径一共有多少条。

$Nleqslant 10000$

题解

树上分治，以p为根的树中路径有两种

1. 

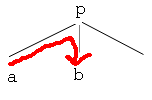
    从一个子树沿着向上，然后朝下进入另外一个子树

2. 

    在p的同一个子树里面

可以看出来，第二种情况仍然会经过一个根

所以考虑第一种情况，然后递归考虑其他根的情况（转化为情况1）就可以了。

题目要求路径长度不超过k，不能直接枚举（时间复杂度$n^2 imes n$，远远超过$10^9$），但是可以在遍历子树的时候寻找其他子树中剩余长度不超过$k-s$的节点数（不好写）

还可以把节点排序，类似于双指针，当l增大时，r一定不会增大，并且保证$l<r$，同时统计l到r中剩余的每个子树节点的个数

时间复杂度$mathcal{O}(R imes (n imes log n+n))$，如果只有一条链，那么$R=mathcal{O}(n)$，仍然会超时，因此需要每次递归都选择重心作为根，那么$R=mathcal{O}(log n)$

时间复杂度$mathcal{O}(n imes log^2 n)$

代码能力下降……又写了一下午

AC代码

#include<cstdio>
#include<cstring>
#include<algorithm>
#include<queue>
#define REP(i,a,b) for(register int i=(a); i<(b); i++)
#define REPE(i,a,b) for(register int i=(a); i<=(b); i++)
using namespace std;
#define MAXN 10007
int n,k;
int vis[MAXN];
int sz[MAXN];
int hd[MAXN], to[MAXN<<1], nxt[MAXN<<1], le[MAXN<<1], es;
inline void init() {
	es=0; memset(vis,0,sizeof vis); memset(hd,-1,sizeof(hd));
	memset(sz,0,sizeof sz);
}
inline void adde(int a, int b, int c) {
	nxt[es]=hd[a];hd[a]=es;to[es]=b;le[es]=c;es++;
	nxt[es]=hd[b];hd[b]=es;to[es]=a;le[es]=c;es++;
}
//FINDS///////////////////////////////////////////////////
int zd, hp, S;
void dfsm(int p) {
	vis[p]=1;
	int s=1, mp=0;
	for(int i=hd[p]; ~i; i=nxt[i]) if((!(vis[to[i]]&1)) && (!(vis[to[i]]&4))) {
		dfsm(to[i]);
		s+=sz[to[i]], mp=max(mp,sz[to[i]]);
	}
	mp = max(mp,S-s);
	if(mp<zd) {
		zd=mp, hp=p;
	}
	sz[p]=s;
}
void finds(int p, int s) {
	zd=0x7fffffff;
	S=s;
	dfsm(p);
}
//GO//////////////////////////////////////////////////////
struct node {
	int l, b;
	bool operator<(const node&r) const {
		return l<r.l;
	}
} que[MAXN]; int qn; int cnt[MAXN];
void go(int p, int b, int l) {
	vis[p]=2;
	que[qn++]=(node){l, b};
	for(int i=hd[p]; ~i; i=nxt[i]) if((!(vis[to[i]]&2)) && (!(vis[to[i]]&4))){
		go(to[i], b, l+le[i]);
	}
}
//////////////////////////////////////////////////////////
int ans;
void calc(int p, int s) {
	finds(p,s);
	p=hp;
	vis[p]=-1;
	qn=0;
	que[qn++]=(node){0,p};
	for(int i=hd[p]; ~i; i=nxt[i]) if(!(vis[to[i]]&4)) {
		go(to[i], to[i], le[i]);
	}
	memset(cnt,0,sizeof cnt);
	REP(i,0,qn) {
		cnt[que[i].b]++;
	}
	sort(que,que+qn);
	int l=0, r=qn-1;
	while(l<r) {
		cnt[que[l].b]--;
		while(l<r && que[l].l+que[r].l>k) {
			cnt[que[r].b]--;
			r--;
		}
		ans+=r-l-cnt[que[l].b];
		l++;
	}
	for(int i=hd[p]; ~i; i=nxt[i]) if(!(vis[to[i]]&4)) {
		calc(to[i], sz[to[i]]);
	}
}
int main() {
	while(~scanf("%d%d", &n, &k) && (n||k)) {
		init(); ans=0;
		REP(i,1,n) {
			int a,b,c; scanf("%d%d%d", &a, &b, &c);
			adde(a,b,c);
		}
		calc(1,n);
		printf("%d
", ans);
	}
}