03-树3 Tree Traversals Again （25 分）

An inorder binary tree traversal can be implemented in a non-recursive way with a stack. For example, suppose that when a 6-node binary tree (with the keys numbered from 1 to 6) is traversed, the stack operations are: push(1); push(2); push(3); pop(); pop(); push(4); pop(); pop(); push(5); push(6); pop(); pop(). Then a unique binary tree (shown in Figure 1) can be generated from this sequence of operations. Your task is to give the postorder traversal sequence of this tree.

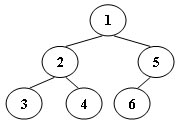
Figure 1

Input Specification:

Each input file contains one test case. For each case, the first line contains a positive integer N (≤) which is the total number of nodes in a tree (and hence the nodes are numbered from 1 to N). Then 2 lines follow, each describes a stack operation in the format: "Push X" where X is the index of the node being pushed onto the stack; or "Pop" meaning to pop one node from the stack.

Output Specification:

For each test case, print the postorder traversal sequence of the corresponding tree in one line. A solution is guaranteed to exist. All the numbers must be separated by exactly one space, and there must be no extra space at the end of the line.

Sample Input:

6
Push 1
Push 2
Push 3
Pop
Pop
Push 4
Pop
Pop
Push 5
Push 6
Pop
Pop

Sample Output:

3 4 2 6 5 1

 #include<cstdio>
 #include<stack>
 #include<cstring>
 using namespace std;
 const int maxn = 35;
 struct Node{
     int data;
    Node* lchild;
    Node* rchild;    
 };
 int n,pre[maxn],in[maxn],num = 0;
 
 Node* createTree(int preL,int preR,int inL,int inR){
     if(preL > preR) return NULL;
     Node* root = new Node;
     root -> data = pre[preL];
     //printf("%d
",root->data);
     int k;
     for(k = inL; k <= inR; k++){
         if(in[k] == pre[preL]) break;
     }
     int numLeft = k - inL;
     root->lchild = createTree(preL+1,preL+numLeft,inL,k-1);
     root->rchild = createTree(preL+numLeft+1,preR,k+1,inR);
     return root; 
 }
 
 void postOrder(Node* root){
     if(root == NULL) return;
    postOrder(root->lchild);
    postOrder(root->rchild);
    printf("%d",root->data);
    num++;
    if(num < n) printf(" ");
 }
 
 int main(){
     int x,k1=0,k2=0;
     scanf("%d",&n);
     stack<int> st;
     char str[5];
     for(int i = 0; i < 2*n; i++){
         scanf("%s",str);
         if(strcmp(str,"Push") == 0){
             scanf("%d",&x);
             st.push(x);
             pre[k1++] = x;
         }else{
             in[k2++] = st.top();
             st.pop();
         }
     }
    // printf("1
");
     Node* root = createTree(0,n-1,0,n-1);
    // printf("2
");
     postOrder(root);
     return 0;
 }