【CODEFORCES】 C. Dreamoon and Strings

C. Dreamoon and Strings

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

Dreamoon has a string s and a pattern string p. He first removes exactly x characters from s obtaining string s' as a result. Then he calculates 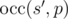 that is defined as the maximal number of non-overlapping substrings equal to p that can be found in s'. He wants to make this number as big as possible.

More formally, let's define 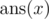 as maximum value of 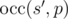 over all s' that can be obtained by removing exactly x characters from s. Dreamoon wants to know 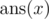 for all x from 0 to |s| where |s| denotes the length of string s.

Input

The first line of the input contains the string s (1 ≤ |s| ≤ 2 000).

The second line of the input contains the string p (1 ≤ |p| ≤ 500).

Both strings will only consist of lower case English letters.

Output

Print |s| + 1 space-separated integers in a single line representing the 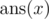 for all x from 0 to |s|.

Sample test(s)

input

aaaaa
aa

output

2 2 1 1 0 0

input

axbaxxb
ab

output

0 1 1 2 1 1 0 0

Note

For the first sample, the corresponding optimal values of s' after removal 0 through |s| = 5 characters from s are {"aaaaa", "aaaa","aaa", "aa", "a", ""}.

For the second sample, possible corresponding optimal values of s' are {"axbaxxb", "abaxxb", "axbab", "abab", "aba", "ab",

题解：这一题是DP。用D[i][j]表示从1至i。已经取走j个字符时的最大匹配串数。那么，第i位就有2种决策。取还是不取。而不取又分两种情况，一种是与前面的字符串没有能匹配的，一种是和前面的字符串有能匹配的。所以我们能够将状态转移方程表演示样例如以下：

D[i][j]=max(D[i-1][k-1],D[i-1][k])    //取还是不取

D[i][j]=max(D[i][j],D[k][j-(i-k-l2)])   //假设与前面有能匹配的情况

分析清楚了以后。能够发现这个问题跟最长不下降子序列事实上非常像。接下来就是编程实现了。要注意边界。D[i][j]的j不能大于i。并且不能为负数。

#include <iostream>
#include <cstring>
#include <cstdio>

using namespace std;

char s1[2005],s2[2005];
int l1,l2,j,d[2005][2005],f;

int mac(int i)
{
    int x=i,y=l2;
    while (x && y)
    {
        if (!y) break;
        if (s1[x-1]==s2[y-1]) {x--; y--;}
        else x--;
    }
    if (!y)
    {
        j=x;
        return 1;
    }
    else
    {
        j=0;
        return 0;
    }
}

int main()
{
    scanf("%s",s1);
    scanf("%s",s2);
    l1=strlen(s1);
    l2=strlen(s2);
    for (int i=1;i<=l1;i++)
    {
        f=mac(i);
        for (int k=0;k<=i;k++)
        {
            d[i][k]=max(d[i-1][k],d[i-1][k-1]);
            if (f && j>=k-i+j+l2 && k-i+j+l2>=0) d[i][k]=max(d[i][k],d[j][k-(i-j-l2)]+1);
        }
    }
    for (int i=0;i<=l1;i++)
        printf("%d ",d[l1][i]);
    return 0;
}